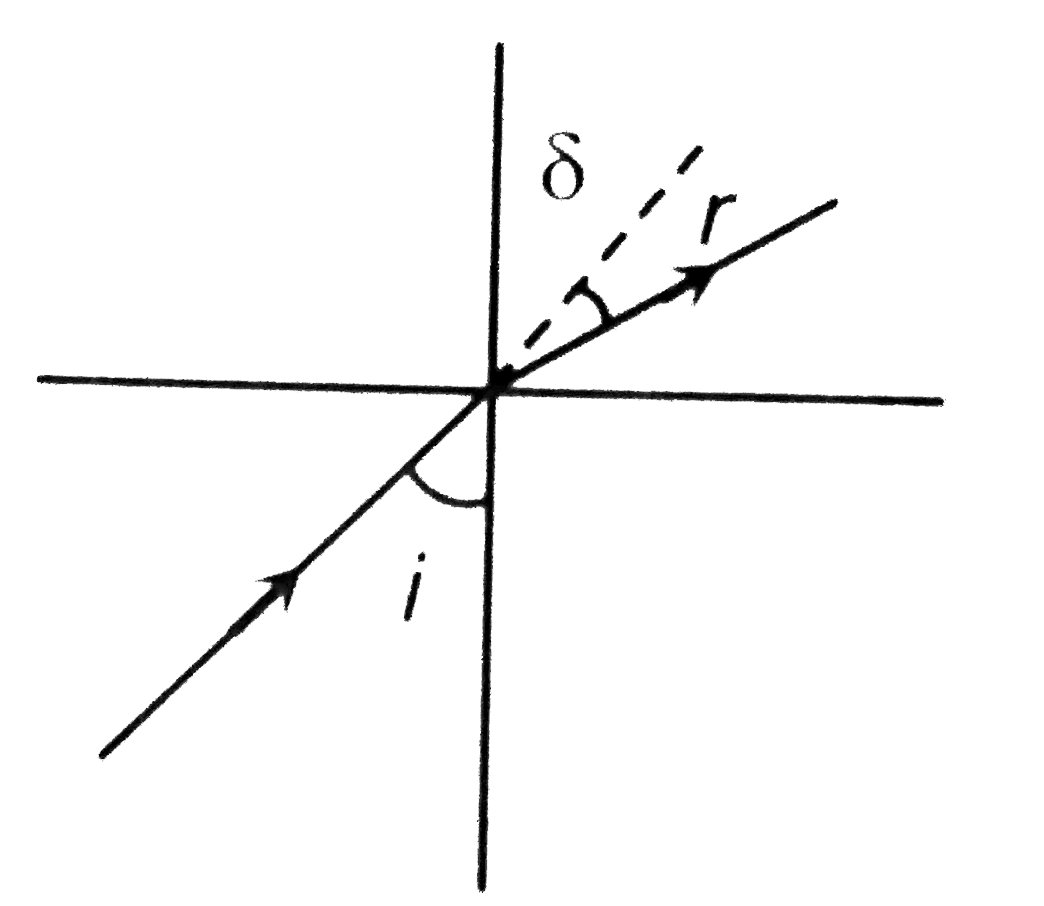
A ray of light travels from a medium of refractive index μ to air. Its angle of incidence in the medium is i, measured from the normal to the boundary , and its angle of deviation is δ.δ is plotted against i. Which of the following best represents the resulting curve ?
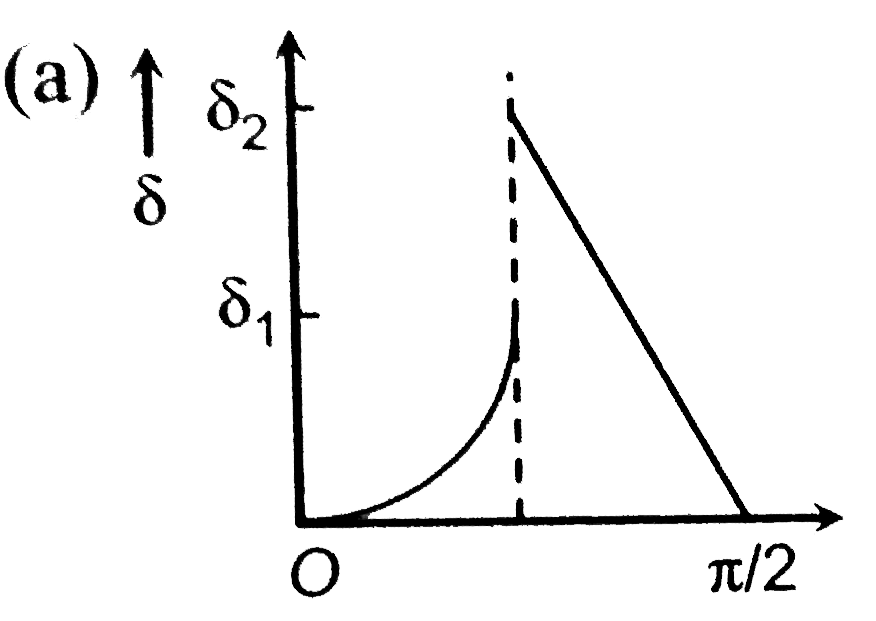
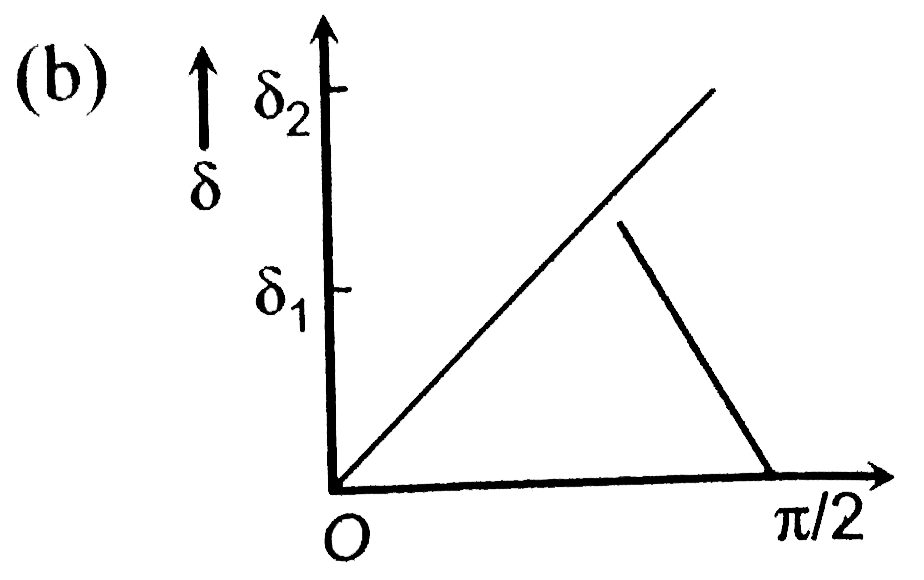
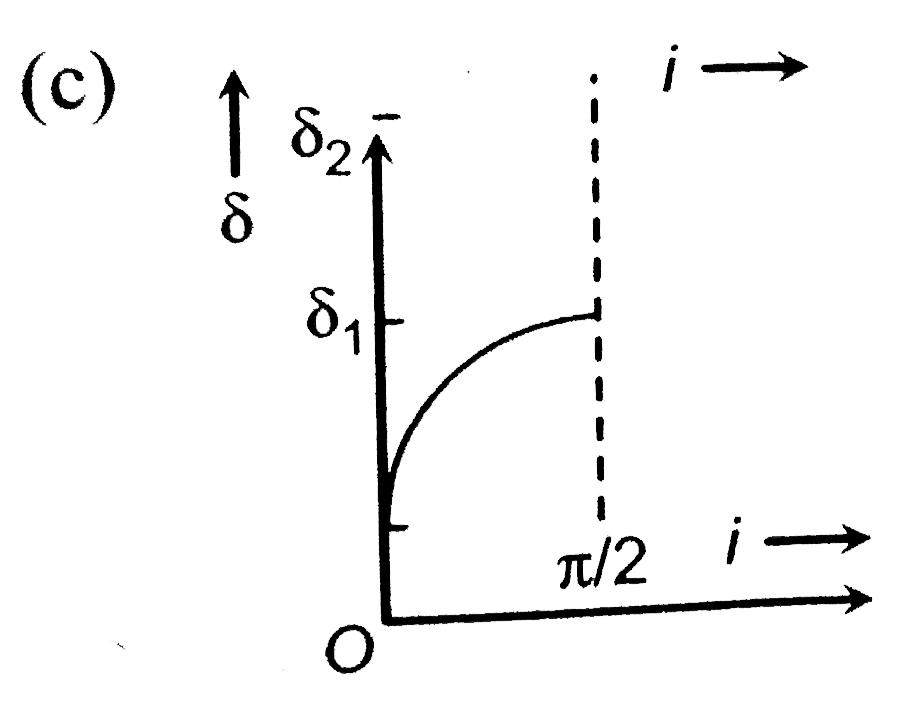
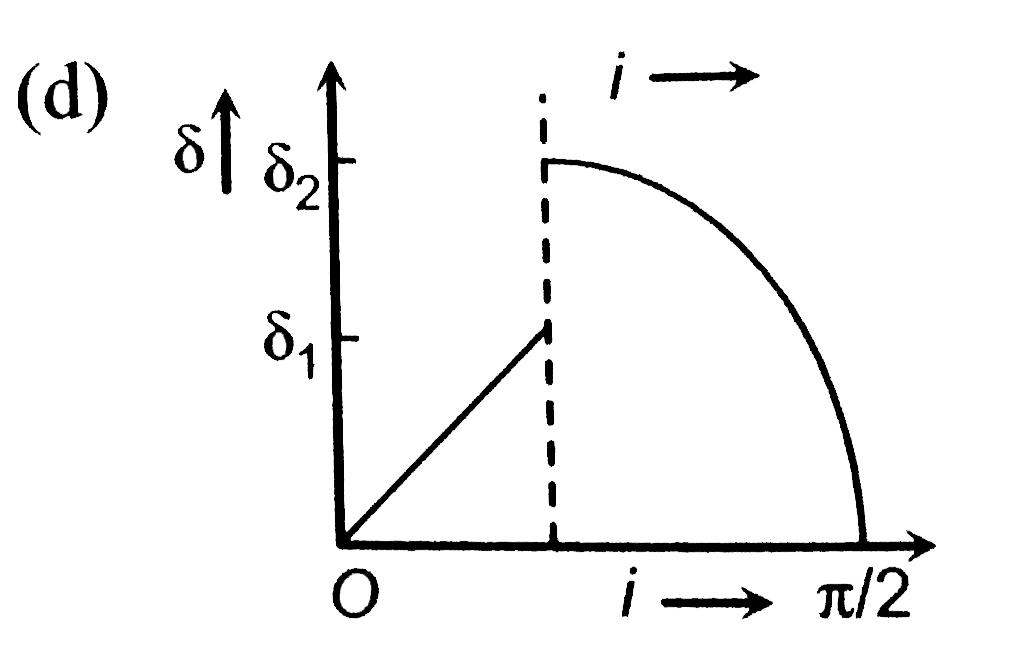
The correct Answer is:A
The ray of light is refracted at the plane surface.
However, since the ray is travelling from a denser to a rarer medium, for an angle of incidence (i) greater then the critical angle (c) the ray will be totally internally reflected.
For i<c , deviation δ=r−i with 1μ=sinisinr
Hence δ=sin−1(μsini)−i
This is a non− linear relation. The maximum value of δ is δ1=π2−c, wherei=c and μ=1sinc
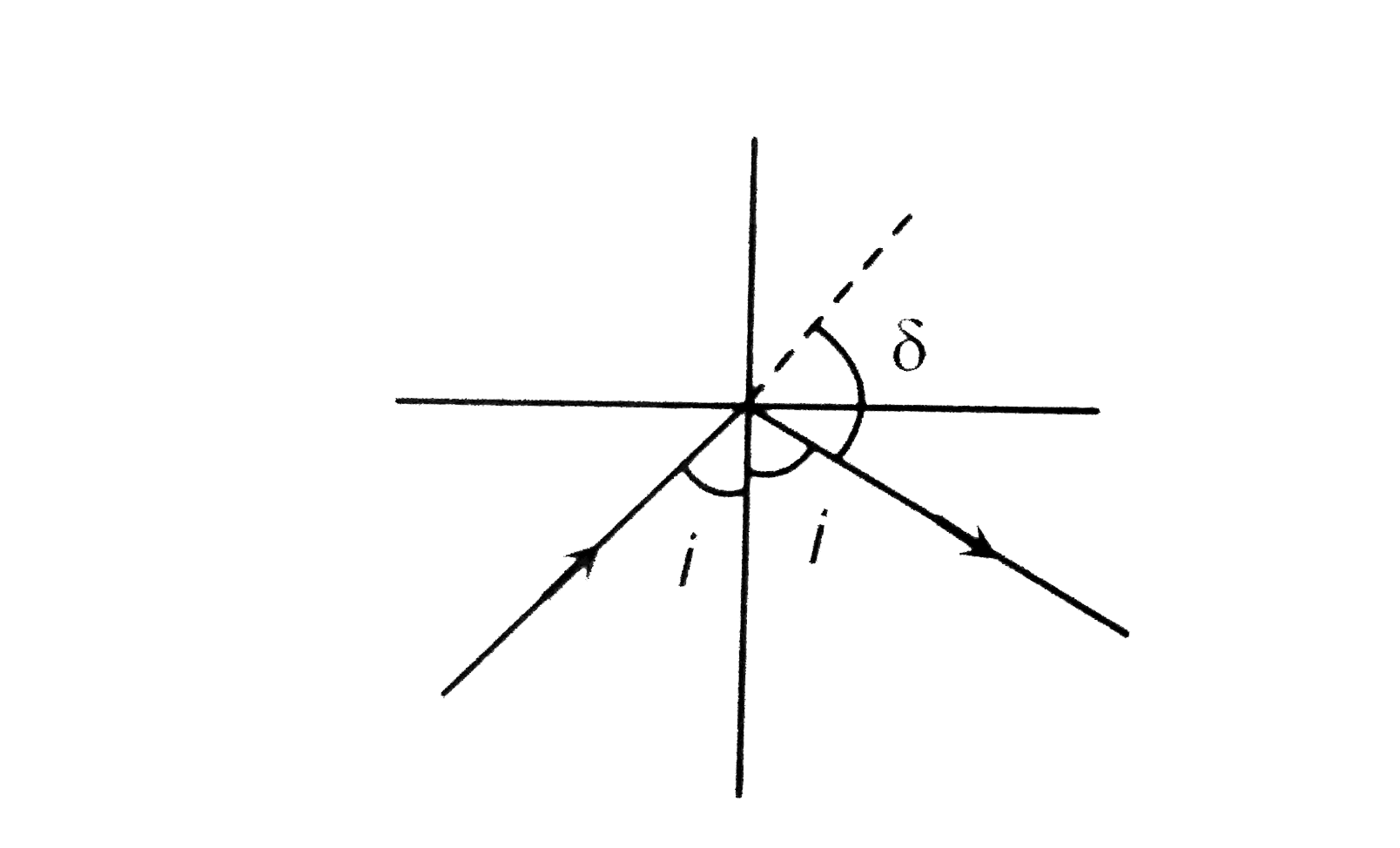
For i>c, deviationd=π−2i
δ decreases linearly with i .
δ2=π−2c=2δ1
Topper's Solved these Questions
- BOOK - A2ZCHAPTER - GEOMETRICAL OPTICSEXERCISE - Refraction At Curved Surface64videos
GEOMETRICAL OPTICS
- BOOK - A2ZCHAPTER - GEOMETRICAL OPTICSEXERCISE - Prism Theory And Dispersion Of Light45videos
GEOMETRICAL OPTICS
- BOOK - A2ZCHAPTER - ELECTROMAGNETIC WAVES AND COMMUNICATION SYSTEM EXERCISE - Section D - Chapter End Test30videos
ELECTROMAGNETIC WAVES AND COMMUNICATION SYSTEM
- BOOK - A2ZCHAPTER - MAGNETISM AND MATTEREXERCISE - Section D - Chapter End Test30videos
MAGNETISM AND MATTER
Similar Questions
A ray of light travels from a medium of refractive index μ to air. Its angle of incidence in the medium is i and its angle of deviation is δ. (a) What is the angle of deviation for i<C and i>C, where Ci s the critical angle. (b) Plot a curve between angle of deviation δ versus angle of incidence i.
View SolutionLight enters from vacuum to a medium of refractive index μ. If angle of incidence is double of angle of refraction, find angle of refraction.
View Solution
Knowledge Check
- Question 1 - Select One
A ray of light travels from a medium of refractive index n into air. If the angle of incidence at the plane surface of separation is θ. And the correspoonding angle of deviation is δ, the variation δ with θ is shown correctly in the figure.
A B
B C
C D
D
- Question 2 - Select One
The ray of light travels from raer medium to denser medium of refractive index μ. The angle of incidence of twice the angle of refraction. The angle of incidence is given by
A2sin−1(μ2)B2cos−1(2μ)C2cos−1(μ2)Dsin−1(μ2) - Question 3 - Select One
A ray of light passes from vaccum into a medium of refractive index n. If the angle of incidence is twice the angle of refraction, then the angle of incidence is
Acos−1(n/2)Bsin−1(n/2)C2cos−1(n/2)D2sin−1(n/2)
A ray of light passes from vaccume into a medium of refractive index n. if the angle of incidence is twice the angle of refraction, then the angle of incidence is
View SolutionA ray of light travelling in air is incident at 45∘ on a medium of refractive index √2. The angle of refractive in the medium is
View SolutionA ray of light passes from vacuum into a medium of refractive index n . If the angle of incidence is twice the angle of refraction , then the relation between the angle of incidence and the refractive index is
View SolutionA ray of light passes from vacuum of 3×108m/s medium of refractive index μ, the angle of incidence is found to be twice the angle of refraction. Then the angle of incidence is:
View SolutionA ray of light passes from vacuum of 3×108m/s medium of refractive index μ, the angle of incidence is found to be twice the angle of refraction. Then the angle of incidence is:
View Solution
