The eye can detect 5×104 photons (m2s)−1 of green light (λ=5000A), whole ear can detect 10−13Wm2 . As a power detector, which is more sensitive and by what factor?
5
10
106
15
The correct Answer is:A
E=123755000=2.475eV≈4×10−19J
So the minimum intensity to which the eye can respond
IEye=(Photon flux) xx ( Energy of a photon)
⇒IEye=(5×104)×(4×10−19)=2×10−14(W/m2)
Now as lesser the intensity required by a detector for detection , more sensitive it will be
SEyeSEar=IEarIEye=10−132×10−14=5 i.e., as intensity (power)
detector , the eye is five times more sensitive than ear.
Topper's Solved these Questions
- DUAL NATURE OF RADIATION AND MATTERBOOK - A2ZCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - Section B - Assertion Reasoning
18 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - A2ZCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - AIPMT/NEET Questions
46 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - A2ZCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - X-Rays
45 Videos
- CURRENT ELECTRICITYBOOK - A2ZCHAPTER - CURRENT ELECTRICITYEXERCISE - Section D - Chapter End Test
29 Videos
- ELECTRIC CHARGE, FIELD & FLUXBOOK - A2ZCHAPTER - ELECTRIC CHARGE, FIELD & FLUXEXERCISE - Section D - Chapter End Test
29 Videos
Similar Questions
Estimate the following the following two numbers should be interesting. The first number will tell you why radio engieers do not need to worry much about a photons. The second number tells you why our eye can never "count photon" even in barely detectable light.
(i) The number of photons emitted per second by a MW transmitter of 10kW power emitting radiowaves of length 500m.
(ii) The number of photons entering the pupil of our eye per second corresponding to the minimum intensity of white light that we humans can precieve (~10−10Wm−2). Take the area of the pupil to be about 0.4cm2, and the average frequency of white light to be about 6×104Hz.(h=6.6×10−34J)
View SolutionThe rate constant of a reaction is 5×10−8 mole litre−1sec−1 .How long it would take to change concentration for 4×10−2M to 2×10−2M?
View Solution
Knowledge Check
Eye of an experimenter detects the green light (λ=4000Å) at 5×104 photons per square meter per second and ear can detect 10−13 watt per square metre. The eye is sensitive as a power detector in comparison to the ear by a factor of
A2 timesB3 timesC4 timesD5 timesThe human eye can barely detect a yellow lings (λ=6000A) that delivers 1.7×10−18W to the retina. The number of photons per second falling on the eye is nearest to
A5×109B5000C50D5At t=O, light of intensity 1012 photons/s−m2 of energy 6eV per photon starts falling on a plate with work founction 2.5eV If area of the plate is 2×10−4m2 and for every 105 photons one photoelectron is emitted, charge on the plate at t=25 s is
A8×10−15CB4×10−14CC12×10−10CD16×10−12C
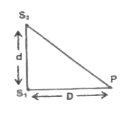
Two sources S1 and S2 emitting light of wave lengths 600 nm are placed a distance 1.0 x 102 cm apart..A detector can be moved on the line which is perpendicular to SiS2 . Find out the position of first.minimum detected.
View SolutionFind the number of photons entering the pupil of our eye per second corresponding to the minimum internsity of white light that we humans can perceive (−10−10Wm−2). Take the area of the pupil to be about 0.4cm2, and the average frequency of white light to be about 6×1014Hz.
View SolutionThe faintest sound the human ear can detect at a frequency of 1000 Hz correspond to an intensity of about 1.00×10−12Wm2, which is called threshold of hearing. The loudest sounds the ear can tolerate at this frequency correspond to an intensity of about 1.00Wm2, the threshold of pain. Detemine the pressure amplitude and displacement amplitude associated with these two limits. Take speed of sound=342ms and density of air=1.20kgm3
View SolutionThreshold frequency of a metal is 5×1013s−1 upon which 1×1014s−1 frequency light is focused. Then the maximum kinetic energy of emitted electron is
View SolutionThe pupil allows light to pass through it and has a diameter of 2×10−3m. If minimum light intensity that should enter the eye is 1.8×10−10W/m−2, then find the number of photon in visible range must enter the pupil to have vision. Wavelength of photon in visible range can be taken as 5,500Å .
View Solution