For an elementry reaction aA→ product, the graph plotted between reaction log[−d[A]dt] vs. time gives a straight line with intercept equal to 0.6 and showing an angle of 45∘ with origin, then:
rate constant=3.98mol litre−1t−1anda=1
rate constant=3.98time−1anda=1
rate constant=1.99mol litre−1t−1anda=2
rate constant=1.99time−1anda=1
The correct Answer is:b
Rate =−dCAdt=KCaA
∴Log[−dCAdt]=logK+alogCA
∴logK=0.6
K=3.98time and a=1=tan45∘
Topper's Solved these Questions
- BOOK - P BAHADURCHAPTER - CHEMICAL KINETICSEXERCISE - Exercise 12videos
CHEMICAL KINETICS
- BOOK - P BAHADURCHAPTER - CHEMICAL KINETICSEXERCISE - Exercise 3A2videos
CHEMICAL KINETICS
- BOOK - P BAHADURCHAPTER - DILUTE SOLUTION AND COLLIGATIVE PROPERTIESEXERCISE - Exercise 9 Advanced Numerical12videos
DILUTE SOLUTION AND COLLIGATIVE PROPERTIES
Similar Questions
For a reaction A→ Product, a graph plotted between 1[A]2 vs time is found to be linear with slope = 0.4 and y intercept equal to 4 as shown. The rate of disapparance of A at the initial stages will be:
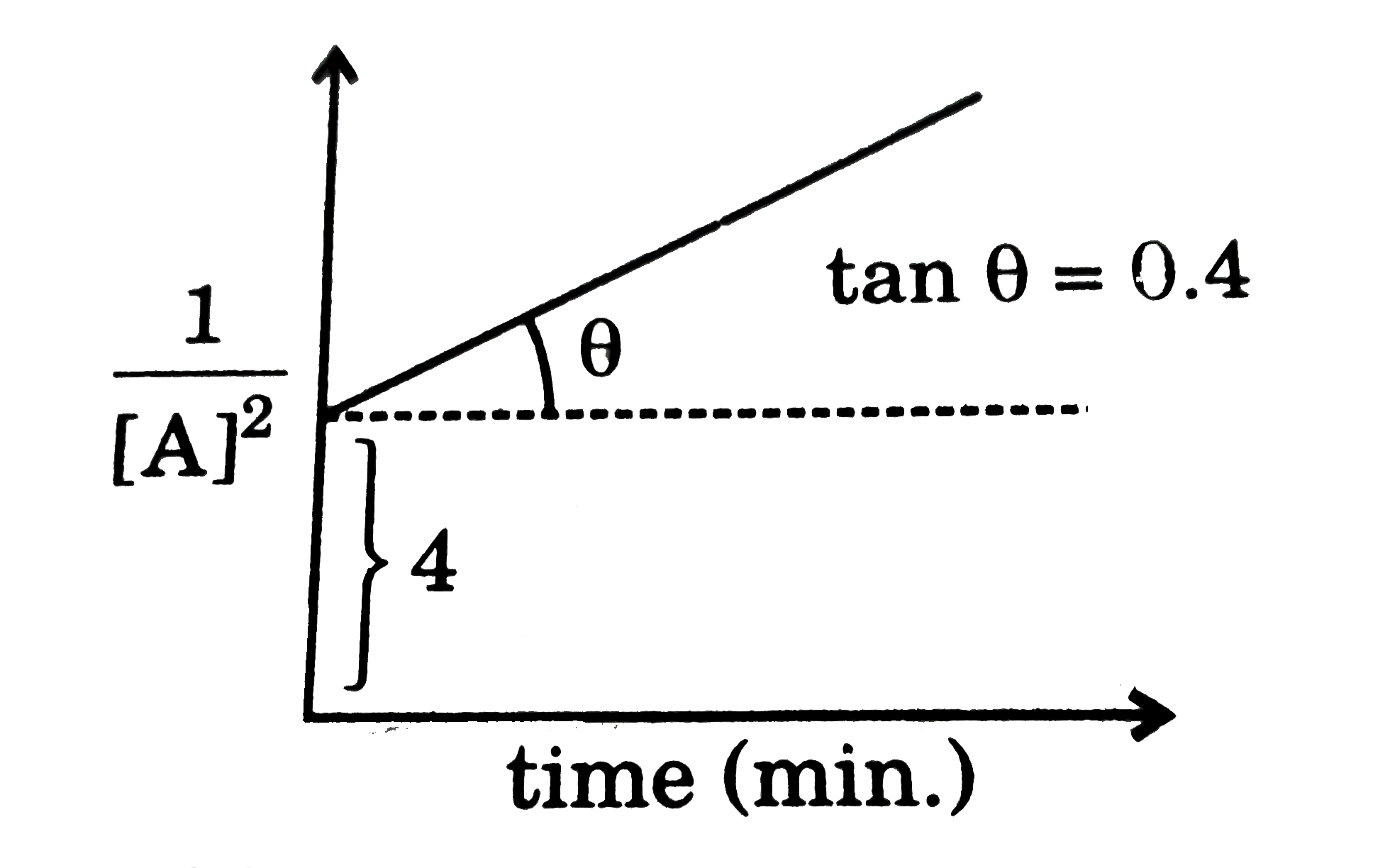
[A] is in terms of molarityView SolutionFor a first order reaction the plot of ‘t’ against log c gives a straight line with a slope equal to :
View Solution
Knowledge Check
- Question 1 - Select One
The instantaneous rate of an elementary chemical reaction
aA+bB(⇔)cC+dD can be given by:
rate =kf[A]a[B]b−kb[C]c[D]d
where kfandkb are rate constants for forward and backward reactions respectively for the reversible reaction if the reaction is an irreversible one , the rate is expressed as rate =k[A]a[B]b where k is rate constant for the given irreversible reaction and (a+b) is the order of reaction it is also evident from the stoichiometry of reaction that rates of disappearance of A is ab times the rate of disappearance of B. The variation of rate constant k with temperature is expressed in terms of Arrhenius equation: k=Ae−(Ea/RT) whereas the ratio kfkb is expressed in terms of van't Hoff isochore: KfKb=Ae−ΔH/RT where Ea and ΔH are energy of activation and enthalpy of reaction respectively
For an elementary reaction aA→ product the graph plotted between log [−d[A]dt] vs. concentration gives a straight line with intercept equal to 0.6 and showing an angle of 45∘ with origin, then:Arate constant =3.98time−1anda=1Brate constant =3.98molL−1t−1anda=1Crate constant =1.99time−1anda=1Drate constant =1.99molL−1t−1anda=2 - Question 2 - Select One
The plots of log10Kvs.1/T leads toa straight line having intercept equal to:
AΔG∘BΔG∘2.303RCΔS∘2.303RDΔH∘2.303R - Question 3 - Select One
A graph plotted between log N vs, time gives a slope and intercept equal to:
A−λ2.303,logN0Bλ2.303,logN0C−λ2.303,N0Dλ2.303,N0
For a first order reaction the plot of ‘t’ against log c gives a straight line with a slope equal to :
View SolutionFor a first order reaction the plot of ‘t’ against log c gives a straight line with a slope equal to :
View SolutionFor the order reaction , plot of log10(a−x) against time t is a straight line with a negative slope equal to ________.
View SolutionFor a first order reaction, the reaction, the plot of logC against 't' (log C vs 't') gives a straight line with slope equal to:
View SolutionFor the first order reaction , plot of log10(a−x) against tine 't' is a straight line with slope equal to __________.
View Solution

