Two metal plates are held together by two rivets width radii of 0.2cm. If the maximum shear stress a single rivet can withstand is 5×108Nm−2, how much force must be applied parallel to the plates to shear off both the rivets?
Here, radius of each rivet,
r=0.2cn=2×10−3m
Maximum shear stress, Smax=5×108Nm−2,
Required force F = shear stress × area of cross-section of two rivets
=(5×108)×2×π×(2×10−3)2
1.26×104N.
Topper's Solved these Questions
- PROPERTIES OF BULK MATTERBOOK - PRADEEPCHAPTER - PROPERTIES OF BULK MATTEREXERCISE - Conceptual Problems
103 Videos
- PROPERTIES OF BULK MATTERBOOK - PRADEEPCHAPTER - PROPERTIES OF BULK MATTEREXERCISE - Advanced problems for competitions
21 Videos
- PROPERTIES OF BULK MATTERBOOK - PRADEEPCHAPTER - PROPERTIES OF BULK MATTEREXERCISE - Long Answer questions
33 Videos
- PHYSICAL WORLD AND MEASUREMENTBOOK - PRADEEPCHAPTER - PHYSICAL WORLD AND MEASUREMENTEXERCISE - Competiton Focus Jee Medical Entrance
18 Videos
- RAY OPTICSBOOK - PRADEEPCHAPTER - RAY OPTICSEXERCISE - Problem For Practice(a)
25 Videos
Similar Questions
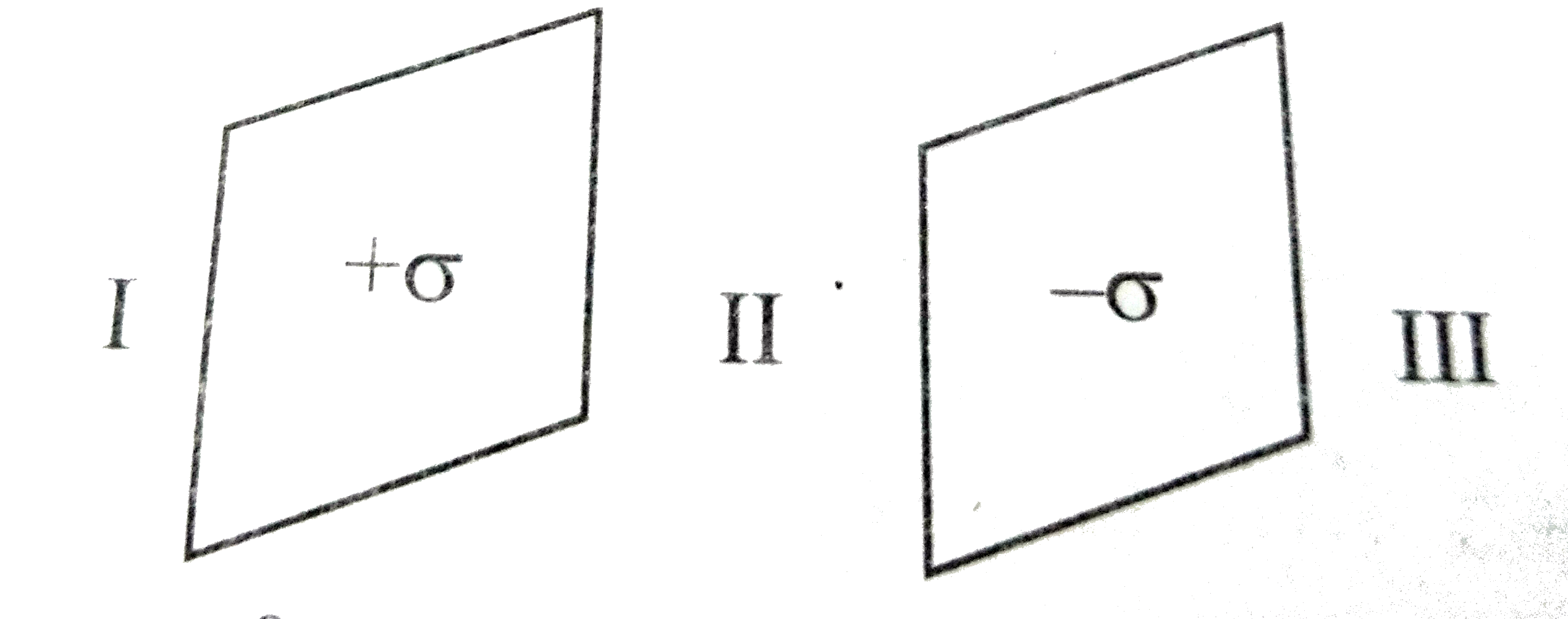
Two large, thin metal plates are parallel and close to each other. On their inner faces, the plates have surface charge densities of opposite signs and of magnitude 19×10−22Cm−2.
What is E (a) to the left of the plates (b) to the right of the plates (c) between the plates ?
Here, σ=19×10−22Cm−2
View SolutionCalculate the force F needed to punch at 1.46 cm diameter hole in a steel plate 1.27cm thick fig. The ultimates shear stress of steel is 3.45×108Nm−2.

View Solution
Knowledge Check
Two long metallic strips are joined together by two rivets each of radius 2mm. Each rivet can withstand a maximum shearing stress of 1.5×109N/m2. Assuming that each rivet shares the stretching load equally, the maximum tensile force the strip can exert without rupture is
A1.88×104NB3.8×104NC6×107ND3×104NTwo glass plates are separated by water. If surface tension of water is 75dyn/cm and the area of each plate wetted by water is 8cm2 and the distance between the plates is 0.12mm, then the force applied to separate the two plates is
A102dynB104dynC105dynD106dynTwo strips of metal are riveted together at their ends by four rivets, each of diameter 6 mm. Assume that each rivet is to carry one quarter of the load. If the shearing stress on the rivet is not to exceed 6.9×107Pa, the maximum tension that can be exerted by the riveted strip is
A2×103NB3.9×103NC7.8×103ND15.6×103N
A well collimated parallel pencil of cathode rays falls through a potential difference 3kV & enters the spacing between two parallel metallic plates, parallel to their length the spacing between the plates being 0.5cm. The pencil strikes a fluorescent screen, mounted perpendicular to the length of the plates at the farther end of the plates & produces fluorescent spot. if now a potential difference of 3V is applied across the two plates, calculate the linear deflection of the spot on the screen. Given the length of the plates is 10 cm.
View SolutionTwo strips of metal are riveted together at their ends by four rivets, each of diameter 6.0 mm. What is the maximum tension that can be exerted by the riveted strip if the shearing stress on the rivet is not to exceed 2.3×109Pa ? Assume that each rivet is to carry one quarter of the load.
View SolutionTwo large metal plates of area 6.0m2 face each other . The plates are 3 cm apart and carry equal and opposite charges on their inner surfaces. If electric field at a point between the plates is 5×104NC−1, then calculate the magnitude of charge on each plates.
View SolutionTwo large thin metal plates are parallel and close to each other. On their inner faces, the plates have surface charge densities of opposite signs and magnitude 27×10−22Cm−2. The electric field →E in region II in between the plates is
View SolutionTwo strips of metal are riveted together at their ends by four rivets, each of diameter 6mm. What is the maximum tension that can be exterted by the riveted strip if the shearing stress on the rivet is not to exceed 6.9×107Pa? Assume that each rivet is to carry one quarter of the load .
View Solution