Does it matter if one uses gauge instead of absolute pressures in applying Bernoulli's equation. Explain.

Video Solution

Text Solution

Generated By DoubtnutGPT

To determine whether it matters if one uses gauge pressure instead of absolute pressure when applying Bernoulli's equation, we can follow these steps:
Step 1: Understand the Definitions
- Gauge Pressure (Pg): This is the pressure measured relative to atmospheric pressure. It does not include the atmospheric pressure in its value.
- Absolute Pressure (Pa): This is the total pressure measured relative to a perfect vacuum. It includes atmospheric pressure.
Step 2: Relationship Between Gauge and Absolute Pressure
- The relationship between gauge pressure and absolute pressure can be expressed as:
$P a = P g + P a t m$
where $P a t m$ is the atmospheric pressure.
Step 3: Write Bernoulli's Equation
- Bernoulli's equation states:
$P + \frac{1}{2} ρ V^{2} + ρ g h = constant$
where $P$ is the pressure at a point, $ρ$ is the fluid density, $V$ is the fluid velocity, and $h$ is the height above a reference level.
Step 4: Apply Bernoulli's Equation Between Two Points
- When applying Bernoulli's equation between two points (1 and 2), we can write:
$P_{1} + \frac{1}{2} ρ V_{1}^{2} + ρ g h_{1} = P_{2} + \frac{1}{2} ρ V_{2}^{2} + ρ g h_{2}$
Step 5: Substitute Absolute Pressure with Gauge Pressure
- If we substitute absolute pressures $P_{1}$ and $P_{2}$ with gauge pressures, we have:
$(P g_{1} + P a t m) + \frac{1}{2} ρ V_{1}^{2} + ρ g h_{1} = (P g_{2} + P a t m) + \frac{1}{2} ρ V_{2}^{2} + ρ g h_{2}$
Step 6: Simplify the Equation
- Rearranging gives:
$P g_{1} + P a t m + \frac{1}{2} ρ V_{1}^{2} + ρ g h_{1} = P g_{2} + P a t m + \frac{1}{2} ρ V_{2}^{2} + ρ g h_{2}$
- Notice that $P a t m$ appears on both sides of the equation and can be canceled out:
$P g_{1} + \frac{1}{2} ρ V_{1}^{2} + ρ g h_{1} = P g_{2} + \frac{1}{2} ρ V_{2}^{2} + ρ g h_{2}$
Step 7: Conclusion
- Since the atmospheric pressure cancels out, it shows that using gauge pressure instead of absolute pressure does not affect the application of Bernoulli's equation. Therefore, it does not matter whether gauge or absolute pressure is used in this context.

Updated on:7/8/2024

Bernoulli's principle does not explain :
Acurved path of a spinning ball.
Blift of a jet.
Cworking of a point sprayer.
Dautomatic blowing of 7 the roots of houses during lizard in hilly areas.
Statement-1 : Bernoullis equation is based on energy conservation.
Statement-2 : Bernoullis equation can only be applied if the flow is streamlined.
Statement-3 : Bernoullies equation can be applied evern if the flow is not streamlined as total energy is always conserved.
AT T T
BT F T
CT T F
DF F F
using the illustration when both surfaces 1 and 2 are exposed to the atmoshere, $P_{1}$ and $P_{2}$ are gauge pressure , and $h_{1}$ and $h_{2}$ are heights .

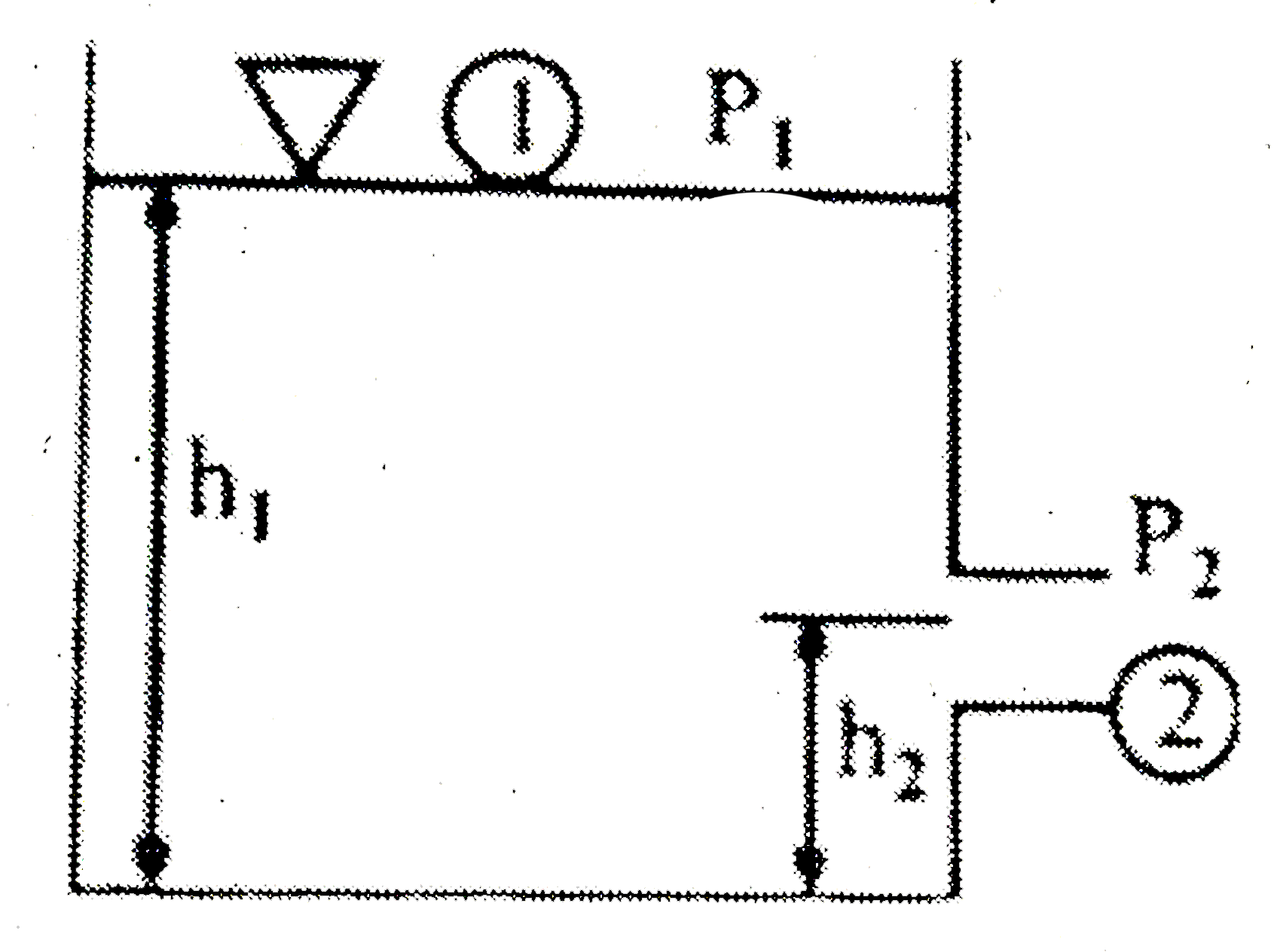
The Bernoulli equation for this situation is $h_{1} + \frac{P_{1}}{W} + \frac{V_{1}^{2}}{2a} = h_{2} + \frac{P_{2}}{W} + \frac{V_{2}^{2}}{2a}$
The velocity of the fluid leavening point (2) is constant and can be expressed as
A $V_{2} = (P_{1} / P_{2}) V_{1}$
B $V_{2} = (P_{1} / P_{2}) a h$
C $V_{2} = {[(h_{1} - h_{2}) (2 a)]}^{1 / 2}$
Dnone of the above