Estimate the rate at which ice would melt in a wooden box 20 mm thick and of inside measurement 200 cm x 100 cm x 100 cm assumming that external temperature is and coefficient of thermal conductivity of wood is .
The correct Answer is:
To estimate the rate at which ice would melt in a wooden box, we can follow these steps:
Step 1: Understand the problem
We need to calculate the rate of heat transfer through the wooden box to determine how much ice will melt. The parameters provided include the dimensions of the box, the thickness of the wood, the external temperature, and the thermal conductivity of wood.
Step 2: Convert units
- The thickness of the wooden box is given as 20 mm. We convert this to centimeters:
Thickness=20mm=2cm
Step 3: Calculate the surface area of the box
The box has the following dimensions:
- Inside dimensions: 200 cm x 100 cm x 100 cm
- The surface area consists of:
- 2 faces of 200 cm x 100 cm
- 2 faces of 100 cm x 100 cm
- Calculating the area:
Area=2×(200×100)+2×(100×100)=2×20000+2×10000=40000+20000=60000cm2
Step 4: Use the formula for heat transfer
The rate of heat transfer dqdt through the box can be calculated using Fourier's law of heat conduction:
dqdt=K⋅A⋅ΔTd
Where:
- K = thermal conductivity of wood = 0.0004 \, \text{cal s}^{-1} \text{cm}^{-1} \degree C^{-1}
- A = surface area = 60000cm2
- ΔT = temperature difference = 27 \degree C - 0 \degree C = 27 \degree C
- d = thickness of the wood = 2cm
Step 5: Substitute the values into the equation
Substituting the values into the equation:
dqdt=0.0004⋅60000⋅272
Step 6: Calculate the heat transfer rate
Calculating the above expression:
dqdt=0.0004⋅60000⋅272=6482=324cal/s
Step 7: Calculate the mass of ice melted per second
To find the mass of ice melted per second, we use the latent heat of fusion for ice, which is L=80cal/g:
Mass melted per second=dqdtL=324cal/s80cal/g=4.05g/s
Conclusion
The rate at which ice would melt in the wooden box is approximately 4.05g/s.
---
We need to calculate the rate of heat transfer through the wooden box to determine how much ice will melt. The parameters provided include the dimensions of the box, the thickness of the wood, the external temperature, and the thermal conductivity of wood.
- The thickness of the wooden box is given as 20 mm. We convert this to centimeters:
Thickness=20mm=2cm
The box has the following dimensions:
- Inside dimensions: 200 cm x 100 cm x 100 cm
- The surface area consists of:
- 2 faces of 200 cm x 100 cm
- 2 faces of 100 cm x 100 cm
- Calculating the area:
Area=2×(200×100)+2×(100×100)=2×20000+2×10000=40000+20000=60000cm2
The rate of heat transfer dqdt through the box can be calculated using Fourier's law of heat conduction:
dqdt=K⋅A⋅ΔTd
Where:
- K = thermal conductivity of wood = 0.0004 \, \text{cal s}^{-1} \text{cm}^{-1} \degree C^{-1}
- A = surface area = 60000cm2
- ΔT = temperature difference = 27 \degree C - 0 \degree C = 27 \degree C
- d = thickness of the wood = 2cm
Substituting the values into the equation:
dqdt=0.0004⋅60000⋅272
Calculating the above expression:
dqdt=0.0004⋅60000⋅272=6482=324cal/s
To find the mass of ice melted per second, we use the latent heat of fusion for ice, which is L=80cal/g:
Mass melted per second=dqdtL=324cal/s80cal/g=4.05g/s
The rate at which ice would melt in the wooden box is approximately 4.05g/s.
Topper's Solved these Questions
- PROPERTIES OF BULK MATTERBOOK - PRADEEPCHAPTER - PROPERTIES OF BULK MATTEREXERCISE - Multiple choice questions-II
14 Videos
- PROPERTIES OF BULK MATTERBOOK - PRADEEPCHAPTER - PROPERTIES OF BULK MATTEREXERCISE - Multiple choice questions-I
173 Videos
- PROPERTIES OF BULK MATTERBOOK - PRADEEPCHAPTER - PROPERTIES OF BULK MATTEREXERCISE - Fill in the Blanks E
10 Videos
- PHYSICAL WORLD AND MEASUREMENTBOOK - PRADEEPCHAPTER - PHYSICAL WORLD AND MEASUREMENTEXERCISE - Competiton Focus Jee Medical Entrance
18 Videos
- RAY OPTICSBOOK - PRADEEPCHAPTER - RAY OPTICSEXERCISE - Problem For Practice(a)
25 Videos
Similar Questions
Estimate the rate at which ice would melt in a wooden box 2.5 mm thick and of inside measurement 100 cm x 60 cm x 40 cm, assumming that external temperature is 32.∘C and coefficient of thermal conductivity of wood is 0.168Wm−1K−1. Given L=80cal/g.
View SolutionA cubical ice box of side 50 cm has a thickness of 5.0 cm. if 5 kg of ice is put in the box, estimate the amount of ice remaining after 4 hours. The outside temperature is 40∘C and coefficient of thermal conductivity of the material of the box = 0.01Js−1m−1.∘C−1. Heat of fusion of ice=335Jg−1.
View SolutionCalculate the rate of loss of heat through a glass window of area 1000cm2 and thickness 0.4 cm when temperature inside is 37∘C and outside is −5∘)C . Coefficient of thermal conductivity of glass is 2.2×10−3cals−1cm−1K−1.
View SolutionA cubical thermocol ice box of side length 30cm has thichess of 5.0cm If 4.0kg of ice is put in the box estimate the amount of ice remaining after 6hr The utside temperature is 45∘C and co-efficinet of the thermal conductivity of the thermocol is 0.01Js−1m−1K1 (Latent Heat of fusion of water =335×103Jkg−1) .
View SolutionSteam at 100∘C is passed into a copper cylinder 10 mm thick and of 200cm2 area. Water at 100∘C collects at the rate of 150g−1min. Find the temperature of the outer surface, if the conductivity of copper is 0.8cals−1cm−1.∘C−1 and the latent heat of steam is 540calg−1.
View SolutionA wooden box having dimensions 80 cm x 60 cm x 30 cm and thickness 3 cm contains ice. What will be the rate of melting of ice if external temperature is 30∘C and coefficient of thermal conductivity is 0.176Wm−1K−1 and latent heat of ice = 80 cal/g.
View SolutionFor a glass window of area 1500cm2 and thickness 0.5 cm, determine the rate of loss of heat if the temperature inside the window is 35∘C and outside is −2∘C . Take, coefficient of thermal conductivity of glass =2.2×10−3cals−1cm−1K−1
View SolutionA thermocole cubical icebox of side 30 cm has a thickness of 5.0 cm if 4.0 kg of ice are put ini the box, estimate the amount of ice remaining after 6 h. The outside temperature is 45∘C and coefficient of thermal conductivity of thermocole=0.01J/kg.
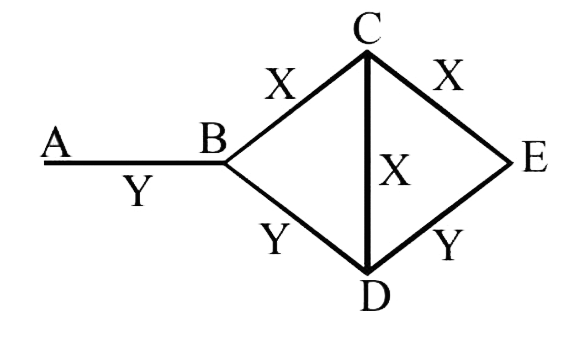
View SolutionThree rods of material X and three rods of material Y are connected as shown in the figure. All the rods are of identical length and cross-sectional area. If the end A is maintained at 60∘C and the junction E at 10∘C. Calculate the temperature of the junction B, C and D. The thermal conductivity of X is 0.92cal/sec−cm∘C and that of Y is 0.46cal/sec−cm−∘C.
View SolutionOn the Dal lake of Kashmir, a layer of 2 cm thick ice is formed when the outside temperature is −10∘C . How much time it will take for the increase in thickness of ice by 0.5 mm? Take, Density of ice =1g/c3 m
Latent heat of ice = 80 cal/g
Thermal conductivity of ice = 0.008cals−1cm−1∘C−1
View Solution
