A second pendulum is takin in a carriage. Find the period of oscillation when the carriage moves with an acceleration of 4⋅2ms−2 (i) vertically upwards (ii) vertically downwards and (ii) in a horizontal direction.
To find the period of oscillation of a second pendulum in a carriage moving with an acceleration of 4.2m/s2 in different directions, we will use the formula for the period of a pendulum:
T=2π√Lgeffective
where L is the length of the pendulum and geffective is the effective acceleration due to gravity.
Step 1: Determine the effective gravity when the carriage moves vertically upwards.
When the carriage moves vertically upwards, the effective gravity is given by:
geffective=g+a
where g=9.8m/s2 and a=4.2m/s2.
Calculating geffective:
geffective=9.8+4.2=14.0m/s2
Now, substituting geffective into the formula for the period:
T1=2π√Lgeffective=2π√L14.0
Step 2: Find the length L of the second pendulum.
For a second pendulum, the period T is 2 seconds. We can use this to find L:
T=2⇒2=2π√L9.8
Squaring both sides:
1=4L9.8π2
Solving for L:
L=9.8π24≈7.69m
Step 3: Calculate T1.
Now substituting L back into the equation for T1:
T1=2π√7.6914.0≈2π√0.549≈2π×0.740≈4.65seconds
Step 4: Determine the effective gravity when the carriage moves vertically downwards.
When the carriage moves vertically downwards, the effective gravity is given by:
geffective=g−a
Calculating geffective:
geffective=9.8−4.2=5.6m/s2
Now substituting into the formula for the period:
T2=2π√Lgeffective=2π√7.695.6≈2π√1.37≈2π×1.17≈7.35seconds
Step 5: Determine the effective gravity when the carriage moves horizontally.
When the carriage moves horizontally, the effective gravity is given by:
geffective=√g2+a2
Calculating geffective:
geffective=√(9.8)2+(4.2)2=√96.04+17.64=√113.68≈10.67m/s2
Now substituting into the formula for the period:
T3=2π√Lgeffective=2π√7.6910.67≈2π√0.72≈2π×0.85≈5.34seconds
Final Results:
- T1≈4.65seconds (upwards)
- T2≈7.35seconds (downwards)
- T3≈5.34seconds (horizontal)
- T1≈4.65seconds (upwards)
- T2≈7.35seconds (downwards)
- T3≈5.34seconds (horizontal)
Topper's Solved these Questions
- BOOK - PRADEEPCHAPTER - OSCILLATIONS AND WAVESEXERCISE - conceptual problems84videos
OSCILLATIONS AND WAVES
- BOOK - PRADEEPCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Very Short Answer Questions99videos
OSCILLATIONS AND WAVES
- BOOK - PRADEEPCHAPTER - OSCILLATIONS AND WAVESEXERCISE - CURIOSITY QUESTION2videos
OSCILLATIONS AND WAVES
- BOOK - PRADEEPCHAPTER - MATHEMATICAL TOOLSEXERCISE - Fill in the blanks5videos
MATHEMATICAL TOOLS
- BOOK - PRADEEPCHAPTER - PHYSICAL WORLD AND MEASUREMENTEXERCISE - Competiton Focus Jee Medical Entrance18videos
PHYSICAL WORLD AND MEASUREMENT
Similar Questions
A second's pendulum is taken in a carriage. Find the period of oscillation when the carriage move s with an acceleration of 4ms−2 (i) vertically upwards (ii) vertically downwards, and (iii) in a horizontal direction.
View SolutionFind the time period of a simple pendulum in a carriage accelerating
downwardsView Solution
Knowledge Check
- Question 1 - Select One
A simple pendulum is oscillating with an angular amplitude of 90∘ as shown in the figure. The value of θ for which the resulting acceleration of the bob is directed (i) vertically downward, (ii) vertically upward and (iii) horizontally is
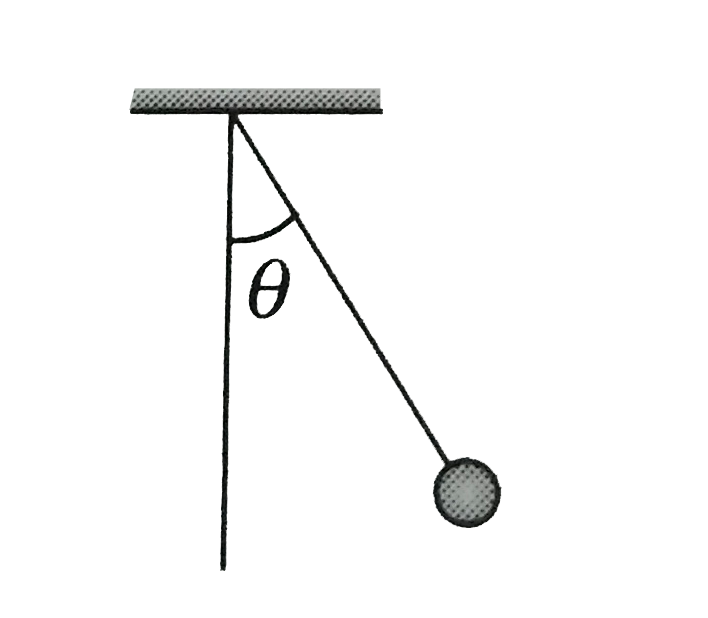 A90∘,0∘,sin−1(1/√3)B0∘,90∘,sin−1(1√3)C90∘,0∘,cos−1(1/√3)D0∘,90∘,cos−1(1/√3)
A90∘,0∘,sin−1(1/√3)B0∘,90∘,sin−1(1√3)C90∘,0∘,cos−1(1/√3)D0∘,90∘,cos−1(1/√3) - Question 2 - Select One
A seconds pendulum is suspended from the ceiling of a trolley moving horizontally with an acceleration of 4m/s2 . Its period of oscillation is
A1.90sB1.70sC2.30sD1.40s - Question 3 - Select One
A simple pendulum is vibrating with an angular amplitude of 90∘ as shown in the figure. For what value of a is the acceleration directed
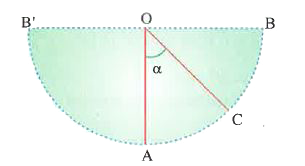
i) vertically upwards
ii) horizontally
iii) vertically downwardA0∘,cos−1(1√3),90∘B90∘,cos−1(1√3),0∘Ccos−1(1√3),0∘,90∘Dcos−1(1√3),90∘,0∘
A second's pendulum is taken to a lift. Calculate the time period of oscillation of pendulum if the (a) lift it moving upwards with a = 4 m/s2 and (b) downwards with a = 3 m/s2
View SolutionFind the time period of a simple pendulum in a carriage accelerating
horizontal with an acceleration a.View SolutionA person of 60 kg enters a lift going up with an acceleration 2ms−2 The vertical upward force acting on the person will be (g=10ms−2)
View SolutionA simple pendulum is suspended from the ceiling of a car and its period of oscillation is T when the car is at rest. The car starts moving on a horizontal road with a constant acceleration g (equal to the acceleration due to gravity, in magnitude) in the forward direction. To keep the time period same, the length of th pendulum
View SolutionA second pendulum is attached to roof of a car that is moving with acceleration 10m/s2 on a straight road. Its frequenct of oscillation is
View Solution

