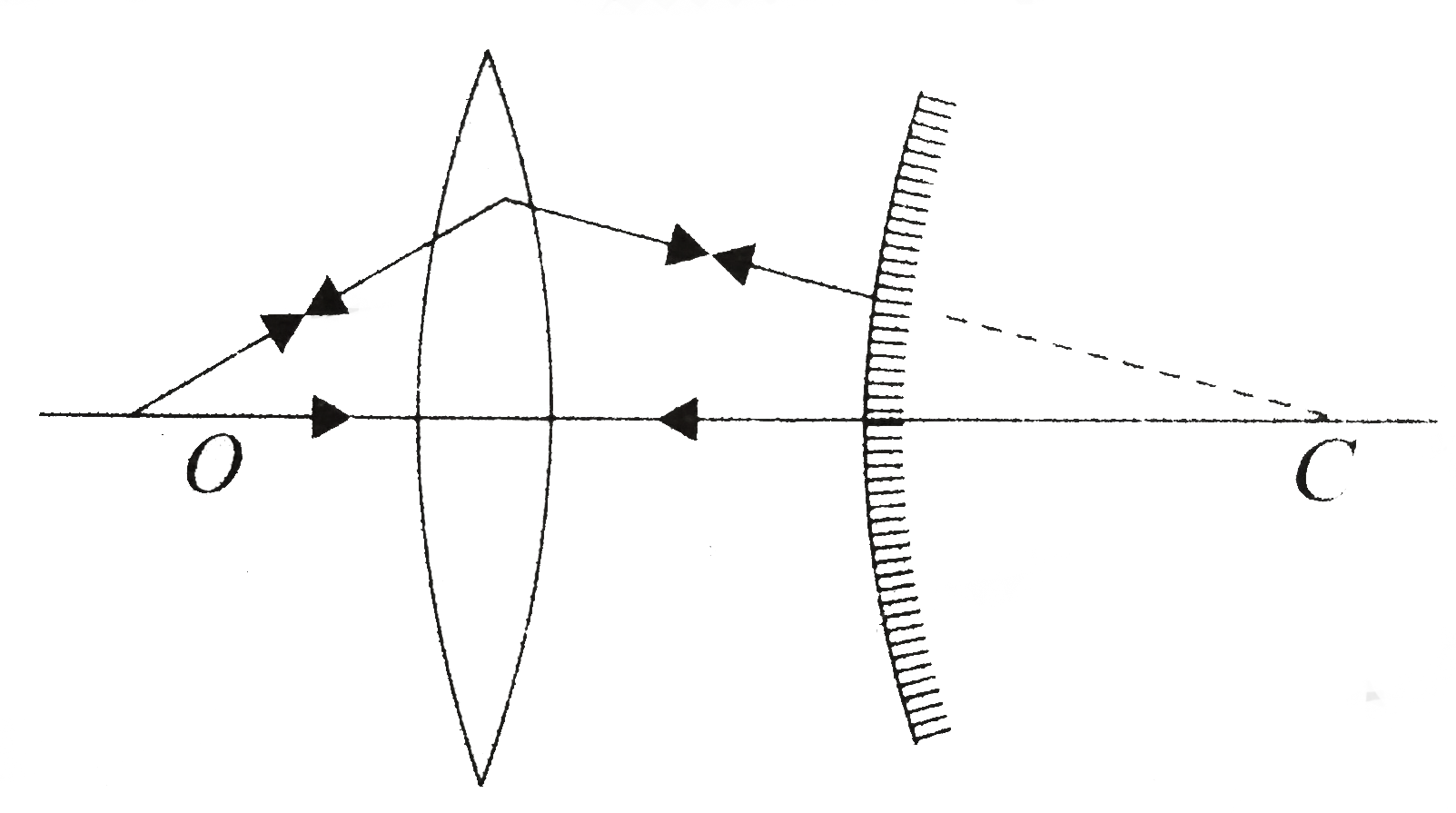
A convex lens and a convex mirror of radius of curvature 20cm are placed co-axially with the convex mirror placed at a distance of 30cm from the lens. For a point object at a distance of 25cm from the lens, the final image due to this combination coincides with the object itself. What is the focal length of convex lens ?
To find the focal length of the convex lens in the given setup, we can follow these steps:
Step 1: Understand the Setup
We have a convex lens and a convex mirror placed coaxially. The radius of curvature (R) of the convex mirror is given as 20 cm. The distance between the lens and the mirror is 30 cm, and there is a point object located 25 cm from the lens.
Step 2: Determine the Focal Length of the Convex Mirror
The focal length (f) of a mirror is related to its radius of curvature (R) by the formula:
f=R2
For the convex mirror:
fmirror=20cm2=10cm
Since it is a convex mirror, the focal length is positive:
fmirror=+10cm
Step 3: Find the Image Distance from the Mirror
The object is located 25 cm from the lens, and the distance from the lens to the mirror is 30 cm. Therefore, the distance of the object from the mirror (u_mirror) can be calculated as:
umirror=30cm−25cm=5cm
Since the object is in front of the mirror, we consider this distance as negative:
umirror=−5cm
Step 4: Use the Mirror Formula to Find the Image Distance
The mirror formula is given by:
1f=1v+1u
Here, f=+10cm and u=−5cm. Rearranging the formula gives:
1v=1f−1u
Substituting the values:
1v=110−1−5
1v=110+15
Converting 15 to a common denominator:
15=210
So,
1v=110+210=310
Thus,
v=103cm
Step 5: Find the Image Distance from the Lens
The image formed by the mirror acts as a virtual object for the lens. The distance from the lens to this image (v_lens) is:
vlens=30cm+103cm=903+103=1003cm
Step 6: Use the Lens Formula to Find the Focal Length
The lens formula is:
1f=1v−1u
Where:
- v=1003cm (positive since the image is on the opposite side of the lens)
- u=−25cm
Substituting these values into the lens formula:
1f=3100−1−25
Converting −125 to a common denominator:
−125=−4100
So,
1f=3100+4100=7100
Thus,
f=1007cm≈14.29cm
Final Answer
The focal length of the convex lens is approximately 14.29cm.
---
We have a convex lens and a convex mirror placed coaxially. The radius of curvature (R) of the convex mirror is given as 20 cm. The distance between the lens and the mirror is 30 cm, and there is a point object located 25 cm from the lens.
The focal length (f) of a mirror is related to its radius of curvature (R) by the formula:
f=R2
For the convex mirror:
fmirror=20cm2=10cm
Since it is a convex mirror, the focal length is positive:
fmirror=+10cm
The object is located 25 cm from the lens, and the distance from the lens to the mirror is 30 cm. Therefore, the distance of the object from the mirror (u_mirror) can be calculated as:
umirror=30cm−25cm=5cm
Since the object is in front of the mirror, we consider this distance as negative:
umirror=−5cm
The mirror formula is given by:
1f=1v+1u
Here, f=+10cm and u=−5cm. Rearranging the formula gives:
1v=1f−1u
Substituting the values:
1v=110−1−5
1v=110+15
Converting 15 to a common denominator:
15=210
So,
1v=110+210=310
Thus,
v=103cm
The image formed by the mirror acts as a virtual object for the lens. The distance from the lens to this image (v_lens) is:
vlens=30cm+103cm=903+103=1003cm
The lens formula is:
1f=1v−1u
Where:
- v=1003cm (positive since the image is on the opposite side of the lens)
- u=−25cm
1f=3100−1−25
Converting −125 to a common denominator:
−125=−4100
So,
1f=3100+4100=7100
Thus,
f=1007cm≈14.29cm
The focal length of the convex lens is approximately 14.29cm.
Topper's Solved these Questions
- RAY OPTICSBOOK - PRADEEPCHAPTER - RAY OPTICSEXERCISE - Solved Example(c )
21 Videos
- RAY OPTICSBOOK - PRADEEPCHAPTER - RAY OPTICSEXERCISE - Solved Example(d)
32 Videos
- RAY OPTICSBOOK - PRADEEPCHAPTER - RAY OPTICSEXERCISE - Sample problem(d)
5 Videos
- PROPERTIES OF BULK MATTERBOOK - PRADEEPCHAPTER - PROPERTIES OF BULK MATTEREXERCISE - Multiple choice questions
7 Videos
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - PRADEEPCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Assertion- Reason Type questions
20 Videos
Similar Questions
A convex lens is placed in contact with a plane mirror. An axial point object at a distance of 20cm from this combination, has its image coinciding with itself. What is the focal length of the convex lens ?
View SolutionA convex lens is placed in contact with a plane mirror. A point object at a distance of 20 cm on the axis of this combination has its image coinciding with itself. What is the focal length of the lens ?
View Solution
Knowledge Check
A point object is placed at a distance of 12cm from a convex lens of focal length 10cm. On the other side of the lens, a convex mirror is placed at a distance of 10cm from the lens such that the image formed by the combination coincides with the object itself. The focal length of the convex mirror is
A20cmB25cmC15cmD30cmAn object is placed at a distance of 15cm from a convex lenx of focal length 10cm. On the other side of the lens, a convex mirror is placed at its focus such that the image formed by the combination coincides with the object itself. The focal length of the convex mirror is
A20cmB10cmC15cmD30cm
A convex lens is placed in contact with a plane mirror. An axial point object at a distance of 20cm from this combination, has its image coinciding with itself. What is the focal length of the convex lens ?
View SolutionAn object is placed at a distance of 30 cm from a convex lens of focal length 20 cm.
View SolutionA convex lens of focal length 15cm, and a concave mirror of radius of curvature 20cm are placed co-axially 10cm apart. An object is placed in front of convex lens so that there is no parallax between the object and its image formed by the combination. Find the position of the object.
View SolutionAn object if placed at a distance of 15cm from a convex lens of focal length 10cm on the other side of the lens, a convex mirror is placed at its foxus such that the image formed by combination coincides with the object itself. Find the focal length of concave mirror.
View SolutionFind the focal length of a convex lens which produces a virtual image at 10 cm from the lens when an object is placed at 5 cm from the lens.
View SolutionAn object is placed at a distance of 30 cm from a convex lens of focal length 20 cm.Find the position of the image .
View Solution
