A person can see the objects lying between from his eye. His vision can be corrected by using lens of power , Is the statement true or false ?
The correct Answer is:1
To determine whether the statement is true or false, we need to analyze the given information about the person's vision and the lens required to correct it.
1. Understanding the Vision Range:
The person can see objects between 25 cm (0.25 m) and 10 m. This means:
- The near point (minimum distance) is 25 cm.
- The far point (maximum distance) is 10 m.
2. Normal Vision Range:
A person with normal vision can see objects clearly from a distance of 25 cm (near point) to infinity (far point). In this case, the person cannot see objects beyond 10 m clearly.
3. Determining the Correction Needed:
To correct the vision for distant objects, we need to bring the image of distant objects (at infinity) to the person's far point, which is 10 m.
4. Using the Lens Formula:
The lens formula is given by:
1f=1v−1u
Here,
- v is the image distance (10 m, since we want to form the image at the far point).
- u is the object distance (for distant objects, we can take u as infinity).
Substituting these values into the lens formula:
1f=110−1∞
Since 1∞=0, we have:
1f=110
Therefore, the focal length f is:
f=10 m
5. Calculating the Power of the Lens:
The power P of a lens is given by:
P=1f (in meters)
Since f=10 m, we convert it to diopters:
P=110=0.1 D
However, since the lens is required to correct for myopia (nearsightedness), the power will be negative:
P=−0.1 D
6. Conclusion:
The statement in the question claims that the vision can be corrected by using a lens of power -0.1 D. Since our calculations confirm that the required power of the lens is indeed -0.1 D, the statement is true.
Final Answer:
True
The person can see objects between 25 cm (0.25 m) and 10 m. This means:
- The near point (minimum distance) is 25 cm.
- The far point (maximum distance) is 10 m.
A person with normal vision can see objects clearly from a distance of 25 cm (near point) to infinity (far point). In this case, the person cannot see objects beyond 10 m clearly.
To correct the vision for distant objects, we need to bring the image of distant objects (at infinity) to the person's far point, which is 10 m.
The lens formula is given by:
1f=1v−1u
Here,
- v is the image distance (10 m, since we want to form the image at the far point).
- u is the object distance (for distant objects, we can take u as infinity).
1f=110−1∞
Since 1∞=0, we have:
1f=110
Therefore, the focal length f is:
f=10 m
The power P of a lens is given by:
P=1f (in meters)
Since f=10 m, we convert it to diopters:
P=110=0.1 D
However, since the lens is required to correct for myopia (nearsightedness), the power will be negative:
P=−0.1 D
The statement in the question claims that the vision can be corrected by using a lens of power -0.1 D. Since our calculations confirm that the required power of the lens is indeed -0.1 D, the statement is true.
True
Topper's Solved these Questions
- BOOK - PRADEEPCHAPTER - RAY OPTICSEXERCISE - (Test Your Grip)Multiple Choice (b)10videos
RAY OPTICS
- BOOK - PRADEEPCHAPTER - RAY OPTICSEXERCISE - (Test Your Grip)Multiple Choice (c)10videos
RAY OPTICS
- BOOK - PRADEEPCHAPTER - RAY OPTICSEXERCISE - Problem For Practice(c)27videos
RAY OPTICS
- BOOK - PRADEEPCHAPTER - PROPERTIES OF BULK MATTEREXERCISE - Multiple choice questions7videos
PROPERTIES OF BULK MATTER
- BOOK - PRADEEPCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Assertion- Reason Type questions20videos
SYSTEMS OF PARTICLES AND ROTATIONAL MOTION
Similar Questions
What is the refractive index of a medium in which light travels with a speed of 2×108m/s ?
02:22View SolutionWhich of the following waves have the maximum wavelength?
02:36View SolutionCalculate the refractive index of the material of an equilaterial prism for which angle of minimum deviation is 60∘.
01:01View SolutionIn the experiment to determine Young's modulus of the material of a wire under tension
used in the arrangement as shown. The percentage error in the measurement
of length is a in the measurement of the radius of the wire is b and in the
measurement of the change in length of the wire is c. Percentage error in the measurement
of Young's modulus for a given load is
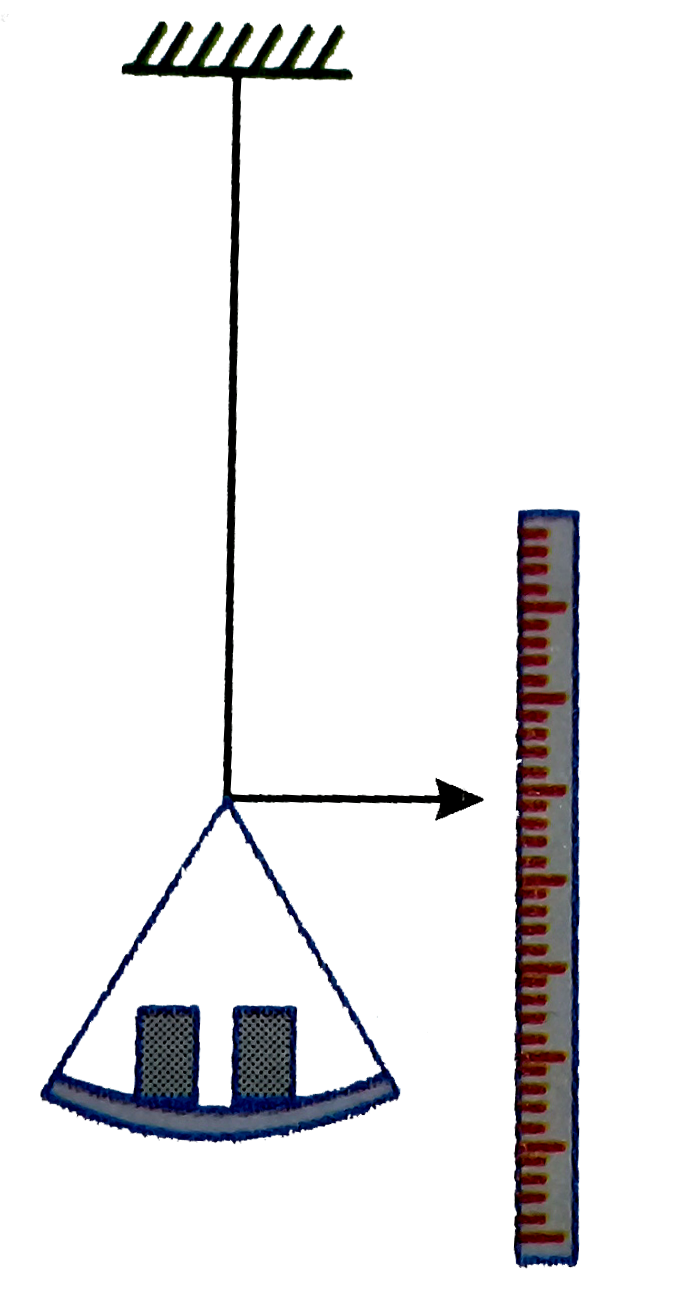 05:29View Solution
05:29View SolutionAssertion : In rolling, all points of a rigid body have the same linear velocity.
Reason : The rotational motion does not affect the linear velocity.04:36View SolutionA man can see the objects upto a distance of one metre from his eyes. For correcting his eye sight so that he can see an object at infinity, he requires a lens whose power is
06:31View SolutionA person can not see objects beyond 50cm.The power of a lens to correct this vision will be
View SolutionA person cannot see objects clearly beyond 2.0 m . The power of lens required to correct his vision will be
View Solutionएक व्यक्ति अपनी आँख से केवल 50 सेमी तथा 400 सेमी दुरी के बीच स्थित वस्तुओं को ही सुस्पष्ट देख सकता है | सुस्पष्ट देखने की अधिकतम दुरी को अनन्त तक करने के लिए उस व्यक्ति को आवश्यक लेंस व उसकी क्षमता होगी :
View SolutionA boy can see the objects distinctly only in between 15 cm and 200 cm from his eyes . What should be the power of the lens (in m^(-1) unit ) he will use to see the objects at infinity distinctly ? What will the least distance of distinct vision of his eyes with spectacles ?
View SolutionA person can see clearly objects between 15 and 100 cm from his eye. The range of his vision if he wears close fitting spetancles having a power of -0.8 diopter is
03:52View SolutionA myopic person can see things clearly lying between 8 cm and 100 cm from his eyes. The lens which enables his to see the moon should have a focal length of :
01:58View SolutionA person can see clearly objects between 15 and 100 cm from his eye. Find the range of his vision is he wears close fitting spectacles having a power of 0.8 diopter :
02:14View SolutionA person cannot see object clearly beyond 2.0 m. The power of lens requred to correct his vision will be :
01:29View SolutionA person can see clearly objects only whenthey lie between 50cm and 400cm from his eyes. In order to increase the maximum distance of distinct vision of infinity, the type and power of the correcting lens, the person has to use, will be
View Solution

