Two identical magnetic dipoles of magnetic moments 1⋅0Am2 each are placed at a separation of 2m with their axes perpendicular to each other. What is the resultant magnetic field at a point midway between the dipoles?
To find the resultant magnetic field at a point midway between two identical magnetic dipoles with magnetic moments of 1Am2 each, placed at a separation of 2m with their axes perpendicular to each other, we can follow these steps:
Step 1: Understand the Configuration
- We have two magnetic dipoles, each with a magnetic moment m=1Am2.
- The distance between the two dipoles is d=2m.
- The point of interest is midway between the two dipoles, which means the distance from each dipole to the point is 1m.
Step 2: Calculate the Magnetic Field due to Each Dipole
- The magnetic field B at a distance r along the axis of a dipole is given by:
B=μ04π⋅2mr3
- The magnetic field at a point on the perpendicular bisector of a dipole is given by:
B=μ04π⋅mr3
Step 3: Calculate the Magnetic Field from Dipole 1
- For the first dipole, at a distance r=1m:
B1=μ04π⋅2m(1)3=μ04π⋅2⋅1
- Substituting μ0=4π×10−7T m/A:
B1=(4π×10−7)4π⋅2=2×10−7T
Step 4: Calculate the Magnetic Field from Dipole 2
- For the second dipole, at the same distance r=1m:
B2=μ04π⋅m(1)3=μ04π⋅1
- Substituting μ0=4π×10−7T m/A:
B2=(4π×10−7)4π=10−7T
Step 5: Determine the Resultant Magnetic Field
- The two magnetic fields B1 and B2 are perpendicular to each other.
- The resultant magnetic field Bnet can be calculated using the Pythagorean theorem:
Bnet=√B21+B22
- Substituting the values:
Bnet=√(2×10−7)2+(10−7)2=√4×10−14+10−14=√5×10−14
- Therefore:
Bnet=√5×10−7T
Final Answer
The resultant magnetic field at the point midway between the dipoles is:
Bnet=√5×10−7T
- We have two magnetic dipoles, each with a magnetic moment m=1Am2.
- The distance between the two dipoles is d=2m.
- The point of interest is midway between the two dipoles, which means the distance from each dipole to the point is 1m.
- The magnetic field B at a distance r along the axis of a dipole is given by:
B=μ04π⋅2mr3
- The magnetic field at a point on the perpendicular bisector of a dipole is given by:
B=μ04π⋅mr3
- For the first dipole, at a distance r=1m:
B1=μ04π⋅2m(1)3=μ04π⋅2⋅1
- Substituting μ0=4π×10−7T m/A:
B1=(4π×10−7)4π⋅2=2×10−7T
- For the second dipole, at the same distance r=1m:
B2=μ04π⋅m(1)3=μ04π⋅1
- Substituting μ0=4π×10−7T m/A:
B2=(4π×10−7)4π=10−7T
- The two magnetic fields B1 and B2 are perpendicular to each other.
- The resultant magnetic field Bnet can be calculated using the Pythagorean theorem:
Bnet=√B21+B22
- Substituting the values:
Bnet=√(2×10−7)2+(10−7)2=√4×10−14+10−14=√5×10−14
- Therefore:
Bnet=√5×10−7T
The resultant magnetic field at the point midway between the dipoles is:
Bnet=√5×10−7T
Topper's Solved these Questions
- MAGNETIC EFFECT OF CURRENT AND MAGNETISMBOOK - PRADEEPCHAPTER - MAGNETIC EFFECT OF CURRENT AND MAGNETISMEXERCISE - Conceptual Problems (d)
2 Videos
- MAGNETIC EFFECT OF CURRENT AND MAGNETISMBOOK - PRADEEPCHAPTER - MAGNETIC EFFECT OF CURRENT AND MAGNETISMEXERCISE - Very Short Answer Questions (a)
2 Videos
- ELECTROSTATICSBOOK - PRADEEPCHAPTER - ELECTROSTATICSEXERCISE - ASSERTION-REASON TYPE QUESTIONS
2 Videos
- OPTICSBOOK - PRADEEPCHAPTER - OPTICSEXERCISE - Multiple choice questions
1 Video
Similar Questions
Two identical magnetic dipoles of magnetic moment 2Am2 are placed at a separation of 2m with their axes perpendicular to each other in air. The resultant magnetic field at a midpoint between the dipoles is x√y×10−7T.F∈d(x+y).
View SolutionFigure shows two identical magnetic dipoles aandb of magnetic moments M each, placed at a separation d, with their axes perpendicular to each other. Find the magnetic field at the point P midway between the dipoles.
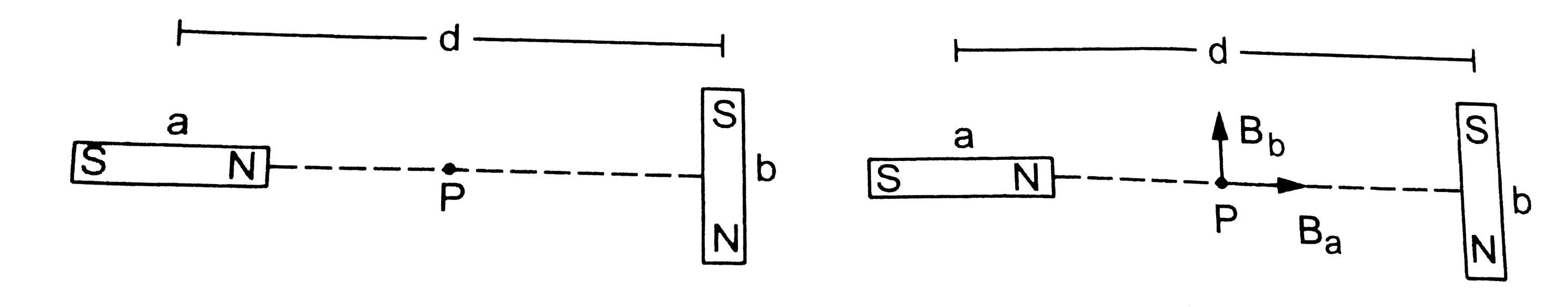
View Solution
Knowledge Check
Two identical magnetic dipoles of magnetic moments 4A−m2 each, are placed at a separation of 4 m with their axis perpendicular to each other. The resultant magnetic field at a point midway between the dipoles is
A5×10−7TB√5×10−7TC10−7TD√52×10−7TTwo identcal magnetic dipole of magnetic moment 1.0A−m2 each, placed at a separation of 2 m with their axis perpendicular to each other. The resultant magnetic field at a point midway between the dipole is
A5×10−7TB√5×10−7TC10−7TDNone of theseTwo identical magnetic dipoles of magnetic moments 2Am2 are placed at a separation of 2m with their axes perpendicular to each other in air. The resultant magnetic field at a mid point between the dipole is
A4√5×10−5TB2√5×10−5TC4√5×10−7TD2√5×10−7T
Two identical bar magnets of magnetic pole strength 10 Am and length 10 cm are placed 2 m apart such that their axes are perpendicular to each other. Calculate the resultant magnetic field at a point midway between the magnets.
View SolutionTwo identical short bar magnets each of magnetic moment 12⋅5Am2 are placed at a separation of 10cm between their centres, such that their axes are perpendicular to each other. Find the magnetic field at a point midway between the two magnets.
View SolutionIwo identical magnetic dipoles of magnetic moment 2 A m2 are placed at a separation of 2 m with their axes perpendicular to each other in air. The resultant inagnetic field at a midpoint between the dipoles is
View SolutionTwo identical short bar magnets, each having magnetic moment M, are placed a distance of 2d apart with axes perpendicular to each other in a horizontal plane. The magnetic induction at a point midway between them is
View SolutionTwo identical short bar magnets, each having magnetic moment M, are placed a distance of 2d apart with axes perpendicular to each other in a horizontal plane. The magnetic induction at a point midway between them is
View Solution