Find the magnetic field induction at a point on the axis of a circular coil carrying current and hence find the magnetic field at the centre of circular coil carrying current.
To find the magnetic field induction at a point on the axis of a circular coil carrying current, we can follow these steps:
Step 1: Understanding the Setup
We have a circular coil of radius R carrying a current I. We want to find the magnetic field induction B at a point located at a distance x along the axis of the coil from its center.
Step 2: Using Biot-Savart Law
The Biot-Savart Law states that the magnetic field dB due to a small current element dL is given by:
dB=μ0I4πdL×RR3
where μ0 is the permeability of free space, R is the position vector from the current element to the point where the field is being calculated, and R is the distance from the current element to that point.
Step 3: Geometry of the Problem
For a circular coil, the distance R from a point on the coil to the point on the axis is given by:
R=√R2+x2
where R is the radius of the coil and x is the distance along the axis from the center of the coil.
Step 4: Components of the Magnetic Field
Due to symmetry, the horizontal components of the magnetic field from opposite elements of the coil will cancel out. Therefore, we only need to consider the vertical components. The vertical component of the magnetic field dB is given by:
dBy=dBsinθ
where θ is the angle between dL and R.
Using the geometry, we find:
sinθ=R√R2+x2
Step 5: Integrating Over the Coil
The total magnetic field B at the point on the axis is obtained by integrating dBy around the entire coil:
B=∫dBy=∫μ0I4πdL⋅RsinθR3
Substituting dB and sinθ:
B=∫μ0I4πdL⋅R⋅R√R2+x2(R2+x2)3/2
Step 6: Evaluating the Integral
The length of the coil is 2πR, so:
B=μ0IR4π⋅2πR(R2+x2)3/2
This simplifies to:
B=μ0IR22(R2+x2)3/2
Step 7: Magnetic Field at the Center of the Coil
To find the magnetic field at the center of the coil, we set x=0:
Bcenter=μ0IR22(R2)3/2=μ0I2R
Final Result
Thus, the magnetic field induction at a point on the axis of a circular coil carrying current is:
B=μ0IR22(R2+x2)3/2
And the magnetic field at the center of the coil is:
Bcenter=μ0I2R
We have a circular coil of radius R carrying a current I. We want to find the magnetic field induction B at a point located at a distance x along the axis of the coil from its center.
The Biot-Savart Law states that the magnetic field dB due to a small current element dL is given by:
dB=μ0I4πdL×RR3
where μ0 is the permeability of free space, R is the position vector from the current element to the point where the field is being calculated, and R is the distance from the current element to that point.
For a circular coil, the distance R from a point on the coil to the point on the axis is given by:
R=√R2+x2
where R is the radius of the coil and x is the distance along the axis from the center of the coil.
Due to symmetry, the horizontal components of the magnetic field from opposite elements of the coil will cancel out. Therefore, we only need to consider the vertical components. The vertical component of the magnetic field dB is given by:
dBy=dBsinθ
where θ is the angle between dL and R.
sinθ=R√R2+x2
The total magnetic field B at the point on the axis is obtained by integrating dBy around the entire coil:
B=∫dBy=∫μ0I4πdL⋅RsinθR3
B=∫μ0I4πdL⋅R⋅R√R2+x2(R2+x2)3/2
The length of the coil is 2πR, so:
B=μ0IR4π⋅2πR(R2+x2)3/2
This simplifies to:
B=μ0IR22(R2+x2)3/2
To find the magnetic field at the center of the coil, we set x=0:
Bcenter=μ0IR22(R2)3/2=μ0I2R
Thus, the magnetic field induction at a point on the axis of a circular coil carrying current is:
B=μ0IR22(R2+x2)3/2
And the magnetic field at the center of the coil is:
Bcenter=μ0I2R
Topper's Solved these Questions
- MAGNETIC EFFECT OF CURRENT AND MAGNETISMBOOK - PRADEEPCHAPTER - MAGNETIC EFFECT OF CURRENT AND MAGNETISMEXERCISE - Conceptual Problems (d)
2 Videos
- MAGNETIC EFFECT OF CURRENT AND MAGNETISMBOOK - PRADEEPCHAPTER - MAGNETIC EFFECT OF CURRENT AND MAGNETISMEXERCISE - Very Short Answer Questions (a)
2 Videos
- ELECTROSTATICSBOOK - PRADEEPCHAPTER - ELECTROSTATICSEXERCISE - ASSERTION-REASON TYPE QUESTIONS
2 Videos
- OPTICSBOOK - PRADEEPCHAPTER - OPTICSEXERCISE - Multiple choice questions
1 Videos
Similar Questions
Knowledge Check
The magnetic field at the centre of current carrying coil is
Aμ0ni2rBμ02πnirCμ0ni4rDμ0niThe magnetic moment of a circular coil carrying current is
ADirectly proportional to the length of the wire in the coilBInversely proportional to the length of the wire in the coilCDirectly proportional to the square of the length of the wire in the coilDInversely proportional to the square of the length of the wire in the coilA flat circular coil, carrying a current, has a magnetic moment μ
Aμ has only magnitude, it does not have directionBThe direction of μ is along the normal to the plane of the coilCThe direction of μ depends on the direction of the current flowDThe direction of μ does not change if the current in the coil is reversed
Magnetic field induction at the centre of a circular coil shown in the figure is
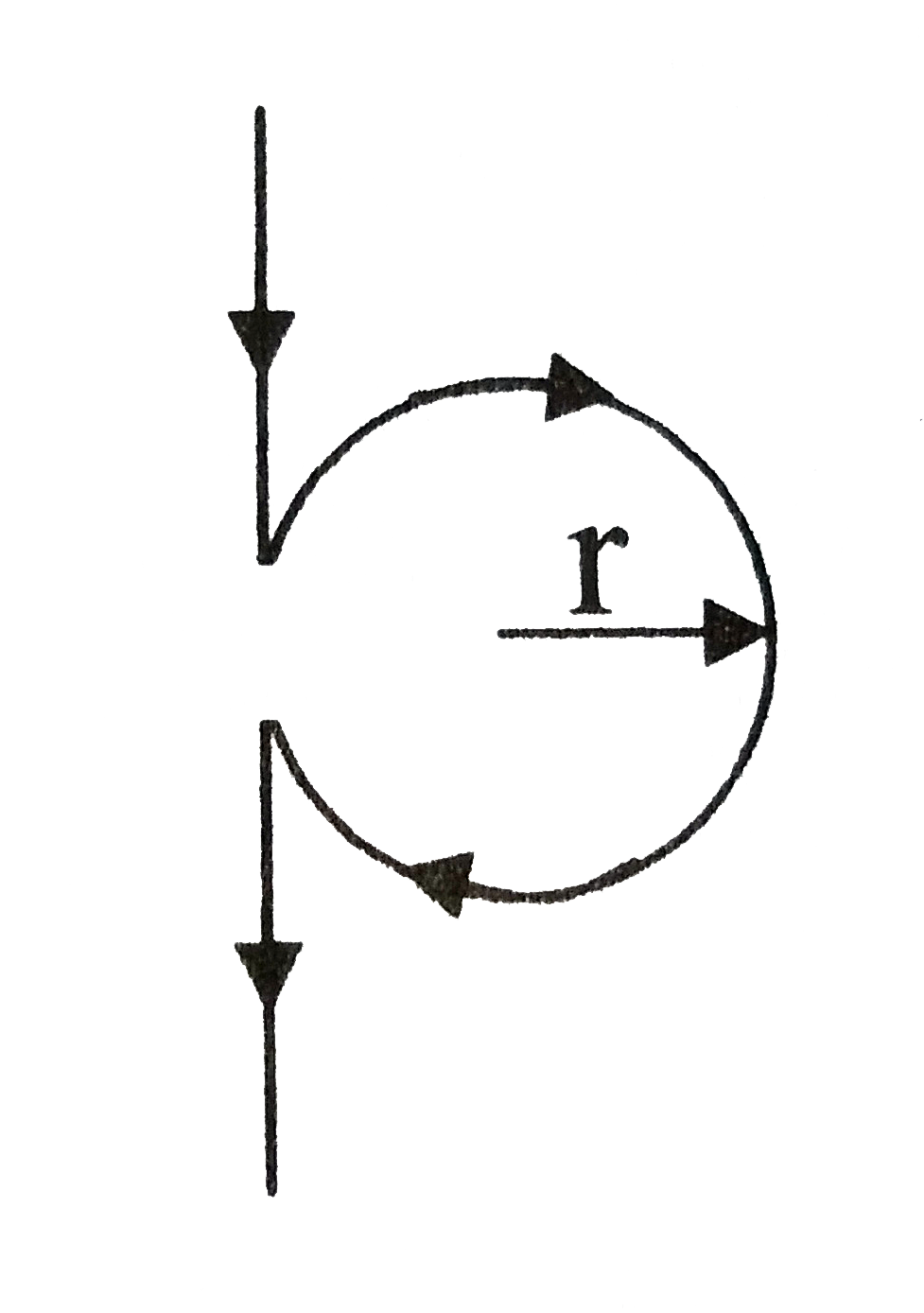
View SolutionUsing Biot-Savart’s law, derive an expression for magnetic field at any point on axial line of a current carrying circular loop. Hence, find magnitude of magnetic field intensity at the centre of circular coil.
View SolutionUsing Biot - Savart's law, derive an expression for magnetic field at any point on axial line of a current carrying circular loop. Hence, find magnitude of magnetic field intensity at the centre of circular coil.
View SolutionThe magnetic field at centre of a hexagonal coil of side l carrying a current I is

View SolutionThe magnetic induction at a point on the axis of a circular current carrying coil at a distance equal to the radius of coil carrying a current of 0.5 A is
View Solution
