If the current density in a linear conductor of radius 'a' varies with r according to relation $J = k r^{2}$ , where k is a constant and r is the distance of a point from the axis of conductor. Find the magnetic field induction at a point distance r from the axis, when (i) $r < a$ and (ii) $r > a$ .

Video Solution

Text Solution

Verified by Experts

The correct Answer is:(i) $\frac{μ_{0} μ_{r} k r^{3}}{r}$ (ii) $\frac{μ_{0} k a^{4}}{4 r}$

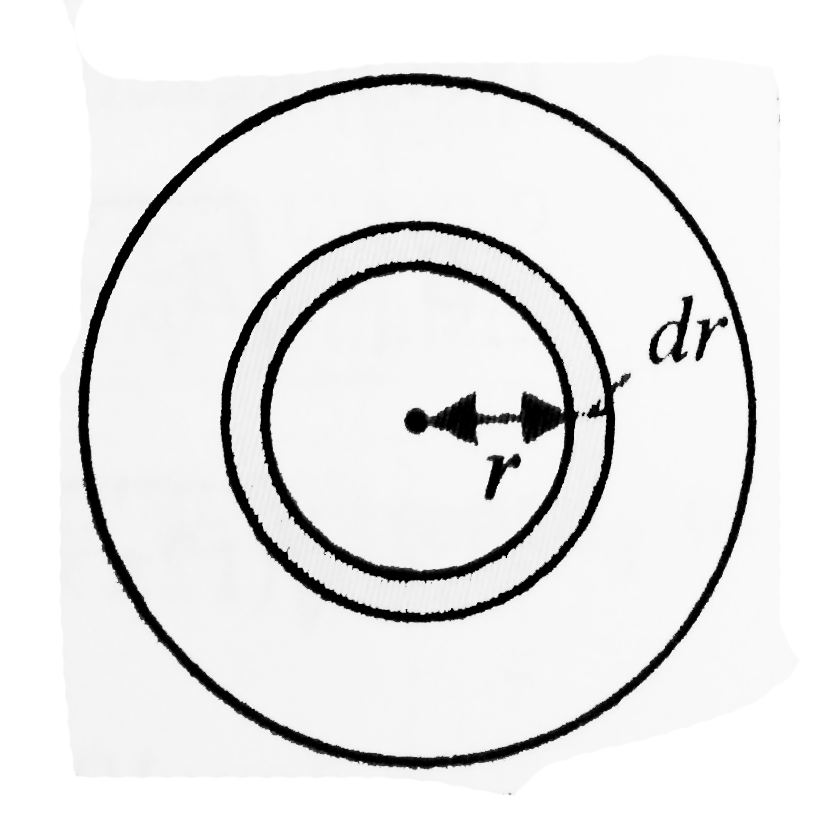
(i) Consider an elementary ring of radius r thickness dr whose centre lies on the axis of the conductor. Area of the elementary ring, $d A = 2 π r d r$ . Current passing through the elementary ring is
$d I = J d A = (k r^{2}) (2 π r d r) = k 2 π r^{3} d r$
Total current passing through the closed path of radius r is,
$I = \int_{0}^{r} k 2 π r^{3} d r = \frac{k π r^{4}}{2}$
Using Ampere circuital law, we have
$\oint \vec{B} . d \vec{l} = μ_{0} μ_{r} I$
$B 2 π r = μ_{0} μ_{r} \frac{π k r^{4}}{2}$ or $B = \frac{μ_{0} μ_{r} k r^{3}}{4}$
(ii) If $r > a$ , then net current through the closed path of radius r is,
$I = \int_{0}^{a} k 2 π r^{3} d r = \frac{π k a^{4}}{2}$
Using Ampere circuital law, we have
$\oint \vec{B} . d \vec{l} = μ_{0} I$ or $B 2 π r = \frac{μ_{0} π k a^{4}}{2}$
$B = \frac{μ_{0} k a^{4}}{4 r}$

|

Updated on:21/07/2023

Class 12 PHYSICS MOVING CHARGES AND MAGNETISM

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Ampere's law provides us an easy way to calculate the magnetic field due to a symmetrical distribution of current. Its mathemfield expression is $\oint \to B . d l = μ_{0} I_{in}$ .
The quantity on the left hand side is known as line as integral of magnetic field over a closed Ampere's loop.
If the current density in a linear conductor of radius a varies with r according to relation $J = k r^{2}$ , where k is a constant and r is the distance of a point from the axis of conductor, find the magnetic field induction at a point distance r from the axis when rlta. Assume relative permeability of the conductor to be unity.
A $\frac{μ_{0} k a^{4}}{4 r}$
B $\frac{μ_{0} k r^{3}}{2}$
C $\frac{μ_{0} k π a^{4}}{2 r}$
D $\frac{μ_{0} k a^{3}}{4}$
Question 2 - Select One
The current density in a wire of radius a varies with radial distance r as $J = \frac{J_{0} r^{2}}{a}$ , where $J_{0}$ is a constant. Choose the incorrect statement.
ATotal current passing through the cross-section of the wire is $I = \frac{π J_{0} a^{3}}{2}$
BTotal current passing through the cross-section of the wire is $I = \frac{3 π J_{0} a^{3}}{2}$
CThe field at a distance r gt a is $B = \frac{μ_{0} J_{0} a^{3}}{4 r}$
DThe field at a distance r gt a is $B = \frac{μ_{0} J_{0} a^{3}}{4 a}$
Question 3 - Select One
Let $r$ be the distance of a point on the axis of a bar magnet from its centre. The magnetic field at such a point is proportional to
A $\frac{1}{r}$
B1/r^2
C1/r^3
Dnone of these

PRADEEP-MAGNETIC EFFECT OF CURRENT AND MAGNETISM-Exercise

Home
Ask Doubt
Profile