A galvanometer can be converted into a voltmeter of certain range by connecting a resistance of in series with it. When the resistance of is connected in series, the range becomes half. Find the resistance of galvanometer.
The correct Answer is:
To solve the problem, we need to find the resistance of the galvanometer (let's denote it as G). We are given two scenarios with different series resistances and their effects on the voltage range of the galvanometer.
1. Define the Variables:
- Let G be the resistance of the galvanometer.
- The first series resistance R1=880Ω.
- The second series resistance R2=420Ω.
- The full-scale deflection current of the galvanometer is denoted as Ig.
- The voltage across the galvanometer when using R1 is V, and when using R2, it becomes V2.
2. Write the Current Equations:
- For the first case (using R1):
Ig=VR1+G=V880+G
- For the second case (using R2):
Ig=V/2R2+G=V/2420+G
3. Set the Two Equations Equal:
Since both expressions represent the same current Ig, we can set them equal to each other:
V880+G=V/2420+G
4. Eliminate V:
We can eliminate V from the equation by multiplying both sides by 2(880+G)(420+G):
2V(420+G)=V(880+G)
Simplifying gives:
2(420+G)=880+G
5. Expand and Rearrange:
Expanding the left side:
840+2G=880+G
Rearranging gives:
2G−G=880−840
Simplifying further:
G=40Ω
Final Answer:
The resistance of the galvanometer G is 40Ω.
- Let G be the resistance of the galvanometer.
- The first series resistance R1=880Ω.
- The second series resistance R2=420Ω.
- The full-scale deflection current of the galvanometer is denoted as Ig.
- The voltage across the galvanometer when using R1 is V, and when using R2, it becomes V2.
- For the first case (using R1):
Ig=VR1+G=V880+G
- For the second case (using R2):
Ig=V/2R2+G=V/2420+G
Since both expressions represent the same current Ig, we can set them equal to each other:
V880+G=V/2420+G
We can eliminate V from the equation by multiplying both sides by 2(880+G)(420+G):
2V(420+G)=V(880+G)
Simplifying gives:
2(420+G)=880+G
Expanding the left side:
840+2G=880+G
Rearranging gives:
2G−G=880−840
Simplifying further:
G=40Ω
The resistance of the galvanometer G is 40Ω.
Topper's Solved these Questions
- BOOK - PRADEEPCHAPTER - MAGNETIC EFFECT OF CURRENT AND MAGNETISMEXERCISE - Test Your Grip (a)1videos
MAGNETIC EFFECT OF CURRENT AND MAGNETISM
- BOOK - PRADEEPCHAPTER - MAGNETIC EFFECT OF CURRENT AND MAGNETISMEXERCISE - Test Your Grip (c)1videos
MAGNETIC EFFECT OF CURRENT AND MAGNETISM
- BOOK - PRADEEPCHAPTER - MAGNETIC EFFECT OF CURRENT AND MAGNETISMEXERCISE - Value Based Questions2videos
MAGNETIC EFFECT OF CURRENT AND MAGNETISM
- BOOK - PRADEEPCHAPTER - ELECTROSTATICSEXERCISE - ASSERTION-REASON TYPE QUESTIONS2videos
ELECTROSTATICS
- BOOK - PRADEEPCHAPTER - OPTICSEXERCISE - Multiple choice questions1videos
OPTICS
Similar Questions
(a) Briefly explain how a galvanometer is converted into a voltmeter.
(b) A voltmeter of a certain range is constructed by connecting a resistance of 90 Ω in series with a galvanometer. When the resistance of 470Ω is connected in series, the range gets halved. Find the resistance of the galvanometer.View SolutionState the principle of working of a galvanometer.
A galvanometer of resistance G is converted into a voltmeter to measure upto V volts by connecting resistance R1 in series with the coil. If a resistance R2 is connected in series with it, then it can measure V/2 volts. Find the resistance, in terms of R1 and R2, required to be connected to convert it into a volt meter that can read upto 2 V. Also find the resistance G of the galvanometer in terms of R1 and R2.View Solution
Knowledge Check
- Question 1 - Select One
A galvanometer of resistance 100 Ω is converted to a voltmeter of range 10 y by connecting a resistance of 10 Ω. The resistance required to convert the same galvanometer to an ammeter of range 1 A is
A0.4ΩB0.3ΩC1.2ΩD0.1Ω
A galvanmeter of resistance G is converted into a voltmeter of range 0 -1V by connecting resistance R1 in series with it. The additional resistance that should be connected in series with R1 to increase the range of the voltmeter to 0-2 V will be :
View SolutionA galvanometer of resistance GΩ can be converted into a voltmeter of range 0 - V volts by connecting a resistance RΩ in series with it. How much resistance will be required to change its range to 0−V2 volts ?
View SolutionA galvanometer of resistance G can be converted into a voltmeter of range θ−V volts by connecting a resistance R in series with it. How much resistance will be required to change its range from 0 to V/2?
View Solution(a) State the principle of working of a galvanometer.
(b) Agalvanometer of resistance G is converted into a voltmeter to measure up to V volts by connecting a resistance R1 , in series with the coil. If a resistance R2 is connected in series with it, then it can measure up to V/2 volts. Find the resistance, in terms of R1andR2, required to be connected to convert it into a voltmeter that can read up to 2 V. Also find the resistance G of the galvanometer in terms of R1 and R2.View SolutionA galvanometer of resistance 5Ω can be converted into a voltmeter of range 0 - V voltmeter of range 0 - V volts by connecting a resistance RΩ in series with it. How much resistance will be required to charge its range to 0−V2 volts?
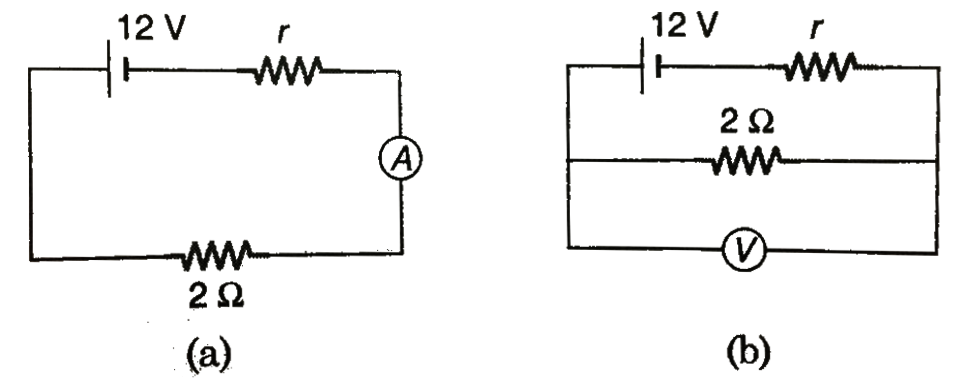
View SolutionA galvanometer (coil resistance 99Ω.) is converted into an ammeter using a shunt of 1Ω and connected as shown in figure (a). The ammeter reads 3A. The same galvanometer is convened into a voltmeter by connecting a resistance of 101Ω in series. This voltmeter is connected at, shown in figure (b). Its reading is found to be 45 of the full scale reading. Find :
(a) internal resistance r of the cell
(b) range of the ammeter and voltmeter
(c) full scale deflection current of the galvanometer.
 View Solution
View SolutionThe resistance of two conductors in series is 18Ω and the resistance becomes 4Ω when connected in parallel. Find the resistance of individual conductors.
View Solution

