Show that average value of radiant flux density 'S' over a single period 'T' is given by S=12cμ0E20.
We know that radiant flux density S (called Poynting vector) is given by
→S=1μ0(→E×→B)=c2∈0(→E×→B)[∵c=1√μ0∈0]
Let the e.m. wave be propagating along x-axis. The electric field vector of e.m. wave be along y-axis and
magnetic field vecotr be along z-axis. Therefore,
→E=→E0cos(kx−ωt)and→B=→B0cos(kx−ωt)∴→E×→B=(→E0×→B0)cos2(kx−ωt)
→S=c2ε0(→E×→B)=c2ε0(→E0×→B0)cos2(kx−ωt)
Average value of the magnitude of radiant flux density over complete cycle is
sav=c2∈0∣∣→E0×→B0∣∣1T∫T0cos2(kx−ωt)dt
=c2∈0E0B0×1T×T2[∵∫T0cos2(kx−ωt)dt=T2]
sav=c22∈0E0(E0c)[Asc=E0/B0]
=c2∈0E20=c2×1c2μ0E20[c=1√μ0∈ror∈0=1c2μ0]
=E202μ0c
Topper's Solved these Questions
- BOOK - PRADEEPCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Solved Example4videos
ELECTROMAGNETIC WAVES
- BOOK - PRADEEPCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - (I) Conceptual problems1videos
ELECTROMAGNETIC WAVES
- BOOK - PRADEEPCHAPTER - ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENTEXERCISE - Multiple Choice Questions1videos
ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENT
- BOOK - PRADEEPCHAPTER - ELECTRONIC DEVICESEXERCISE - Fill in the Blanks1videos
ELECTRONIC DEVICES
Similar Questions
A plane EM wave travelling along z direction is described by
E=E0sin(kz−ωt)ˆiandB=B0sin(kz−ωt)ˆj.
show that
(i) The average energy density of the wave is given by uav=14ε0E20+14B20μ0.
(ii) The time averaged intensity of the wave is given by Iav=12cε0E20.View SolutionShow that average energy density of electromagnetic radiation can be written as ε0E2rms .
View Solution
Knowledge Check
- Question 1 - Select One
Poynting vectors →S is defined as →S=1μ0→E×→B. The average value of '→S' over a single period 'T' is given by
AE20/2cμ0BE20/cμ0C2E022/cμ0DE20/cμ20 - Question 2 - Select One
The average value of current for the current shown for time period 0 to T2 s
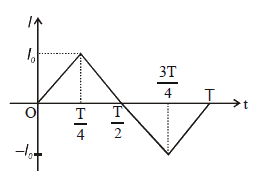 Al0√2Bl02Cl0√3Dl02√3
Al0√2Bl02Cl0√3Dl02√3 - Question 3 - Select One
Assertion (A) : The average value of <sin2ωt> is zero.
Reason (R ) : The average value of function F(t) over a period T is <F(t)>=1T∫T0F(t)dtABoth Assertion and Reason are true and Reason is the correct explanation of Assertion.BBoth Assertion and Reason are true but Reason is not the correct explanation of Assertion.CAssertion is true but Reason is falseDAssertion is false but Reason is true
A closed coil having 50 turns, area 300cm2, is rotated from a position where it plane makes an angle of 45∘ with a magnetic field of flux density 2.0T to a position perpendlcular to the field in a time of 0.1s. What is the average EMF induced in the coil ?
View SolutionShow that the entropy of a perfect gas is given by S=CVInT+RInV+S0
View SolutionDetermine the average value of the electromotive force Em over one period, ie, over the time from t= 0 to t=T, if electromotive force is computed by the formula
E=E0 sin 2πtT
where T is the duration of the period in seconds, E0 the amplitude (the maximum value) of the electromotive force corresponding to the value t= 0.25T. The fraction 2πtT is called the phase.View SolutionThe average value of a saw-tooth voltage V=V0(2tT−1) Over 0 to T2, (T- time period) is given by
View SolutionA particle performing S.H.M. with amplitude 'A' and period T. The average values of magnitude of distance over a half period is
View Solution

