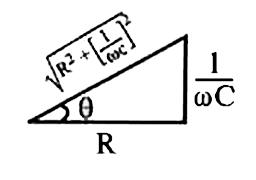
For an A.C. Circuit, contianing a resistance and a capacitance in series, the angle between current and e.m.f. is given by $tan θ = 1 (ω C R)$ . The power factor of this circuit is

A

$ω C R$

B

$\frac{R}{ω C}$

C

$\frac{R}{\sqrt{R^{2} + {(\frac{1}{ω C})}^{2}}}$

D

$\frac{R}{\sqrt{R + \frac{1}{ω C}}}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

$tan θ = \frac{1}{\frac{ω C}{R}}$
$∴ cos θ = \frac{R}{\sqrt{R^{2} + {(\frac{1}{ω C})}^{2}}}$

|

Updated on:21/07/2023

Class 12 PHYSICS ELECTROMAGNETIC INDUCTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
In an $A . C$ circuit having resistance and capacitance
Aemf leads the current
Bcurrent lags behind the emf
Cboth the current and emf are in phase
Dcurrent leads the emf.
Question 2 - Select One
If an alternating e.m.f. is applied to a series L-R circuit, the phase angle between e.m.f. and current is given by $tan θ = \frac{ω L}{R}$ . Hence the power factor of the series L-R circuit is
A1. $\frac{ω L}{R}$
B2. $\frac{R}{ω L}$
C3. $\frac{R}{R + ω L}$
D4. $\frac{R}{\sqrt{R^{2} + ω^{2} L^{2}}}$
Question 3 - Select One
In an A.C. Circuit containing an inductance and a capacitance in series, the current is found to be maximum, when $L = 0.5 H$ and $C = 8 μ F$ . Then the angular frequency of the applied alternating e.m.f. will be
A $25 r a d / sec$
B $500 r a d / sec$
C $750 r a d / sec$
D $1000 r a d / sec$

MARVEL PUBLICATION-ELECTROMAGNETIC INDUCTION AND ALTERNATING CURRENTS -TEST YOUR GRASP - 16

Home
Ask Doubt
Profile