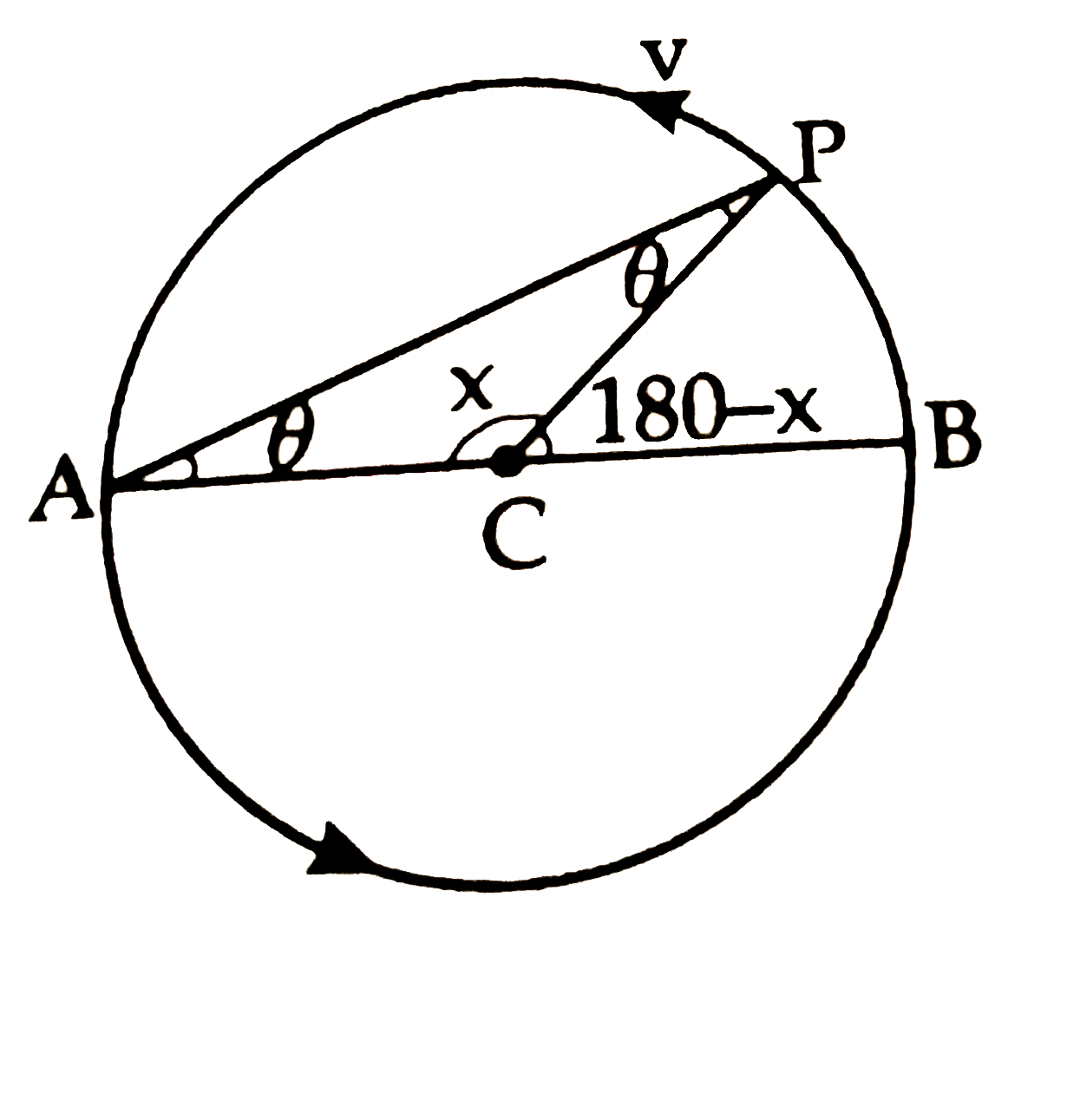
A particle P is moving in a circle of radius r with a uniform speed u. C is the centre of the circle and AB is diameter. The angular velocity of P about A and V are in the ratio :

A

$1 : 1$

B

$1 : 2$

C

$2 : 1$

D

$4 : 2$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

$A C = C P$ =radius of the circle
$∴ ∠ C A P and C P A = θ$ .
from $Δ A C P,$
$θ + θ \times = 180$
$∴ \times = 180 - 20$
$∴ ∠ B C P = 180 - x$
$∴ = 2 θ$
The angular velocity of p w .r t to A is ,
$ω_{P A} = \frac{θ}{t}$
The angular velocity of Pw.r.t to C is
$ω_{P C} = \frac{2 θ}{t}$
$∴ \frac{ω_{P A}}{ω_{P C}} = \frac{θ}{t} \times \frac{t}{2 θ} = \frac{1}{2}$

|

Updated on:21/07/2023

Class 12 PHYSICS CIRCULAR MOTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A particle P is moving in a circle of radius a with a uniform speed u, C is the centre of the circle and AB is a diameter. The angular velocities of P about A and C are in the ratio
A $1 : 1$
B $1 : 2$
C $2 : 1$
D $4 : 1$
Question 2 - Select One
A particle P is moving in a circle of radius r with a uniform speed u. C is the centre of the circle and AB is diameter. The angular velocity of P about A and C are in the ratio :

A $1 : 1$
B $1 : 2$
C $2 : 1$
D $4 : 1$
Question 3 - Select One
A particle is moving in a circle with uniform speed its motion is
Anot periodic
Bperiodic and simple harmonic
Cperiodic but not simple harmonic
Dnone of the above

NIKITA PUBLICATION-CIRCULAR MOTION-Multiple Choice Question

Home
Ask Doubt
Profile