Three masses, each equal to M, are placed at the three corners of a square of side a. the force of attraction on unit mass at the fourth corner will be
Gm3a2
Gm√3a2
3Gma2
Gma2(√2+1/2)
The correct Answer is:d
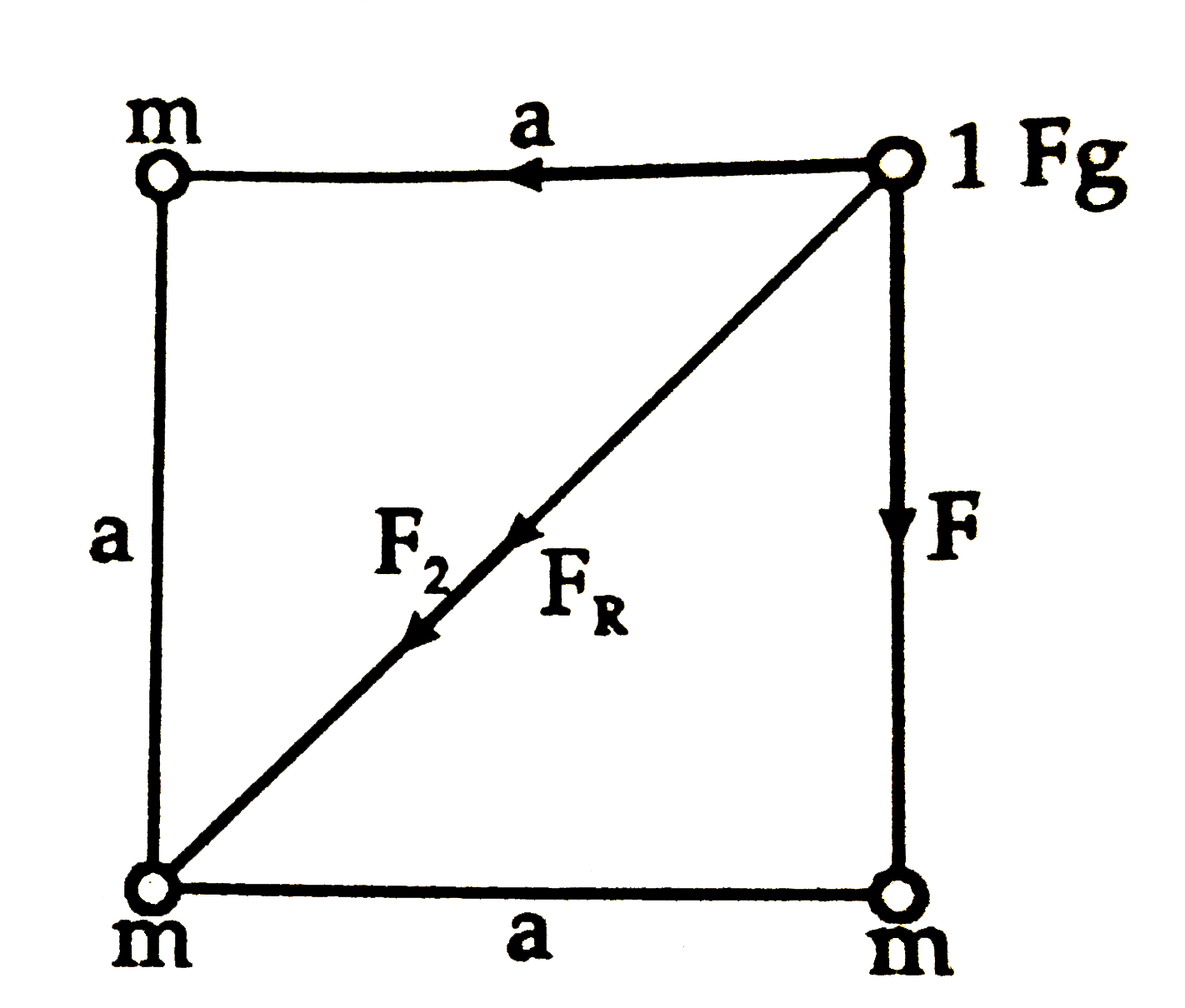
F=Gm×1a2=Gma2
FR=√F2+F2=√2F
FR=√2Gma2
FR and F2 are in the same direction then,
FR1=FR+F2
=√2Gma2+Gm2a2=Gma2[√2+12]
FR1=Gma2[2√2+12]
Topper's Solved these Questions
Similar Questions
Four masses, each equal to M are placed at the four corners of a square of side a. Calculate the force of attraction on another mass m, kept at the centre of the square.
View SolutionThree charges, each equal to q, are placed at the three. corners of a square of side a . Find the electric field at. the fourth corner.
View Solution
Knowledge Check
- Question 1 - Select One
Equal charges q are placed at the three corners B, C, D of a square ABCD of side a. The potential at A is
A14πε0qaB3q4πε0Cq4πε0a[2+1√2]Dq4πε0a[1+√2] - Question 2 - Select One
Equal charges q are placed at the three corners B, C, D of a square ABCD of side 'a'. The ratio of potential at A to centre O is
A2√2+13√2B2+√26C2√2+16D2√2+13 - Question 3 - Select One
Three equal masses each of mass 'm' are palced at the three corners of an equilateral of side a
If a fourth particle of equal mass is placed at the centre of triangle then net force acting on it is equal to .AGm2a2B4Gm23a2C3Gm2a2Dzero
If four different masses m1,m2,m3 and m4 are placed at the four corners of a square of side a, the resultant gravitational force on a mass m kept at the centre is
View SolutionThree identical particles each of mass 0.1kg are arranged at three corners of a square of side √2m. The distance of the centre of mass from the fourth corners is
View SolutionThree point masses each of mass m are placed at the corners of an equilateral triangle of side 'a'. Then the moment of inertia of this system about an axis passing along one side of the triangle is
View SolutionThree point masses, each of mass m, are placed at the corners of an equilateral triangle of side L. The moment of inertia of this system about an axis along one side of the triangle is
View SolutionFour point masses, each of value m, are placed at the corners of a square ABCD of side 1. The moment of inertia of this system about an axis through A and parallel to BD is
View Solution

