If the radius of the earth shrinks by 1.5% (mass remaining same), then the value of acceleration due to gravity changes by
1%
2%
3%
4%
The correct Answer is:c
g=GMR2
∴ Δgg=−2ΔRR=−2×1.5=−3%
Topper's Solved these Questions
Similar Questions
Knowledge Check
The value of acceleration due to gravity of earth :
Ais the same on equator and polesBis the least on polesCis the least on equatorDincrease from pole to equatorThe value of acceleration due to gravity of the earth is
A98m/s2B9.8m/s2C0.98m/s2D0.098m/s2The value of acceleration due to gravity on earth depends on
Amass of the bodyBradius of the bodyCshape of the bodyDmass of the earth
If the radius of the earth were to shrink by 1% its mass remaining the same, the acceleration due to gravity on the earth's surface would
View SolutionIf the radius of the earth where to shrink by 1% with its mass remaining the same, the acceleration due to gravity on the earth's surface would :
View SolutionIf the radius of the earth where to shrink by 1% with its mass remaining the same, the acceleration due to gravity on the earth's surface would :
View SolutionThe Earth is not a perfect sphere. Due to the flattening of Earth at the poles, the radius of Earth is minimum at the poles and hence the value of g is maximum at the poles. One the other hand, the radius of the Earth is maximum at the equator of the Earth. As the mass and radius of moon are smaller than that of the Earth, so the value of g on moon is 1.63m/s2 . As we go up from the surface of the Earth, the distance from the centre of the Earth increases and hence the value of g decreases. The value of g decreases as we go down inside the Earth and it become zero at the centre of the Earth .
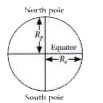
If the radius of the Earth were to shrink by 1% and its mass remaining the same, the acceleration due to gravity on the Earth's surface would
View SolutionIf the radius of the Earth shrinks by 2%, mass remaing same, then how would the have of acceleration due to gravity change?
View Solution