If ∫ex−1(x2−5x+4)2xdx=AF(x−1)+BF(x−4)+c and F(x)=∫exxdx, then-Solution in Bengali
A=−23
B=43e3
A=23
B=83e3
The correct Answer is:A,D
Step by step video, text & image solution for If int(e^(x-1))/((x^(2)-5x+4))2xdx=AF(x-1)+BF(x-4)+c and F(x)=int (e^(x))/(x)dx, then- by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- INTEGRATION BY PARTS BOOK - CHHAYA PUBLICATIONCHAPTER - INTEGRATION BY PARTS EXERCISE - Integer Answer Type
5 Videos
- INTEGRATION BY PARTS BOOK - CHHAYA PUBLICATIONCHAPTER - INTEGRATION BY PARTS EXERCISE - Matrix Match Type
2 Videos
- INTEGRATION BY PARTS BOOK - CHHAYA PUBLICATIONCHAPTER - INTEGRATION BY PARTS EXERCISE - Long Answer Type Questions
47 Videos
- INTEGRALS OF SOME SPECIAL FORM OF FUNCTIONSBOOK - CHHAYA PUBLICATIONCHAPTER - INTEGRALS OF SOME SPECIAL FORM OF FUNCTIONSEXERCISE - Comprehension Type
6 Videos
- INTRODUCTION TO THREE-DIMENSIONAL COORDINATE GEOMETRY BOOK - CHHAYA PUBLICATIONCHAPTER - INTRODUCTION TO THREE-DIMENSIONAL COORDINATE GEOMETRY EXERCISE - Sample questions for Competitive Exams ( D Comprehension Type)
4 Videos
Similar Questions
If ∫x2cos2xdx=x3k+18(2x2sin2x+2xcos2x−sin2x)+c, then the value of k is-
View SolutionMatch the following Column I and Column II
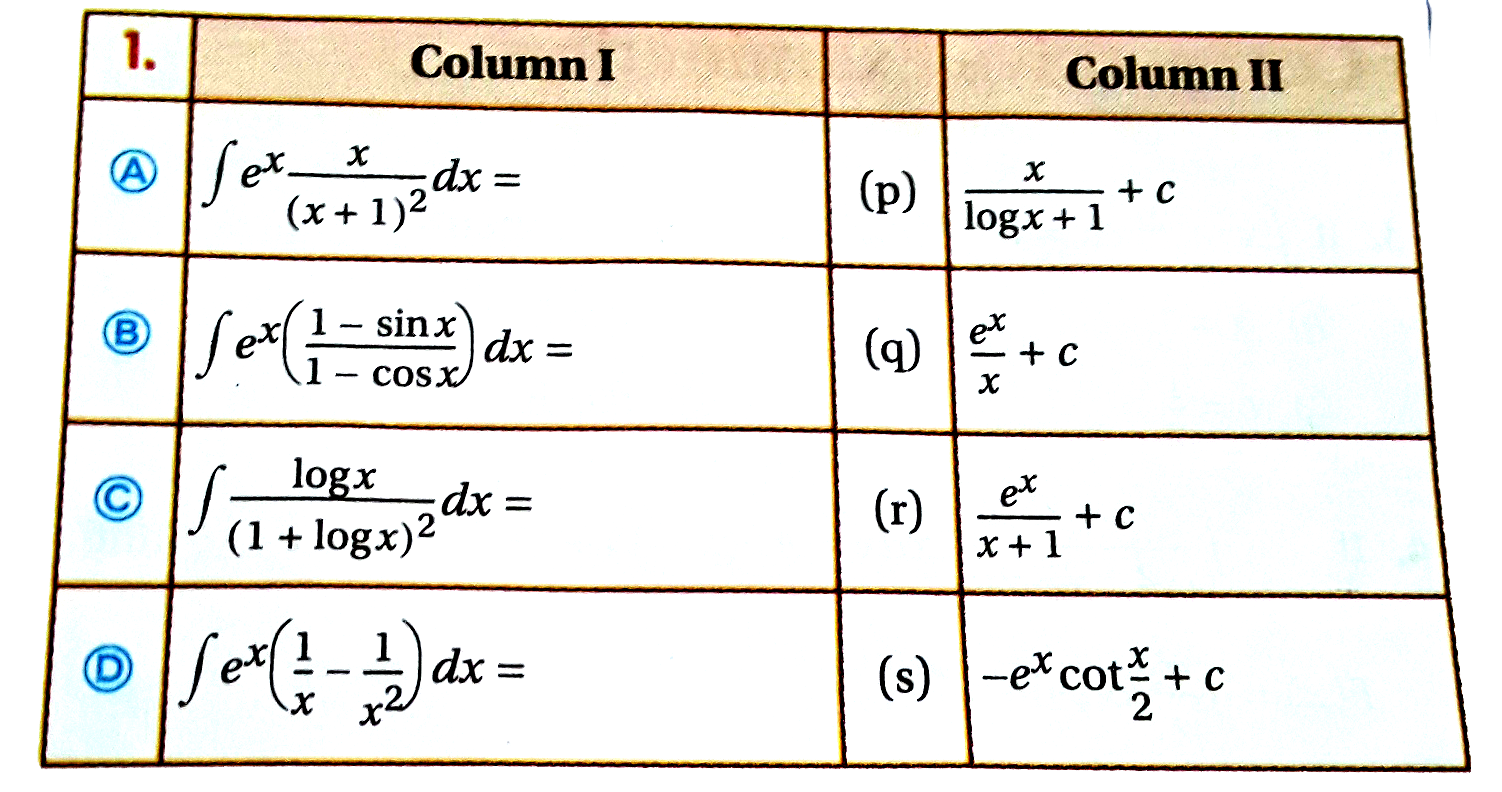
View Solution∫x2sin−1x(1−x2)52dx
View Solution∫dx(x−3)√x
View SolutionThe point ( 2,3,4) lies on octant
View SolutionIf ∫ex−1(x2−5x+4)2xdx=AF(x−1)+BF(x−4)+CandF(x)=∫exxdx,then (a)A=−23 (b) b=(43)e3 (c)A=23 (d) d∈R
View SolutionSTATEMENT-1 : ∫dxex+e−x+2=−1ex+1+c
and
STATEMENT-2 : ∫d(f(x))(f(x))2=−1f(x)+c
View SolutionIf ∫f'(x)ex2dx=(x−1)ex2+c , then f (x) is
View SolutionIf ∫x3e5xdx−e5x54(f(x))+03 then f(x)=
View SolutionA: ∫ex(1+xlogxx)=exlogx+c
R: ∫ex[f(x)+f'(x)]dx=exf(x)+c
View SolutionIf ∫x3e5xdx=e5x54(f(x))+c, then f(x)=
View SolutionIf ∫f'(x)ex2dx=(x−1)ex2+c then f (x) is . . . . .
View SolutionI = int e^(x) { f (x) + f' (x) } dx can be found using segmented integration. As I = int e^(x) f (x) + dx + int e^(x) f' (x) dx = int e^(x) dx + e^(x) f (x) - int e^(x) f (x) dx + c = e^(x) f (x) + c And int e^(ax) { f (x) + (f' (x))/(a) }dx = a^(ax) (f(x))/(a) + c int e^(x) (tan^(-1) x + (1)/(1 + x^(2)) ) dx = :
View SolutionI = int e^(x) { f (x) + f' (x) } dx can be found using segmented integration. As I = int e^(x) f (x) + dx + int e^(x) f' (x) dx = int e^(x) dx + e^(x) f (x) - int e^(x) f (x) dx + c = e^(x) f (x) + c And int e^(ax) { f (x) + (f' (x))/(a) }dx = a^(ax) (f(x))/(a) + c int e^(x) (tan x - log cos x ) dx = : int e^(x) ((1 + sin x)/(1 + cos x )) dx = :
View SolutionIf ∫ex(x2−5x+8) dx = ex f(x) + c then f(x) =
View Solution
