Repeated application of intergration by parts gives us, the reduction formula if the integrand is dependent on a natural number n.
If ∫cosmxsinnxdx=cosm−1x(1−n)sinn−1x+A∫cosm−2xsinn−2xdx+c, then A is equal to-Solution in Bengali
mm+n
m−1m+n
mm+n−1
m−1m−n
The correct Answer is:D
Step by step video, text & image solution for Repeated application of intergration by parts gives us, the reduction formula if the integrand is dependent on a natural number n. If int(cos^(m)x)/(sin^(n)x)dx=(cos^(m-1)x)/((1-n)sin^(n-1)x)+A int (cos^(m-2)x)/(sin^(n-2)x)dx+c, then A is equal to- by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- INTEGRATION BY PARTS BOOK - CHHAYA PUBLICATIONCHAPTER - INTEGRATION BY PARTS EXERCISE - Assertion-Reason Type
2 Videos
- INTEGRATION BY PARTS BOOK - CHHAYA PUBLICATIONCHAPTER - INTEGRATION BY PARTS EXERCISE - Matrix Match Type
2 Videos
- INTEGRALS OF SOME SPECIAL FORM OF FUNCTIONSBOOK - CHHAYA PUBLICATIONCHAPTER - INTEGRALS OF SOME SPECIAL FORM OF FUNCTIONSEXERCISE - Comprehension Type
6 Videos
- INTRODUCTION TO THREE-DIMENSIONAL COORDINATE GEOMETRY BOOK - CHHAYA PUBLICATIONCHAPTER - INTRODUCTION TO THREE-DIMENSIONAL COORDINATE GEOMETRY EXERCISE - Sample questions for Competitive Exams ( D Comprehension Type)
4 Videos
Similar Questions
Statement-I: ∫exsinxdx=ex2(sinx−cosx)+c
Statement-II: ∫ex(f(x)+f'(x))dx=exf(x)+c
View SolutionMatch the following Column I and Column II
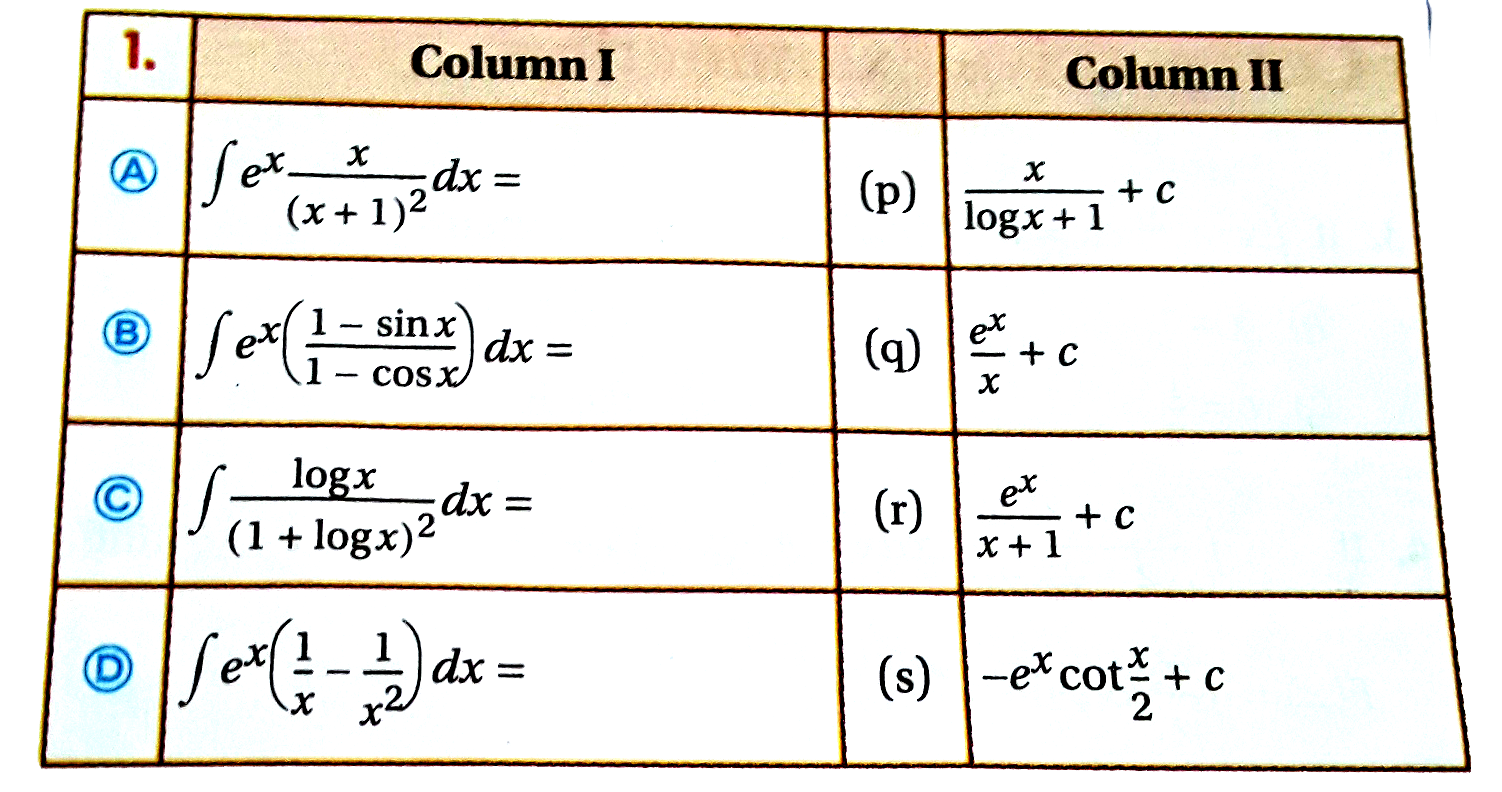
View Solution∫dx(x−3)√x
View SolutionThe point ( 2,3,4) lies on octant
View SolutionIf m,n∈N, then
∫π/20(sinmx)1n(sinmx)1n+(cosmx)1ndx is equal to
View SolutionProve :
∫sinmxsinnxdx[m2≠n2] ,
=12[sin(m−n)xm−n−sin(m+n)xm+n]+c
View Solution∫dxx√ax+b
View Solution∫cos3xsinxdx
View SolutionDerive reduction formula for
l(n,m)=∫sinnxcosmxdx.
View SolutionIf Im,n=∫cosmx⋅cosnxdx, show that (m+n)Im,n=cosmx⋅sinnx+mI(m−1,n−1)
View SolutionLet Im,n=∫sinnxcosmxdx. Then , we can relate In,m with each of the following :
(i) In−2,m (ii)In+2,m
(iii) In,m−2 (iv)In,m+2
(v) In−2,m+2 In+2,m−2
Suppose we want to establish a relation between In,m and In,m−2, then we get
P(x)=sinn+1xcosm−1x ...(i)
In In,m and In,m−2 the exponent of cos x in m and m−2 respectively, the minimum of the two is m - 2, adding 1 to the minimum we get m−2+1=m−1. Now, choose the exponent of sin x for m - 1 of cos x in P(x). Similarly, choose the exponent of sin x for
P(x)=(nH)sinnxcosmx−(m−1)sinn+2xcosm−2x.
Now, differentiating both the sides of Eq. (i), we get
=(n+1)sinnxcosmx−(m−1)sinnx(1−cos2x)cosm−2x
=(n+1)sinnxcosmx−(m−1)sinnxcosm−2x+(m−1)sinnxcosnx
=(n+m)sinnxcosmx−(m−1)sinnxcosm−2x
Now, integrating both the sides, we get
sinn+1xcosm−1x=(n+m)In,m−(m−1)In,m−2
Similarly, we can establish the other relations.
The relation between I4,2 and I2,2 is
View SolutionLet Im,n=∫sinnxcosmxdx. Then , we can relate In,m with each of the following :
(i) In−2,m (ii)In+2,m
(iii) In,m−2 (iv)In,m+2
(v) In−2,m+2 In+2,m−2
Suppose we want to establish a relation between In,m and In,m−2, then we get
P(x)=sinn+1xcosm−1x ...(i)
In In,m and In,m−2 the exponent of cos x in m and m−2 respectively, the minimum of the two is m - 2, adding 1 to the minimum we get m−2+1=m−1. Now, choose the exponent of sin x for m - 1 of cos x in P(x). Similarly, choose the exponent of sin x for
P(x)=(nH)sinnxcosmx−(m−1)sinn+2xcosm−2x.
Now, differentiating both the sides of Eq. (i), we get
=(n+1)sinnxcosmx−(m−1)sinnx(1−cos2x)cosm−2x
=(n+1)sinnxcosmx−(m−1)sinnxcosm−2x+(m−1)sinnxcosnx
=(n+m)sinnxcosmx−(m−1)sinnxcosm−2x
Now, integrating both the sides, we get
sinn+1xcosm−1x=(n+m)In,m−(m−1)In,m−2
Similarly, we can establish the other relations.
The relation between I4,2 and I6,2 is
View SolutionLet Im,n=∫sinnxcosmxdx. Then , we can relate In,m with each of the following :
(i) In−2,m (ii)In+2,m
(iii) In,m−2 (iv)In,m+2
(v) In−2,m+2 In+2,m−2
Suppose we want to establish a relation between In,m and In,m−2, then we get
P(x)=sinn+1xcosm−1x ...(i)
In In,m and In,m−2 the exponent of cos x in m and m−2 respectively, the minimum of the two is m - 2, adding 1 to the minimum we get m−2+1=m−1. Now, choose the exponent of sin x for m - 1 of cos x in P(x). Similarly, choose the exponent of sin x for
P(x)=(nH)sinnxcosmx−(m−1)sinn+2xcosm−2x.
Now, differentiating both the sides of Eq. (i), we get
=(n+1)sinnxcosmx−(m−1)sinnx(1−cos2x)cosm−2x
=(n+1)sinnxcosmx−(m−1)sinnxcosm−2x+(m−1)sinnxcosnx
=(n+m)sinnxcosmx−(m−1)sinnxcosm−2x
Now, integrating both the sides, we get
sinn+1xcosm−1x=(n+m)In,m−(m−1)In,m−2
Similarly, we can establish the other relations.
The relation I4,2 and I4,4 is
View SolutionDerive reduction formula for
l(n,m)=∫sinnxcosmxdx.
View SolutionIf Im,n=∫cosmx⋅cosnxdx, show that (m+n)Im,n=cosmx⋅sinnx+mI(m−1,n−1)
View Solution
