Three capacitors 2μF,3μF and 5μF can withstand voltages to 3V,2V and 1V respectively. Their series combination can withstand a maximum voltage equal to
5 Volts
(31/6) Volts
(26/5) Volts
None
The correct Answer is:B
To find the maximum voltage that the series combination of the three capacitors can withstand, we need to analyze the individual capacitors and their voltage ratings.
1. Identify the Capacitors and Their Ratings:
- Capacitor C1: 2μF with a maximum voltage of 3V
- Capacitor C2: 3μF with a maximum voltage of 2V
- Capacitor C3: 5μF with a maximum voltage of 1V
2. Determine the Charge on Each Capacitor:
- The charge Q on each capacitor in series is the same. The maximum charge that each capacitor can hold can be calculated using the formula:
Q=C×V
- For C1:
Q1=C1×Vmax1=2μF×3V=6μC
- For C2:
Q2=C2×Vmax2=3μF×2V=6μC
- For C3:
Q3=C3×Vmax3=5μF×1V=5μC
3. Identify the Limiting Factor:
- In a series circuit, the charge Q must be the same across all capacitors. The capacitor with the smallest charge capacity limits the total charge in the circuit.
- Here, C3 can only handle 5μC, which is the smallest charge capacity.
4. Calculate the Maximum Voltage Across Each Capacitor:
- Now we can calculate the voltage across each capacitor when Q=5μC:
- For C1:
V1=QC1=5μC2μF=2.5V
- For C2:
V2=QC2=5μC3μF≈1.67V
- For C3:
V3=QC3=5μC5μF=1V
5. Calculate the Total Voltage in Series:
- The total voltage Vtotal across the series combination is the sum of the voltages across each capacitor:
Vtotal=V1+V2+V3=2.5V+1.67V+1V≈5.17V
6. Conclusion:
- The maximum voltage that the series combination of the capacitors can withstand is approximately 5.17V.
- Capacitor C1: 2μF with a maximum voltage of 3V
- Capacitor C2: 3μF with a maximum voltage of 2V
- Capacitor C3: 5μF with a maximum voltage of 1V
- The charge Q on each capacitor in series is the same. The maximum charge that each capacitor can hold can be calculated using the formula:
Q=C×V
- For C1:
Q1=C1×Vmax1=2μF×3V=6μC
- For C2:
Q2=C2×Vmax2=3μF×2V=6μC
- For C3:
Q3=C3×Vmax3=5μF×1V=5μC
- In a series circuit, the charge Q must be the same across all capacitors. The capacitor with the smallest charge capacity limits the total charge in the circuit.
- Here, C3 can only handle 5μC, which is the smallest charge capacity.
- Now we can calculate the voltage across each capacitor when Q=5μC:
- For C1:
V1=QC1=5μC2μF=2.5V
- For C2:
V2=QC2=5μC3μF≈1.67V
- For C3:
V3=QC3=5μC5μF=1V
- The total voltage Vtotal across the series combination is the sum of the voltages across each capacitor:
Vtotal=V1+V2+V3=2.5V+1.67V+1V≈5.17V
- The maximum voltage that the series combination of the capacitors can withstand is approximately 5.17V.
Similar Questions
Three capacitors of 2μF,3μFand5μF are independently charged with batteries of emf’s 5V, 20V and 10V respectively. After disconnecting from the voltage sources. These capacitors are connected as shown in figure with their positive polarity plates are connected to A and negative polarity is earthed. Now a battery of 20V and an uncharged capacitor of 4μF capacitance are connected to the junction A as shown with a switch S. When switch is closed, find :
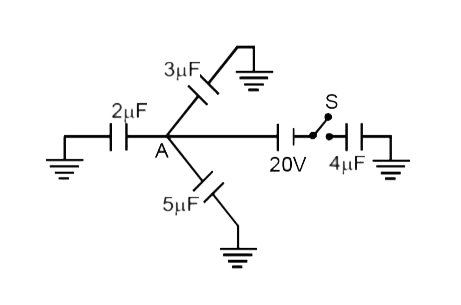 (a) the potential of the junction A. (b) final charges on all four capacitors.View Solution
(a) the potential of the junction A. (b) final charges on all four capacitors.View SolutionThree capacitors of 2μF,3μFand5μF are independently charged with batteries of emf’s 5V, 20V and 10V respectively. After disconnecting from the voltage sources. These capacitors are connected as shown in figure with their positive polarity plates are connected to A and negative polarity is earthed. Now a battery of 20V and an uncharged capacitor of 4μF capacitance are connected to the junction A as shown with a switch S. When switch is closed, find :
 (a) the potential of the junction A. (b) final charges on all four capacitors.View Solution
(a) the potential of the junction A. (b) final charges on all four capacitors.View Solution
Knowledge Check
- Question 1 - Select One
Three capacitors 2μF,3μFand5μFcan withstand voltages to 3 V, 2V and 1V respectively. Their series combination can withstand a maximum voltage equal to
A5 voltsB(31/6) VoltsC(26/5) VoltsDNone - Question 2 - Select One
A capacitor of capacitance C1=1μF withstand a maximum voltage of V1=6KV, and another capacitor of capacitance C2=2μF, can with stand a maximum voltage of V2=4KV. If they are connected in series, what maximum voltage will the system withstand?
A3kVB6kVC10kVD9kV - Question 3 - Select One
A capacitor of capacitance C1=1μF can withstand maximum voltage V1=6kV and another capacitor of capacitance C2=3μF can withstand maximum voltage V24kV. When the two capacitors are connected in series, the combined system can withstand a maximum voltage of
A4kVB6kVC8kVD10kV
Three capcaitors of capacities 1μF,2μF and 3μF are charged by 10V,20V and 30V respectively. Now positive plates of first two capacitors are connected with the negative plate of third capacitor on one side and negative plates of first wo capacitors are connectes with positive of third capacitor on the other side. Find
a. common potential V
b. final charges on different capacitors.View SolutionA capacitor of capacitance C1=1μF withstand a maximum voltage of V1=6KV, and another capacitor of capacitance C2=2μF, can with stand a maximum voltage of V2=4KV. If they are connected in series, what maximum voltage will the system withstand?
View SolutionTwo capacitors 3μF and 4μF have same maximum voltage rating V0. When they are connected in series, they can withstand a maximum potential difference of 700V. What is V0 ?
View SolutionThree capacitors 2μF,3μFand6μF are joined with each other. What is the minimum effective capacitance?
View Solution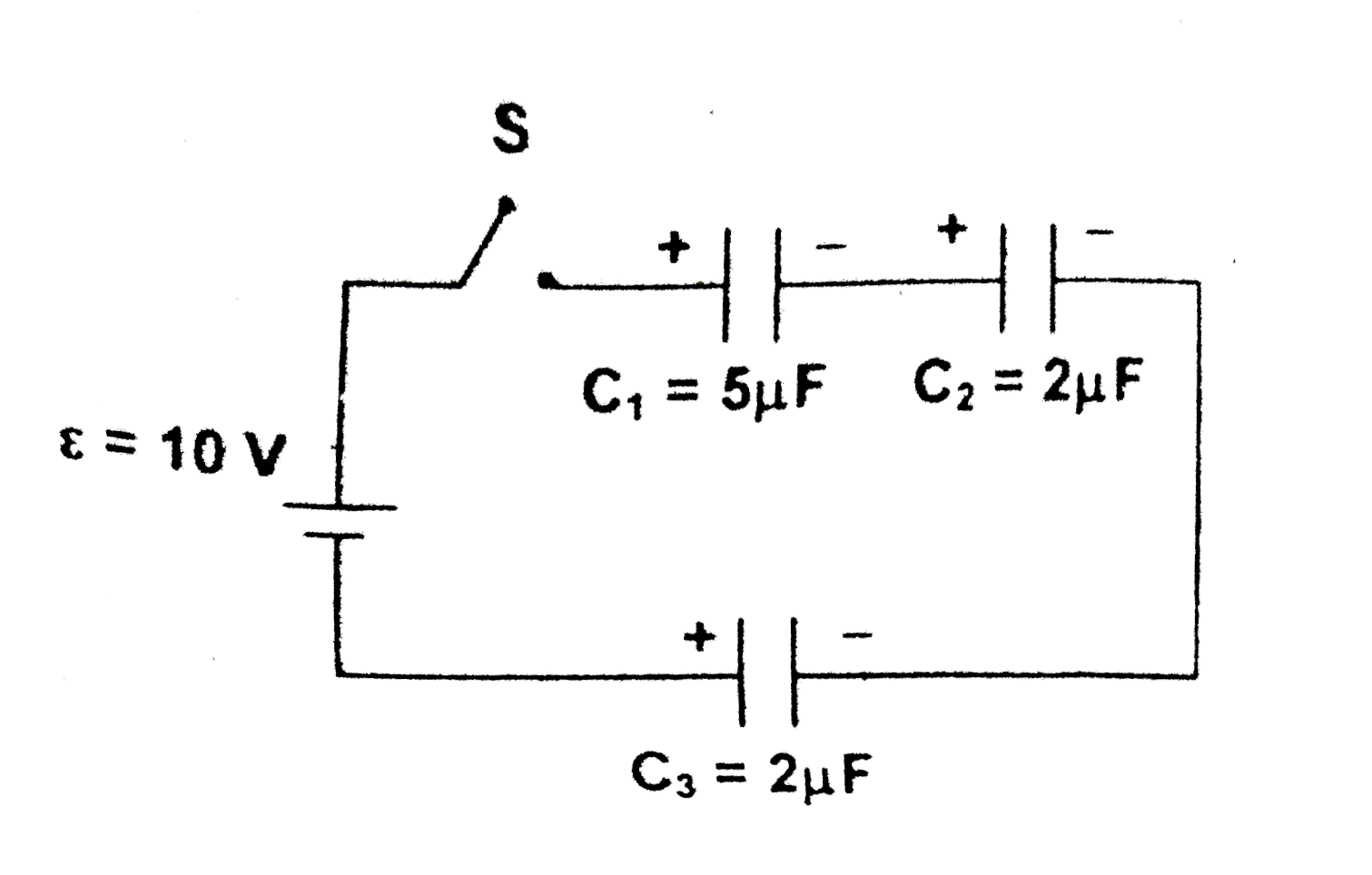
Three capacitors of capacitances 5μF,2μF and 2μF are charged to 20 V, 30 V and 10 V respectively and then connected in the circuit with polarities as shown. The magnitude of charge flown through the battery after closing the switch S isView Solution

