The value of the resistance R in figure is adjusted such that power dissipated in the 2Ω resistor is maximum. Under this condition
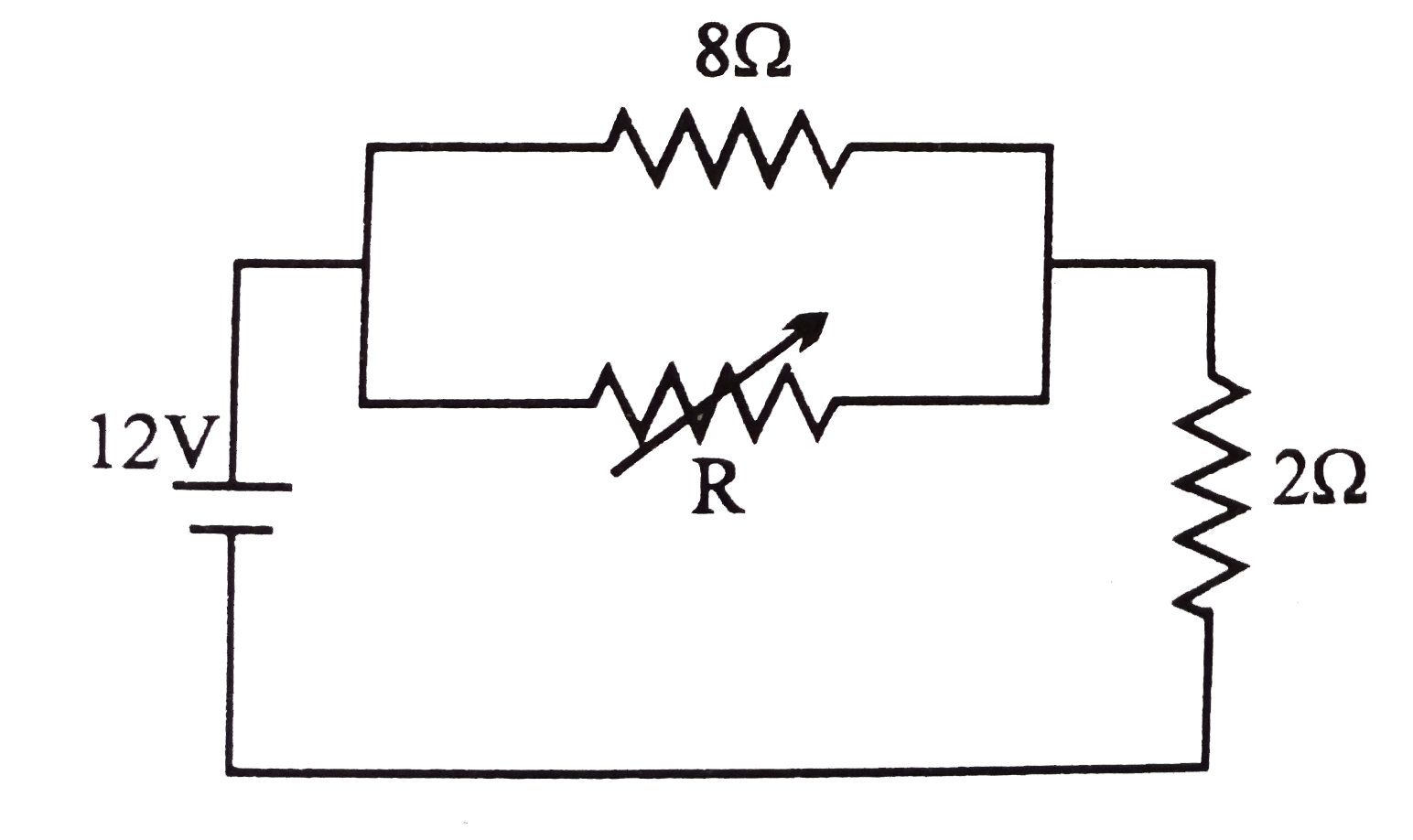
R=0
R=8Ω
power dissipated in the 2Ω resistor is 72W.
power dissipated in the 2Ω resistor is 8W.
The correct Answer is:A, C
Power will be maximum in 2Ω when current through it is maximum. It happens when R=0
Imax=6A
P2Ω=(6)2+2=72 watt
Similar Questions
Knowledge Check
- Question 1 - Select One
The power dissipated 6Ω resistor is
 A278WB916WC616WD316W
A278WB916WC616WD316W - Question 2 - Select One
A current of 3A flows through the 2Ω resistor shown in the circuit the power dissipated in the 5Ω resistor is
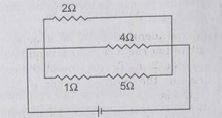 A4WB2WC1WD5W
A4WB2WC1WD5W - Question 3 - Select One
A current of 3A flows through the 2Ω resistor shown in the circuit the power dissipated in the 5Ω resistor is
 A5 watt.B4 watt.C2 watt.D1 watt
A5 watt.B4 watt.C2 watt.D1 watt
The power dissipated (in watt) in 3Ω resistance in the following circuit is
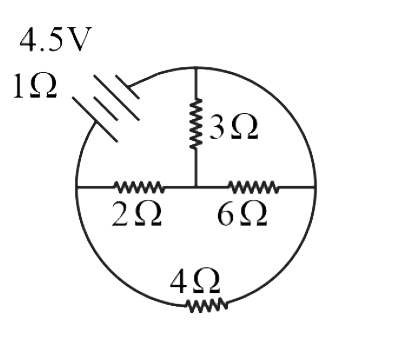 View Solution
View SolutionIn the circuit shown in the figure , the resistance marked R can be varied by using a Rheostat. The power across the 5 Omega resistor will be maximum if the Rhoestat has resistance equal to
_PHY_E01_015_Q01.png) View Solution
View SolutionFind the value of R in figure so that there is no current in the 50(Omega) resistor.
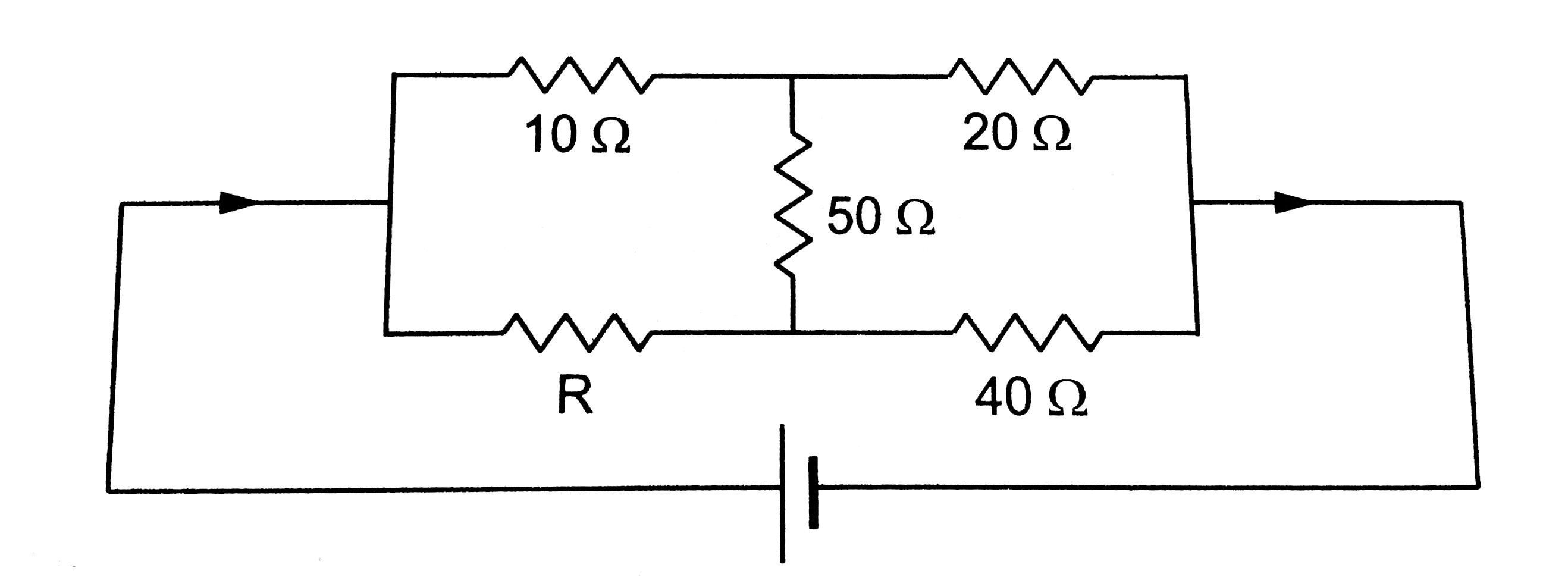 View Solution
View SolutionThree cells of emf 16V, 2V and 3V and internal resistance 2Ω,1Ω and 1/2Ω respectively are connected with an unknown resistance R as shown in the figure. What is the value of R such that power developed through R is maximum in steady state condition?
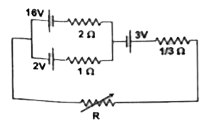 View Solution
View SolutionA current of 3 A flows through the 2Ω resistor shown in the circuit. The power dissipated in the 5 Ω resistor is
 View Solution
View Solution