Find time of flight if projectile thrown horizontally with speed 10ms−1 from a long inclined plane which makes an angle of θ=45∘ from horizontal.
√2sec
2√2sec
2sec
none
The correct Answer is:C
To find the time of flight of a projectile thrown horizontally from a long inclined plane at an angle of θ=45∘ with a speed of 10m/s, we can follow these steps:
Step 1: Understand the Problem
We have a projectile launched horizontally from an inclined plane. The angle of the incline is 45∘. The initial speed of the projectile is 10m/s.
Step 2: Resolve the Initial Velocity
Since the projectile is thrown horizontally, its initial vertical component of velocity (uy) is 0m/s. The horizontal component of velocity (ux) is 10m/s.
Step 3: Determine the Acceleration Components
The acceleration due to gravity (g) acts downward. We need to resolve this into components along the incline. The component of gravitational acceleration acting perpendicular to the incline is:
g_{\perpendicular} = g \cos(45^\circ) = \frac{g}{\sqrt{2}}
And the component acting parallel to the incline is:
g∥=gsin(45∘)=g√2
Step 4: Calculate Time of Flight
Since the projectile is thrown horizontally, we need to find the time it takes to fall vertically until it reaches the incline. The vertical motion can be described by:
s = u_y t + \frac{1}{2} g_{\perpendicular} t^2
Here, uy=0, so the equation simplifies to:
s = \frac{1}{2} g_{\perpendicular} t^2
The distance s that the projectile falls vertically until it hits the incline can be expressed in terms of the angle of the incline. For a 45∘ incline, the vertical distance fallen is equal to the horizontal distance traveled.
Step 5: Set Up the Relationship
Since the incline is at 45∘, the horizontal distance traveled x is equal to the vertical distance fallen y:
x=y
Using the relationship from the projectile motion:
x=uxt
And for the vertical distance:
y = \frac{1}{2} g_{\perpendicular} t^2
Setting x=y:
u_x t = \frac{1}{2} g_{\perpendicular} t^2
Step 6: Solve for Time of Flight
Substituting ux=10m/s and g_{\perpendicular} = \frac{g}{\sqrt{2}} :
10t=12(g√2)t2
10t=gt22√2
Rearranging gives:
t=20√2g
Substituting g≈9.81m/s2:
t≈20√29.81≈2.87s
Final Answer
The time of flight is approximately 2.87s.
---
We have a projectile launched horizontally from an inclined plane. The angle of the incline is 45∘. The initial speed of the projectile is 10m/s.
Since the projectile is thrown horizontally, its initial vertical component of velocity (uy) is 0m/s. The horizontal component of velocity (ux) is 10m/s.
The acceleration due to gravity (g) acts downward. We need to resolve this into components along the incline. The component of gravitational acceleration acting perpendicular to the incline is:
g_{\perpendicular} = g \cos(45^\circ) = \frac{g}{\sqrt{2}}
And the component acting parallel to the incline is:
g∥=gsin(45∘)=g√2
Since the projectile is thrown horizontally, we need to find the time it takes to fall vertically until it reaches the incline. The vertical motion can be described by:
s = u_y t + \frac{1}{2} g_{\perpendicular} t^2
Here, uy=0, so the equation simplifies to:
s = \frac{1}{2} g_{\perpendicular} t^2
The distance s that the projectile falls vertically until it hits the incline can be expressed in terms of the angle of the incline. For a 45∘ incline, the vertical distance fallen is equal to the horizontal distance traveled.
Since the incline is at 45∘, the horizontal distance traveled x is equal to the vertical distance fallen y:
x=y
Using the relationship from the projectile motion:
x=uxt
And for the vertical distance:
y = \frac{1}{2} g_{\perpendicular} t^2
Setting x=y:
u_x t = \frac{1}{2} g_{\perpendicular} t^2
Substituting ux=10m/s and g_{\perpendicular} = \frac{g}{\sqrt{2}} :
10t=12(g√2)t2
10t=gt22√2
Rearranging gives:
t=20√2g
Substituting g≈9.81m/s2:
t≈20√29.81≈2.87s
The time of flight is approximately 2.87s.
Similar Questions
A projectile si thrown with velocity of 50m/s towards an inclined plane from ground such that is strikes the inclined plane perpendiclularly. The angle of projection of the projectile is 53∘ with the horizontal and the inclined plane is inclined at an angle of 45∘ to the horizonta..

(a) Find the time of flight.
(b) Find the distance between the point of projection and the foot of inclined plane.View SolutionA projectile is thrown horizontally with a speed of 20ms−1 .If g is (10ms−2) ,then the speed of the projectile after 5 second will be nearly
View Solution
Knowledge Check
- Question 1 - Select One
A particle is projected horizontally with a speed V=5m/s from the top of a plane inclined at an angle θ=37∘ to the horizontal (g=10m/s2)
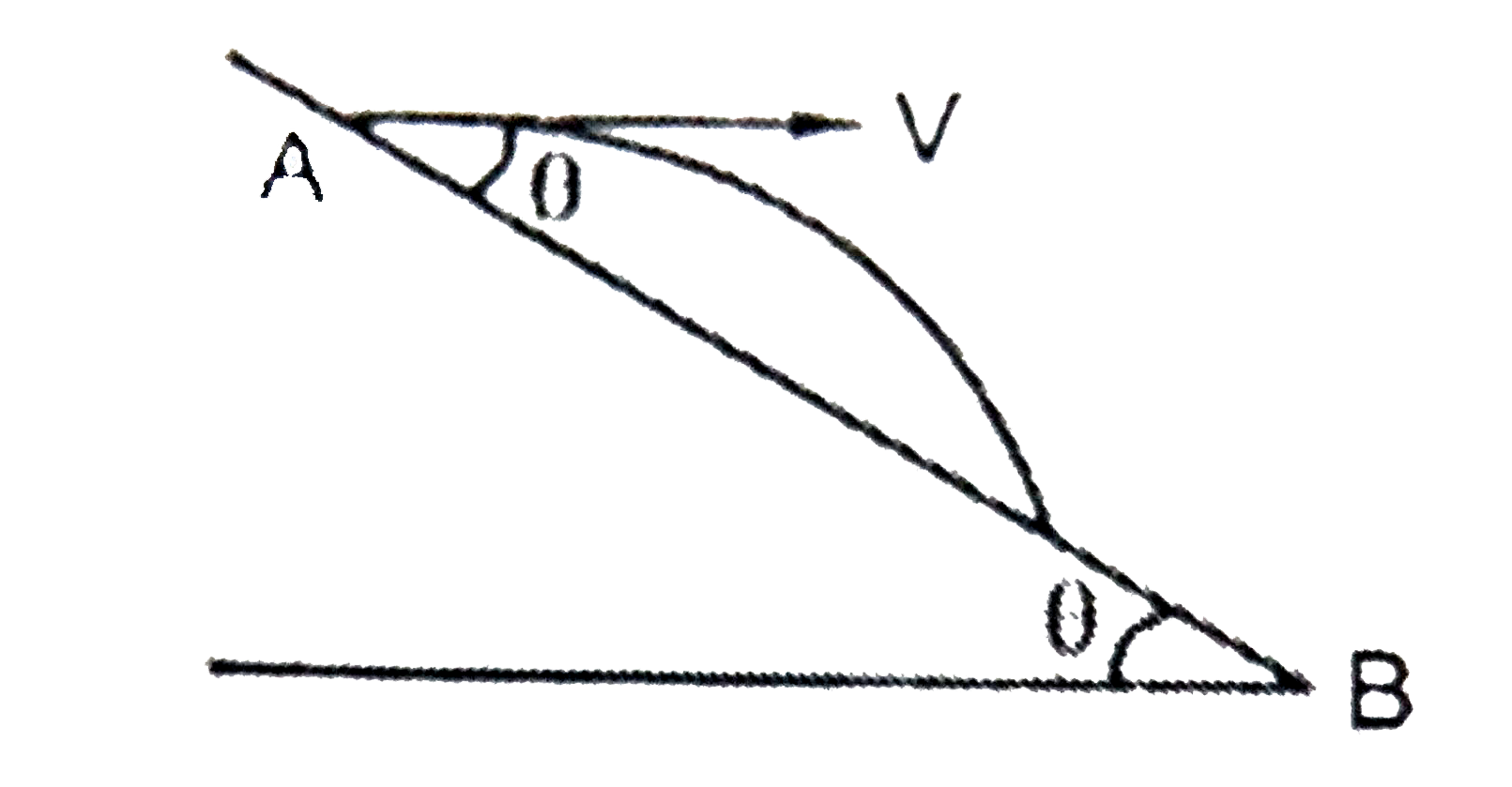
Find the time taken by the particle to hit the plane.A34sB3sC4sD43s - Question 2 - Select One
A particle is projected from horizontal ground with speed 5ms−1 at 53∘ with horizontal. Find time after which velocity of particle will be 45∘ with horizontal:
A110 secB310 secC510 secD710 sec - Question 3 - Select One
A particle is projected horizontally with a speed V=5m/s from the top of a plane inclined at an angle θ=37∘ to the horizontal (g=10m/s2)
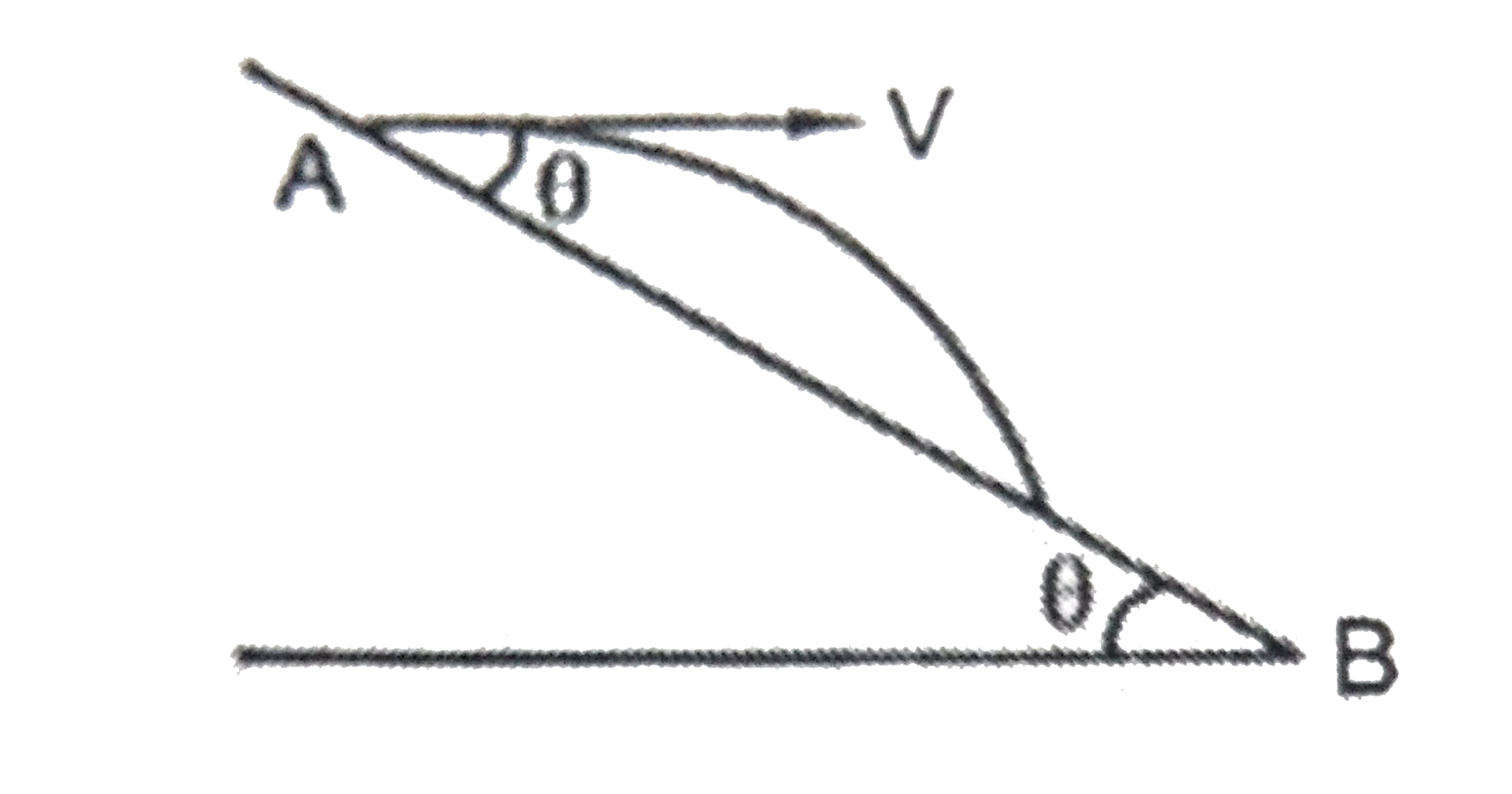
What is the velocity of the particle just before it hits the plane?A5√3m/sB52√13m/sC10√13m/sD5√26m/s
A particle is projected horizontally with a speed u from the top of plane inclined at an angle θ with the horizontal. How far from the point of projection will the particle strike the plane ?
 View Solution
View SolutionA body is thrown horizontally with an initial velocity of 10m/s from a cliff. At what time (in sec.) will the velocity vector make an angle of 45∘ with horizontal?
View SolutionA particle is thrown horizontally from the top of an inclined plane , with a speed of 5m/s . If the inclination of the plane is 30∘ with the horizontal , how far from the point of projection will the particle strike the plane ?
View SolutionA ball is projected from a point on an inclined plane horizontally. It takes 1√2 seconds to strike the plane. If the incline plane makes an angle of 30∘ with horizontal then find the range of the ball on incline plane in meters[g=10m/s2]
View SolutionA particle is projected from horizontal making an angle of 53∘ with initial velocity 100ms−1. The time taken by the particle to make angle 45∘ from horizontal is
View Solution

