A solid sphere with a velocity (of centre of mass) v and angular velocity ω is gently placed on a rough horizontal surface. The frictional force on the sphere :
must be forward (in direction of v)
must be backward (opposite to v)
cannot be zero
none of the above
The correct Answer is:D
Case I
(i) If Rω>v than point of contact slides backward and friction direction.
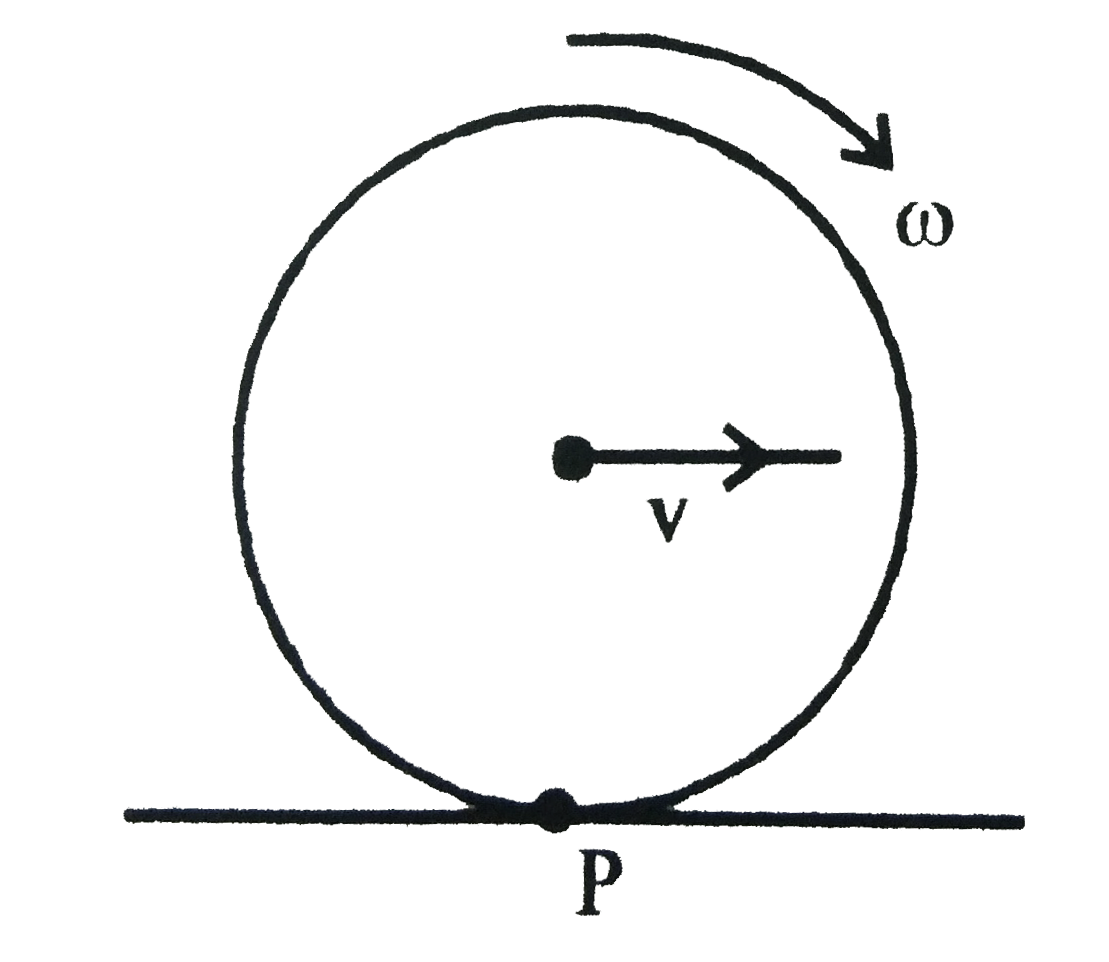
vP=v−Rω
(ii) If v>Rω than point contact slides forward and friction will act in backward direction
vP=v−Rω
(iii) If v=Rω,f=0,vP=0
Case II
so friction willm act in backward direction.
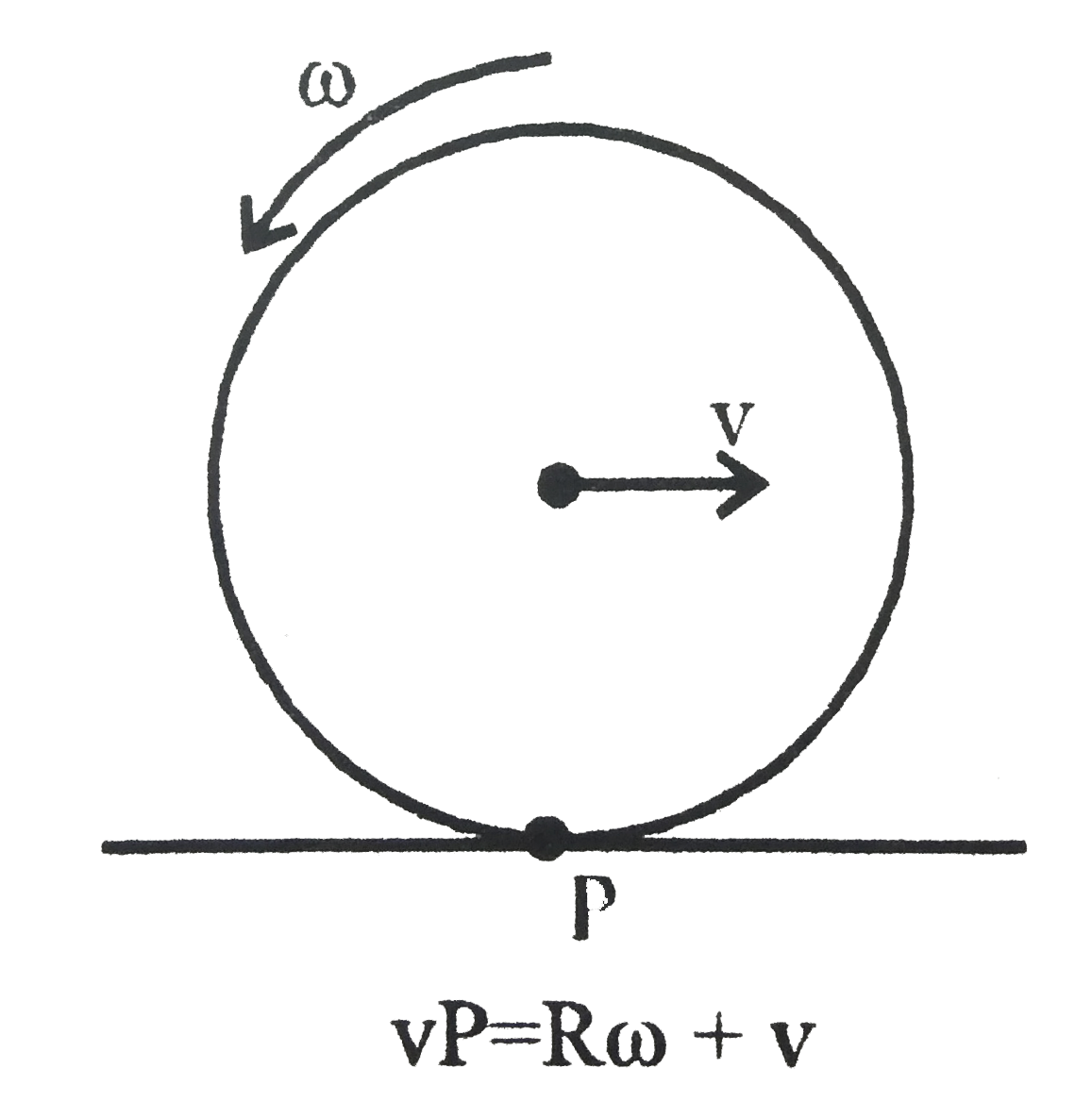 .
.
Similar Questions
A solid cylinder is rolling without slipping with velocity of its centre of mass v and angular velocity about its centre of mass ω on a horizontal frictionless surface as shown in figure. If it collides with a frictionless vertical wall X, then after collision its velocity and angular velocity respectively become
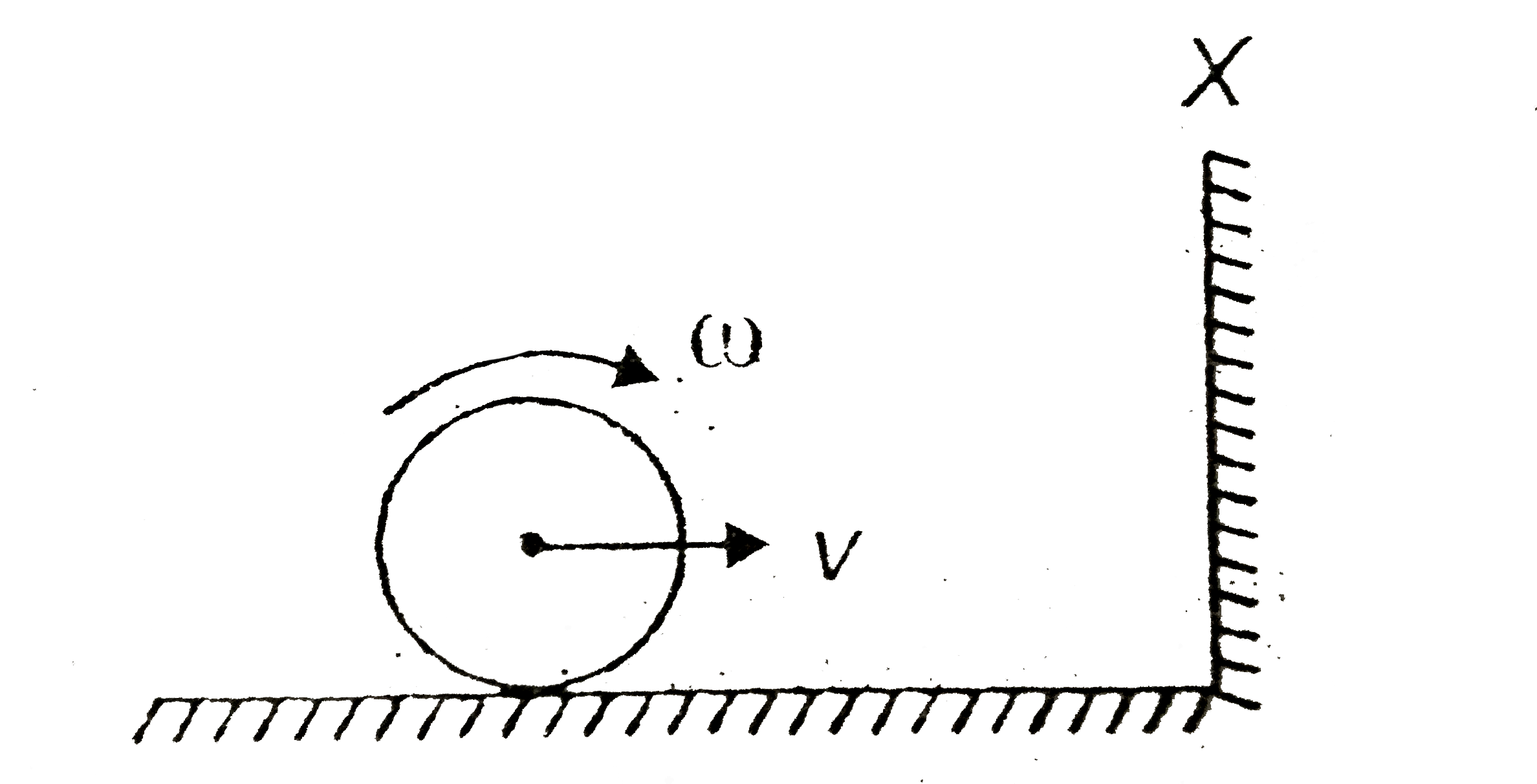 View Solution
View SolutionA solid sphere spinning about a horizontal axis with an angular velocity ω is placed on a horizontal surface. Subsequently it rolls without slipping with an angular velocity of :
View Solution
Knowledge Check
- Question 1 - Select One
A ring of radius R is first rotated with an angular velocity ω and then carefully placed on a rough horizontal surface. The coefficient of friction between the surface and the ring is μ. Time after which its angular speed is reduced to half is
Aω0μR2gBω0g2μRC2ω0RμgDω0R2μg - Question 2 - Select One
A hoop of radius r and mass m rotating with an angular velocity ω0 is placed on a rough horizontal surface. The initial velocity of the centre of the hoop is zero. What will be the velocity of the centre of the hoop when it ceases ot slip?
Arω04Brω03Crω02Drω0 - Question 3 - Select One
A hoop of radius r mass m rotating with an angular velocity ω0 is placed on a rough horizontal surface. The initial velocity of the centre of the hoop is zero. What will be the velocity of the centre of the hoop when it cases to slip ?
Arω02Brω0Crω04Drω03
A solid sphere of mass m and radius R is moving with velocity of centre of massvˆi and angular velocity about centre ofmass ωˆi If the coefficient of friction between the sphere and ground μ the frictional force vectoris (consider v=ωR):
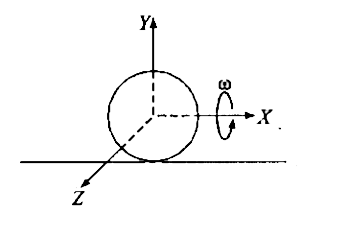 View Solution
View SolutionA solid sphere and a hollow sphere of equal mass and radius are placed over a rough horizontal surface after rotating it about its mass centre with same angular velocity ω0. Once the pure rolling starts let v1 and v2 be the linear speeds of their centres of mass. Then
View SolutionA disc is given an angular velocity ω0 and a linear velocity v0 as shown in the figure. It is released on a rough horizontal surface of friction coefficient μ. Mark the correct statement (ω0=3v0/R)
 View Solution
View SolutionA disc is given an initial angular velocity ω0 and placed on a rough horizontal surface as shown Fig. The quantities which will not depend on the coefficient of friction is/are
 View Solution
View SolutionConsider the system shown. A solide sphere (of mass m and radius R) is placed over a plak (of mass 2m and placed over a smooth horizontal surface.) A horizontal force F is applied at the top of the sphere, as shown. Consider that the friction between the sphere and plank is sufficient enough for no slipping of the sphere over then plank.
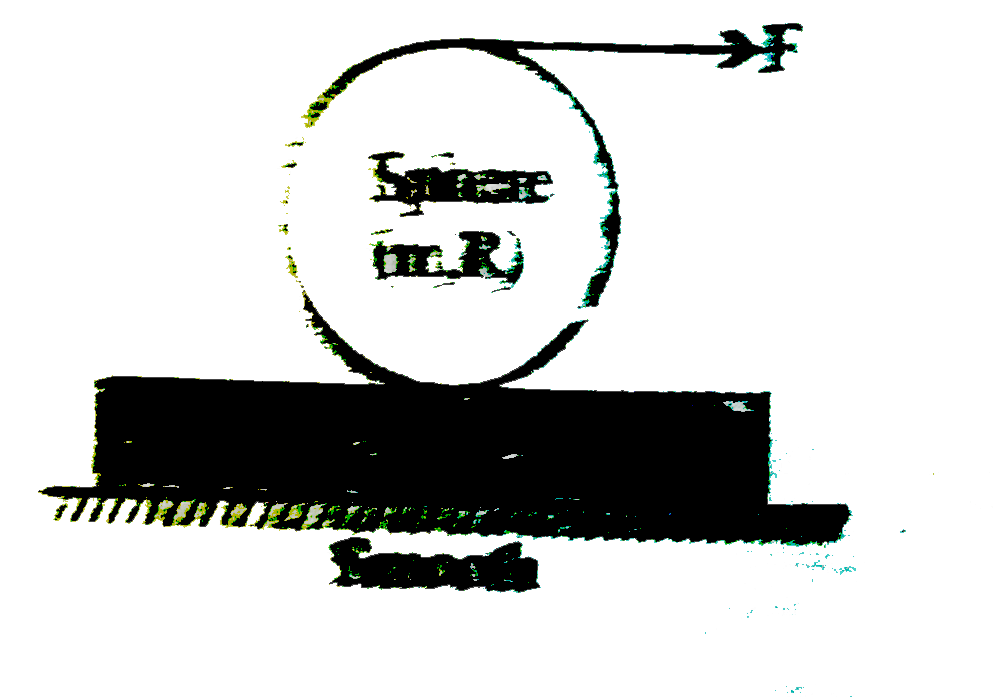
Acceleration of the plank isView Solution

