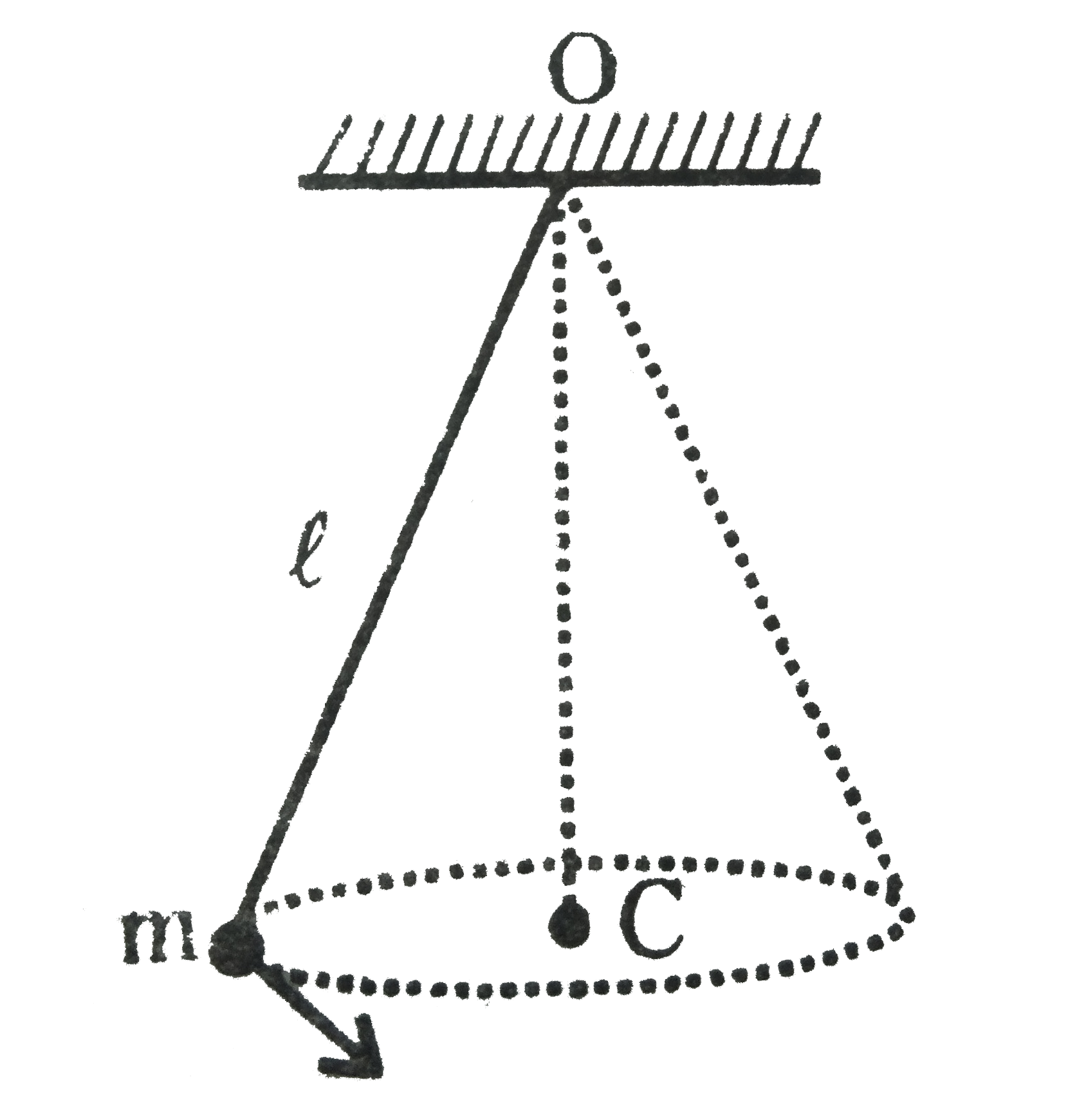
A small ball of mass m suspended from the celling at a point O by a thread of length l moves along a horizontal circle with a constant angular velocity ω.
 .
.
linear momentum of system (ball + rod) is conserved
angular momentum about C is conserved
kinetic energy of system (ball + rod) before the collision is equal to kinetic energy of system just after the collision
linear momentum of ball is conserved
The correct Answer is:B, C, D
Since ball moves with constant angular velocity inhorizontal circle than
(A) For angular momentum about A, it will have angular momentum perpendicular to l That will have two component one along CO which is constant and another in the plane which canges direction.
(B) Angular momentum about C is constant (mvr) along OC.
Similar Questions
A small ball of mass m suspended from the ceiling at a point O by a thread of length l moves along a horizontal circle with a constant angular velocity ω. Relative to which points does the angular momentum M of the ball remain constant? Find the magnitude of the increment of the vector of the ball's angular momentum relative to the point O picked up during half a revolution.
View SolutionFor a particle moving in a horizontal circle with constant angular velocity:
View Solution
Knowledge Check
A particle of mass m is suspended from the ceiling through a string of length L. The particle moves in a horizontal circle of radius r. The speed of the particle is
Arg√L2−r2Br√g(L2−r2)14Cr√g(L2−r2)12DmgL(L2−r2)12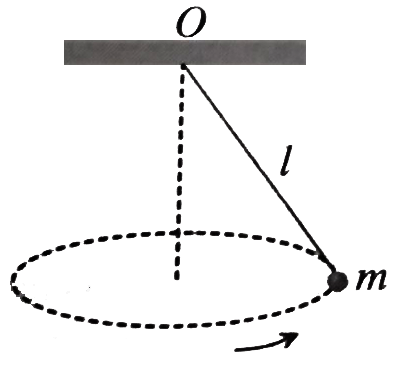
A point mass m is suspended from a light thread of length l, fixed at O, is whirled in a horizontal circle at constant speed as shown. From your point of view, stationary with respect to the mass, the forces on the mass areA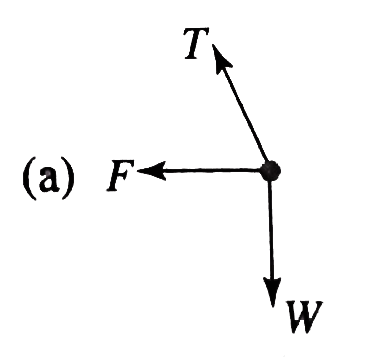 B
B C
C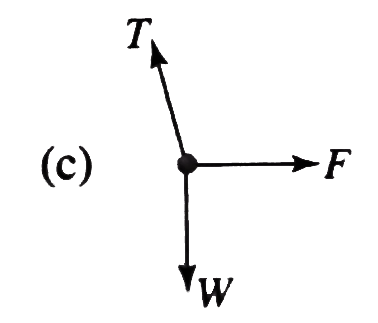 D
D
Two masses M and m are attached to a vertical axis by weightless threads of combined length l . They are set in rotational motion in a horizontal plane about this axis with constant angular velocity ω . If the tensions in the threads are the same during motion, the distance of M from the axis is
AMlM+mBmlM+mCM+mMlDM+mml
Consider a conical pendulum having bob is mass m is suspended from a ceiling through a string of length L. The bob moves in a horizontal circle of radius r. Find (a) the angular speed of the bob and (b) the tension in the string.
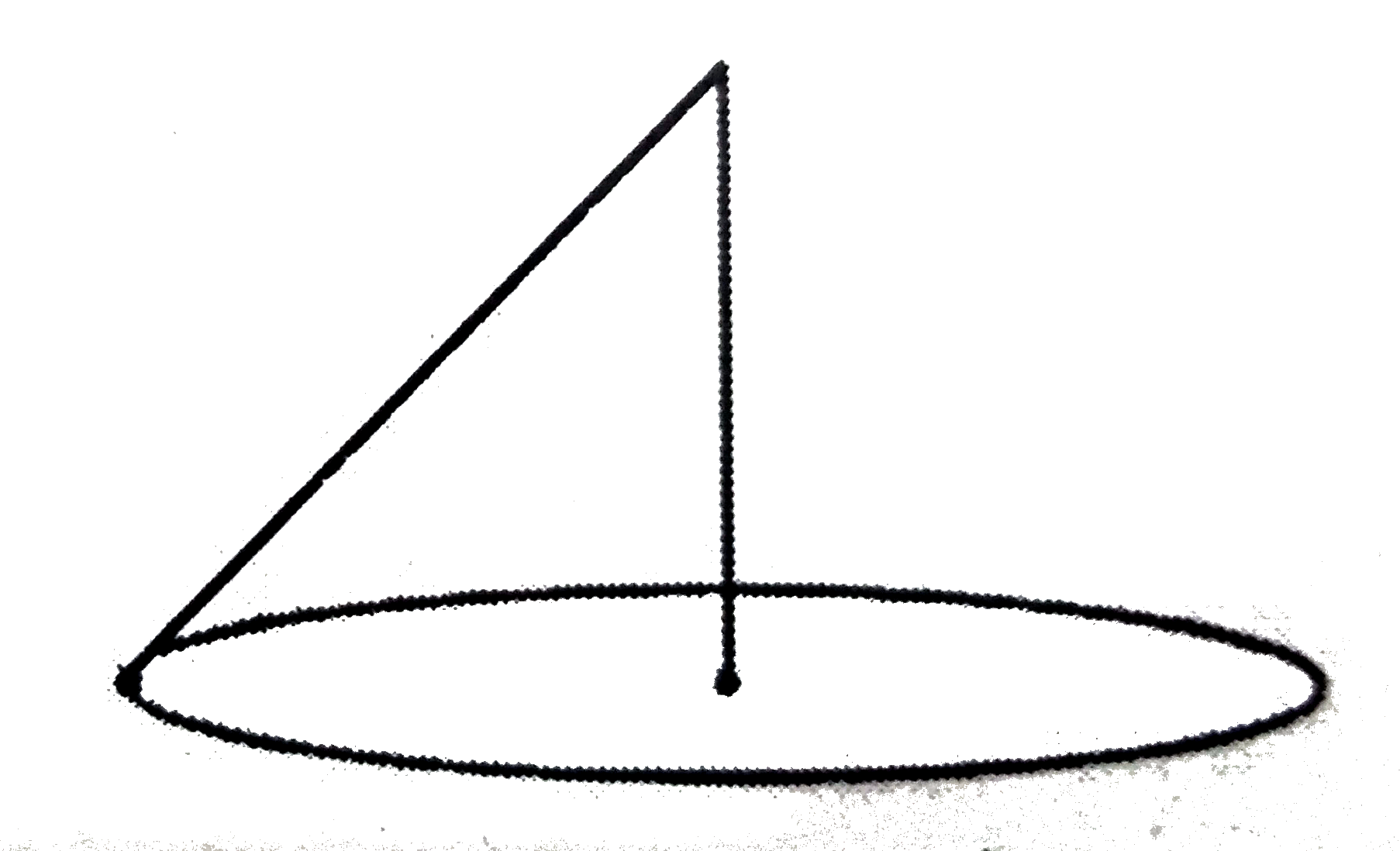
View Solution
A point mass m is suspended from a light thread of length l, fixed at O, is whirled in a horizontal circle at constant speed as shown. From your point of view, stationary with respect to the mass, the forces on the mass are
View Solution
A point mass m is suspended from a light thread of length l, fixed at O, is whirled in a horizontal circle at constant speed as shown. From your point of view, stationary with respect to the mass, the forces on the mass are
View SolutionA body of mass m s connected to an inextensible thread of length L si whirled in horizontal circle. Find the maximum angular velocity with which it can be whirsted without breaking the thread (Breaking stress of thread =S)
View SolutionA particle of mass m is attached to one end of a weightless and inextensible string of length L. The particle is on a smooth horizontal table. The string passes through a hole in the table and to its other and is attached a small particle of equal mass m. The system is set in motion with the first particle describing a circle on the table with constant angular velocity ω1 and the second particle moving in the horizontal circle as a conical pendulum with constant angular velocity ω2 Show that the length of the portions of the string on either side of the hole are in the ratio ω22:ω21
View Solution
