A thin prism of angle 5∘is placed at a distance of10cm from object.What is the distance of the image from object?Given μof prism=1.5).
π8cm
π12cm
5π36cm
π7cm
The correct Answer is:C
To solve the problem step by step, we will follow the principles of ray optics and the properties of a thin prism.
Step 1: Understand the setup
We have a thin prism with an angle of 5∘ placed at a distance of 10cm from the object. The refractive index (μ) of the prism is given as 1.5.
Step 2: Calculate the angle of deviation (δ)
For a thin prism, the angle of deviation can be calculated using the formula:
δ=(μ−1)×A
where A is the angle of the prism.
Substituting the values:
δ=(1.5−1)×5∘=0.5×5∘=2.5∘
Step 3: Convert the angle of deviation to radians
To convert degrees to radians, we use the conversion factor π180:
δ=2.5∘×π180=2.5π180=π72radians
Step 4: Use the small angle approximation
Since δ is a small angle, we can use the small angle approximation:
tan(δ)≈δ
Thus, we can write:
tan(δ)≈δ≈d10
where d is the distance between the object and the image.
Step 5: Set up the equation
From the small angle approximation:
d10≈π72
Step 6: Solve for d
Now, we can solve for d:
d≈10×π72=10π72=5π36
Step 7: Conclusion
Thus, the distance of the image from the object is:
d=5π36cm
Final Answer
The distance of the image from the object is 5π36cm.
---
We have a thin prism with an angle of 5∘ placed at a distance of 10cm from the object. The refractive index (μ) of the prism is given as 1.5.
For a thin prism, the angle of deviation can be calculated using the formula:
δ=(μ−1)×A
where A is the angle of the prism.
δ=(1.5−1)×5∘=0.5×5∘=2.5∘
To convert degrees to radians, we use the conversion factor π180:
δ=2.5∘×π180=2.5π180=π72radians
Since δ is a small angle, we can use the small angle approximation:
tan(δ)≈δ
Thus, we can write:
tan(δ)≈δ≈d10
where d is the distance between the object and the image.
From the small angle approximation:
d10≈π72
Now, we can solve for d:
d≈10×π72=10π72=5π36
Thus, the distance of the image from the object is:
d=5π36cm
The distance of the image from the object is 5π36cm.
Similar Questions
Knowledge Check
Two thin lenses of powers 2D and 4D are placed in close contact. An object is placed at a distance of 50 cm from the combination. What is the distance of the image from the combined lens ?
A30 cmB40 cmC25 cmD35 cmAn object is placed at a distance of 10 cm in front of a plane mirror, then the distance of image from mirror is:
A5 cmB10 cmC20 cmD0An object is placed at a distance of 10 cm from a convex lens of power 5 D. find the position of the image
A- 20 cmB30 cmC20 cmD- 30 cm
A lens is made of three thin different mediums. Radius of curvature and refractive index of each medium is shown iin Figure., Surface AB is straight. An object is placed at some distance from the lens by which a real image is formed on the screen placed at a distance of 10cm from the lens. Find
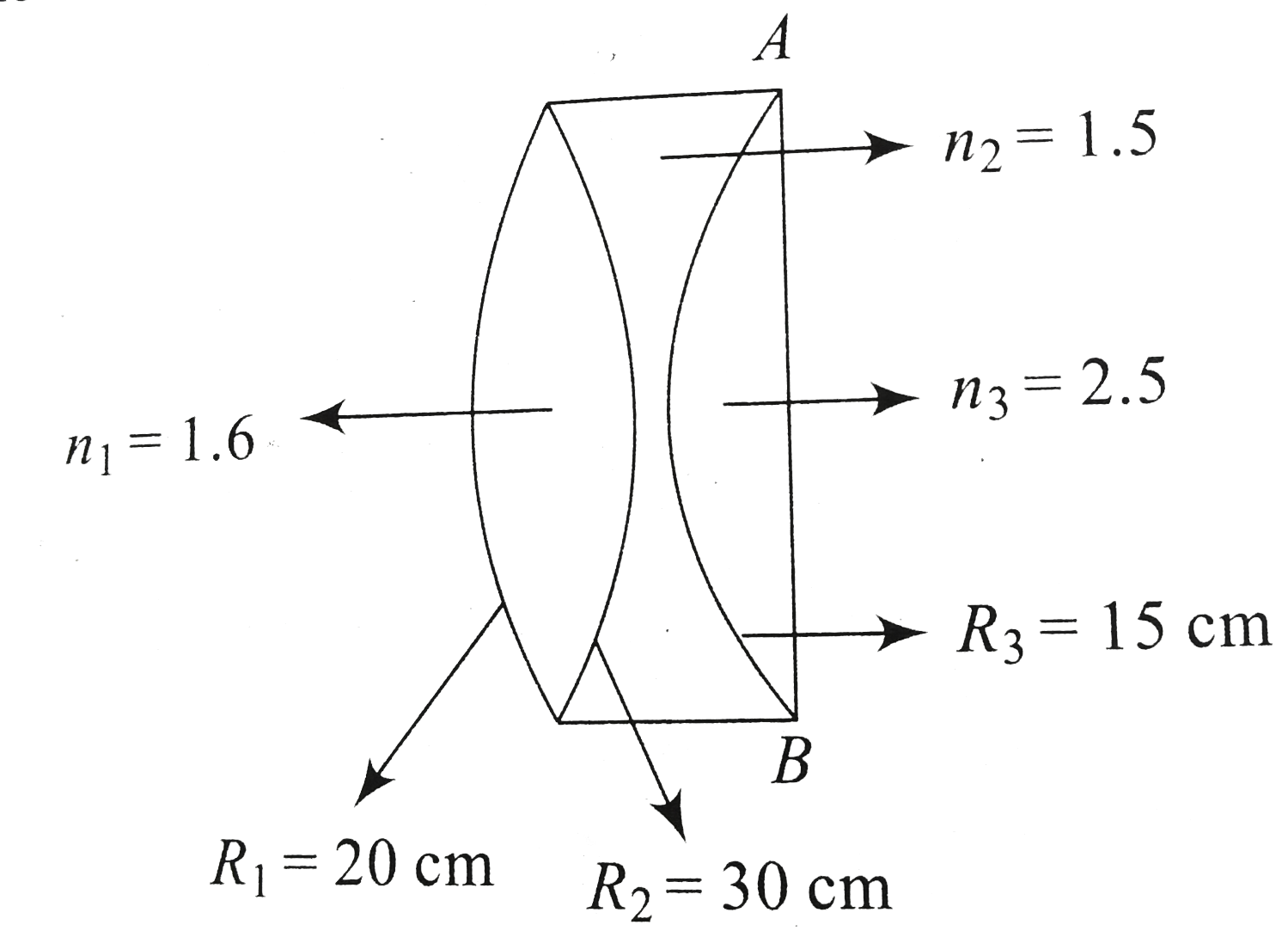
a. The distance of the object from the lens.
b. A slab of thiskness 1.5cm and refractive index 1.5 is introduced between the image and the lens. Find the new position of the object so that image is again formed on the screen.
c. Find the position of the image when same slabe is placed on the other side of the lens {as in part (b)}
View SolutionTwo thin lenses of powers 2D and 3D are placed in contact. An object is placed at a distance of 30 cm from the combination The distance in cm of the image from the combination is
View SolutionIf the object is placed at a distance of 10 cm from a plane mirror, then the image distance is ______ .
View SolutionAn object is placed at a distance of 20 cm from the pole of a concave mirror of focal length 10 cm. The distance of the image formed is
View SolutionIf the object is placed at a distance of 10 cm from a plane mirror, then the image distance is ______ .
View Solution