An inclinded plane making an angle of 30∘ with the horizontal electric field of 100Vm−1 as shown in Figure. A particle of mass 1kg and charge 0⋅01C is allowed to slide down from rest from a height of 1m. If the coefficient of friction is 0*2, find time taken by the particle to reach the bottom.
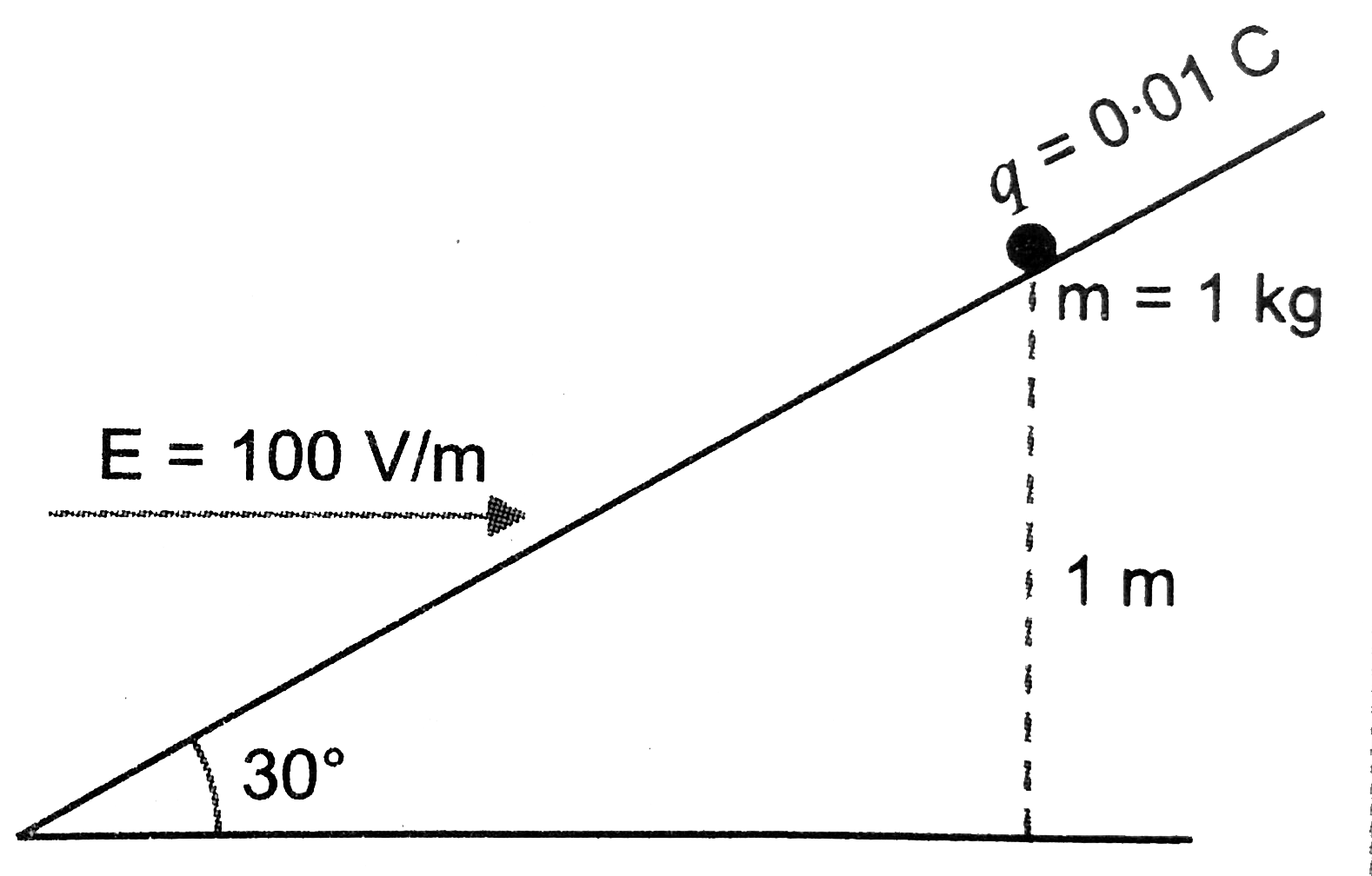
Here, θ=30∘,E=100Vm−1
m=1kg,q=0⋅01C
u=0,h=1m,μ=0⋅2
t=?
As is clear from Fig.
R=mgcos30∘+qEsin30∘
=1×9⋅8√32+0⋅01×100×12=8⋅9868
Net force on the charged particale down the incline

f=mgsin30∘−qEcos30∘−F(=μC)
=1×9⋅8×12−0⋅01×100×√32−0⋅2×8⋅9868
f=4⋅9−0⋅866−1⋅7936=2⋅2404
Acceleration of charged particle down the plane
a=fm=2⋅24041=2⋅2404m/s2
As sinθ=hl,l=hsinθ=1sin30∘=2m
From s=ut+12at2
l=0+12×2⋅2404t2
t2=2l2⋅2404=2×22⋅2404=1⋅785
t=√1⋅785=1⋅336s
Similar Questions
An inclined plane making an angle of 30∘ with the horizontal is placed in a uniform horizontal electric field 200 NC as shown in the figure. A body of mass 1kg and charge 5 mC is allowed to slide down from rest at a height of 1m. If the coefficient of friction is 0.2, find the time taken by the body to reach the bottom. [g=9.8m/s2,sin30∘=12,cos30∘=√32]
 View Solution
View SolutionA partical of mass 1kg rests on rought contact with a plane inclined at 30∘ to the horizontal and is just about to slip, Find the coefficient of friction between the plane and the particle.
View Solution
Knowledge Check
- Question 1 - Select One
An inclined plane of length 5.60 m making an angle of 45∘ with the horizontal is placed in a uniform electric field E=100Vm−1. A particle of mass 1kg and charge 10−2C is allowed to slide down from rest position from maximum height of slope. If the coefficient of friction is 0.1, then the time taken by the particle to reach the bottom is
A1 sB1.41 sC2 sDNone of these - Question 2 - Select One
A track has two inclined surface AB and DC each of length 3 m and angle of inclination of 30∘ with the horizontal and a central horizontal part of length 4m shown in figure. A block of mass 0.2 kg slides from rest from point A. The inclined surfaces are frictionless. If the coefficient of friction between the block and the horizontal flat surface is 0.2, where will the block finally come to rest ? [in 10−1m]
 A0.5 m away from point BB3.5 m away from point BC0.5 m away from point CD1.5 m away from point C
A0.5 m away from point BB3.5 m away from point BC0.5 m away from point CD1.5 m away from point C - Question 3 - Select One
When a body slides down from rest along a smooth inclined plane making an angle of 30∘ with the horizontal, it takes time 20s. When the same body slides down from rest along a rough inclined plane making the same angle and through the same distance, it takes time 20p is, where p is some number greater than 1. The coefficient of friction between the body and the rough plane is
Aμ=(1−1p2)1√3Bμ=(1−19p2)Cμ=(1−p2)1√3Dμ=√1−9p2
When a body slides down from rest along a smooth inclined plane making an angle of 30∘ with the horizontal, it takes time T. When the same body slides down from the rest along a rough inclined plane making the same angle and through the same distance, it takes time aT, where a is a constant greater than 1. The co-efficient of friction between the body and the rough plane is 1√x(a2−1a2) where x = ________.
View SolutionA particle slides down a smooth in clined plane of elevation θ fixed in an elevastor going up with an accelerationa0. The base of the incline has a length L.Find the time taken by the particle to reach the bottom.
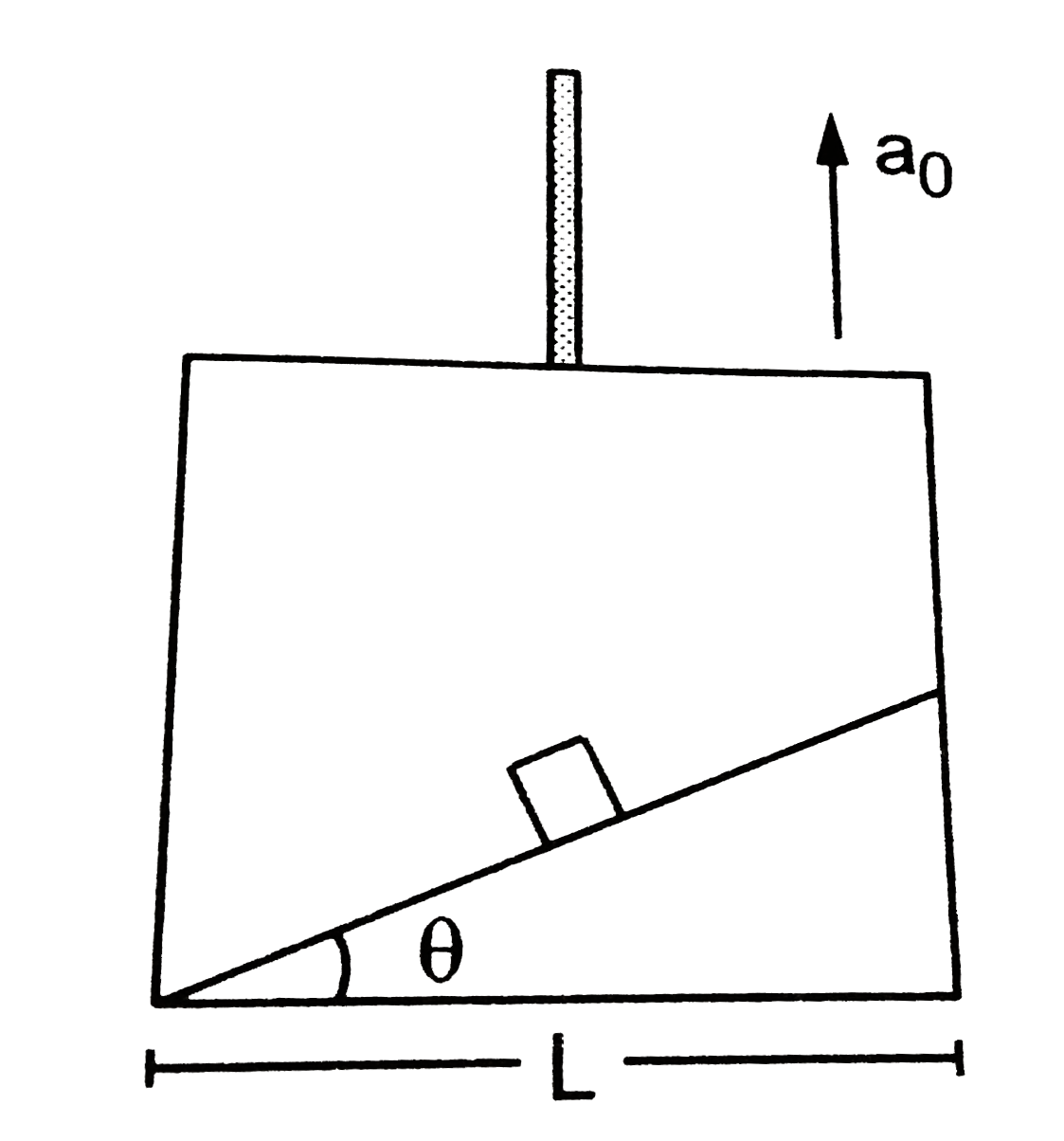 View Solution
View SolutionA particle is projected from horizontal making an angle of 53∘ with initial velocity 100ms−1. The time taken by the particle to make angle 45∘ from horizontal is
View SolutionA body is allowed to slide from the top along a smooth inclined plane of length 5m at an angle of inclination 30∘.If g=10ms−2, time taken by the body to reach the bottom of the plane is
View SolutionA particle of mass m is projected up from the bottom of an inclined plane with initial velocity v0 at angle 45∘ with an inclined plane of inclineation 30∘ as shown in figure. At the same time a small block of same mass m is released from rest at a height h. The particle hits the block at some point on inclined plane.
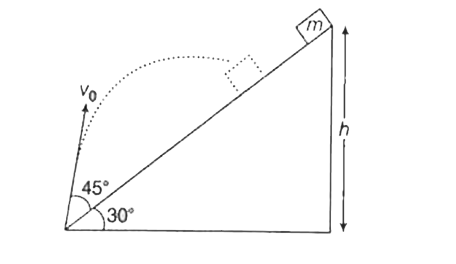
If the particle sticks to the block after collision, the velocity of block parallel to the inclined plane just after collision will be (take g=10m/s2)View Solution

