The electric field outside a charged long straight wire is given by E=1000rVm−1, and is directed outwards. What is the sign of the charge on the wire ? If two points A and B are situated such that rA=0.2m and rB=0.4m, find the value of (VB−VA).
The correct Answer is:+ charge ; −693.1V
To solve the problem step by step, we will first determine the sign of the charge on the wire and then calculate the potential difference VB−VA between points A and B.
Step 1: Determine the sign of the charge on the wire
Given that the electric field E outside the charged long straight wire is given by:
E=1000rV/m
and it is directed outwards. The direction of the electric field indicates the nature of the charge. If the electric field is directed outward from the wire, it means that the wire has a positive charge.
Conclusion for Step 1:
The charge on the wire is positive.
Step 2: Calculate the potential difference VB−VA
The potential difference between two points in an electric field can be calculated using the formula:
VB−VA=−∫rBrAEdr
Given that:
- rA=0.2m
- rB=0.4m
Substituting the expression for E:
VB−VA=−∫0.40.21000rdr
Step 3: Perform the integration
The integral of 1r is:
∫1rdr=lnr
Thus, we have:
VB−VA=−1000(lnr)0.40.2
Calculating the definite integral:
=−1000(ln(0.4)−ln(0.2))
Using the property of logarithms:
ln(0.4)−ln(0.2)=ln(0.40.2)=ln(2)
So we can rewrite the potential difference as:
VB−VA=−1000ln(2)
Step 4: Substitute the value of ln(2)
Using the approximate value ln(2)≈0.693:
VB−VA=−1000×0.693=−693V
Final Answer
Thus, the potential difference VB−VA is:
VB−VA=−693V
The charge on the wire is positive.
- rB=0.4m
Topper's Solved these Questions
- BOOK - PRADEEPCHAPTER - ELECTROSTATICSEXERCISE - FILL IN THE BLANKS8videos
ELECTROSTATICS
- BOOK - PRADEEPCHAPTER - ELECTROSTATICSEXERCISE - PROBLEMS FOR PRACTICE8videos
ELECTROSTATICS
- BOOK - PRADEEPCHAPTER - ELECTROSTATICSEXERCISE - VALUE BASED QUESTIONS7videos
ELECTROSTATICS
- BOOK - PRADEEPCHAPTER - ELECTRONIC DEVICESEXERCISE - Fill in the Blanks1videos
ELECTRONIC DEVICES
- BOOK - PRADEEPCHAPTER - MAGNETIC EFFECT OF CURRENT AND MAGNETISMEXERCISE - Competition Focus (Multiple Choice Questions)2videos
MAGNETIC EFFECT OF CURRENT AND MAGNETISM
Similar Questions
The electric field in a certain region is given by E=(5ˆi−3ˆj)kV/m. Find the difference in potential VB−VA. If A is at the origin and point B is at a. (0,0,5)m, b. (4,0,3) m.
View SolutionThe electric potential at 0.9m from a point charge is +50V. What is the magnitude and sign of the charge ?
View Solution
Knowledge Check
- Question 1 - Select One
The electric field in a region is radially outward and at a point is given by E=250 r V/m (where r is the distance of the point from origin). Calculate the charge contained in a sphere of radius 20 cm centred at the origin
A2.22×10−6CB2.22×10−6CC2.22×10−10CDZero - Question 2 - Select One
The electric potential V at any point ( x,y, z) in space is given by V=4x2V . The electric field E (in Vm) at the point ( 1,0,2) is
A+8 in x directionB8 in - x directionC16 in +x directionD16 in -x direction
The electric field in a region is radially outward with magnitude E = 2r. The charge contained in a sphere of radius a = 2m centerd at the origin is 4xπε0. Find the value of x.
View SolutionWhat is the excess charge on a conducting sphere of radius r = 0.35 m if the potential of the sphere is 1500 V and V = 0 at infinity ?
View SolutionThe electric foield at a point A on the perpendicular bisector of a uniformly charged wire of length l=3m and total charge q = 5 nC is x V/m. The distance of A from the centre of the wire is b = 2m. Find the value of x.
View SolutionCharges are placed on the vertices of a square as shown. Let E r be the electric field and V the potential at the centre. If the charges on A and B are interchanged with those on D and C respectively, then :-
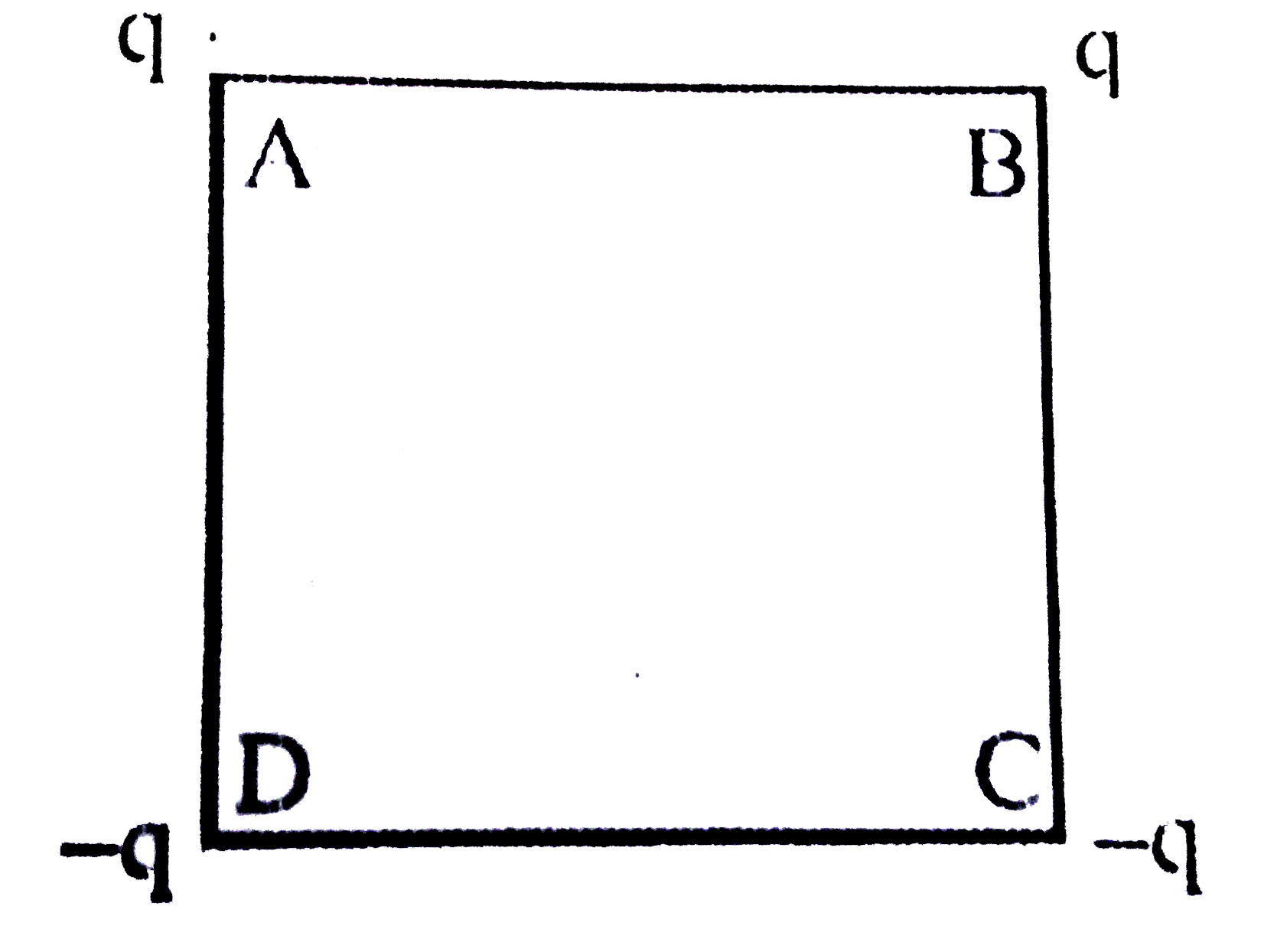 View Solution
View SolutionThe electric field in a region is radially outward and varies with distance r as E=250 r ( volt per square metre).Calculate the charge contained in a sphere of radius 0.2 m centeredat the origin.
View SolutionFind out electric field intensity at point A (0, 1m, 2m) due to a point charge −20μC situated at point B(√2m,0,1m).
View Solution

