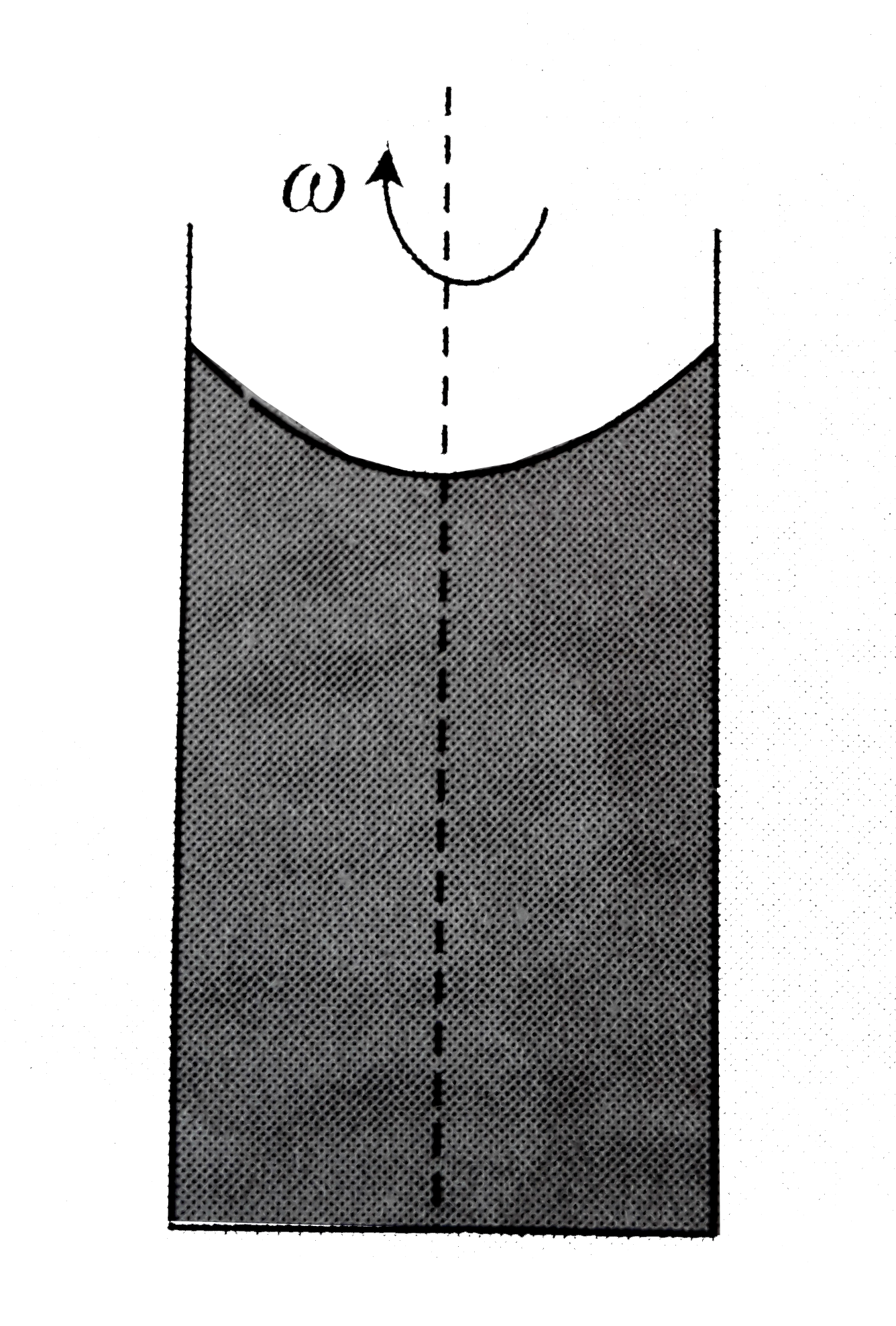
Find the mass of a mole of colloid particles if during their centrifuging with an angular velocity $ω$ about a vertical axis the concentration of the particles at the distance $r_{2}$ from the rotation axis is $η$ times greater than that at the distance $r_{1}$ (in the same horizontal plane). The densities of the particles and the solvent are equal to $ρ$ and to $ρ_{0}$ respectively.

Video Solution

Text Solution

Verified by Experts

In a centrifuge rotating with angular velocity $ω$ about an axis, there is a centrifugal acceleration $ω^{2} r$ where $r$ is the radial distance from the axis. In a fluid if there are suspened colloidal particle they experience an additional force. If $m$ is the mass of each particle then its volume is $\frac{m}{ρ}$ and the excess force on this particle is.
$\frac{m}{ρ} (ρ - ρ_{0}) ω^{2} r$ outward corresponding to a potential energy $- \frac{m}{2 ρ} (ρ - ρ_{0}) ω^{2} r^{2}$
This gives rise to a concentration variation.
$n (r) = n_{0} exp (+ \frac{m}{2 ρ k T} (ρ - ρ_{0}) ω^{2} r^{2})$
Thus $\frac{n (r_{2})}{n (r_{1})} = η = exp (+ \frac{M}{2 ρ R T} (ρ - ρ_{0}) ω^{2} (r_{2}^{2} - r_{1}^{2}))$
where $\frac{m}{k} = \frac{M}{R}, M = N_{A} m$ is the molecular weight
Thus $M = \frac{2 ρ R T 1 n η}{(ρ - ρ_{0}) ω^{2} (r_{2}^{2} - r_{1}^{2})}$ .

Updated on:21/07/2023