Two glass discs of radius R=5.0cm were wetted with water and put together so that the thickness of the water layer between them was h=1.9μm. Assuming the wetting to be complete. Find the force that has to be applied at right angles to the plates in order to pull them apart.
This is analogous to the previous prolem except that : A=πR2
So F=2πR2αh=0.6kN.
Topper's Solved these Questions
- THERMODYNAMICS AND MOLECULAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - THERMODYNAMICS AND MOLECULAR PHYSICSEXERCISE - Phase Transformations
35 Videos
- THERMODYNAMICS AND MOLECULAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - THERMODYNAMICS AND MOLECULAR PHYSICSEXERCISE - Transport Phenomena
38 Videos
- THERMODYNAMICS AND MOLECULAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - THERMODYNAMICS AND MOLECULAR PHYSICSEXERCISE - The Second Law Of Thermodynamics - Entropy
47 Videos
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Relativistic Mechanics
49 Videos
Similar Questions
Two identical smooth disc of radius R have been placed on a frictionless table touching each other. Another circular plate is placed between them as shown in figure. The mass per unit area of each object is σ, and the line joining the centers of the plate and the disc is θ
(a) Find the minimum horizontal force F0 that must be applied to the two discs to keep them together.
(b) Angle θ can be changed by changing the size of the circular plate. Find F0 when θ→0.
[use cosθ=1−θ22 and sinθ=θfor smallθ]
(c) Find F0 when θ→π2 . Explain the result .
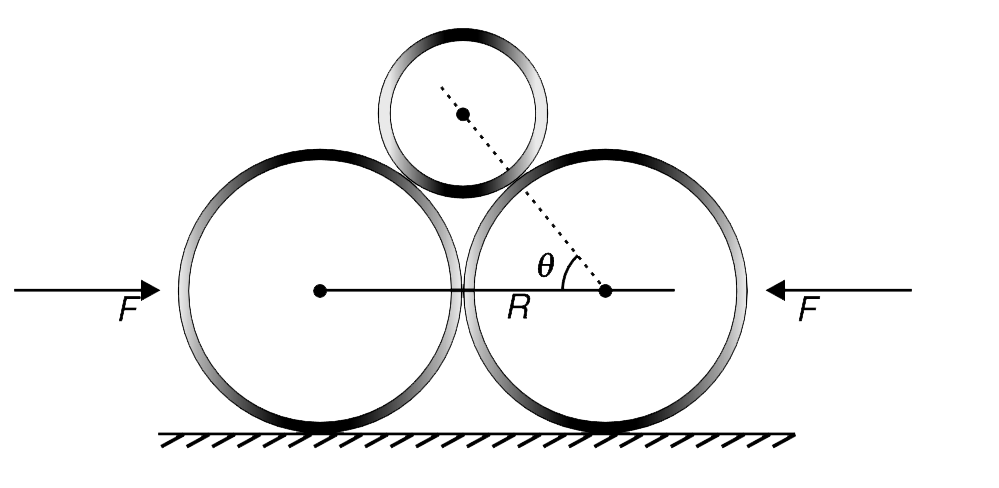
View SolutionTwo vertical, parallel glass plates are partially submerged in water. The distance between the plates is d=100μm, their length is l=10 cm. Assuming that the water between the plates does not reach the upper edge of the plates and that the wetting is complete, find the force of attraction, indicating how the force arises. Surface tension of water =73m N/m.
View Solution
Knowledge Check
Two vertical parallel glass plates are partically submerged in water. The distance between the plates is d=0.10mm, and their width is l=12cm assuming that the water between the plates does not reach the upper edges of the plates and the wetting is complete. Find the force of their mutual attraction
A13 NB26 NC39 ND6.5 NTwo glass plates are separated by water. If surface tension of water is 75dyn/cm and the area of each plate wetted by water is 8cm2 and the distance between the plates is 0.12mm, then the force applied to separate the two plates is
A102dynB104dynC105dynD106dynA mercury drop shape as a round tablet of radius R and thickness h is located between two horizontal glass plates. Assuming that h<<R, find the mass m of a weight which has to be placed on the copper plate to diminish the distance between the plates by n-times the contact angle is equal to θ. calculate m if T is surface tension of the liquid.
Am=2πRT2|cosθ|gh(n2−1)Bm=2πR2T|sinθ|gh(n2−1)Cm=2πR2T|cosθ|gh(n2+1)Dm=2πR2T|cosθ|gh(n2−1)
Two vertical parallel glass plates are partically submerged in water. The distance between the plates is d=0.10mm, and their width is l=12cm assuming that the water between the plates does not reach the upper edges of the plates and the wetting is complete, it is found that the force of their mutual attraction, is (n+20)N. What is the value of n? (T=0.073Nm)
View SolutionA mercury drop shaped as a round tablet of radius R and and thickness h is located between two horizontal glass plates. Assuming that h<<R, find the mass m of a weight which has to be placed on the upper plate to diminish the distance between the plates n-times. The contact angle equals θ. Calculate m if R=2.0cm,h=0.38mm,n=2.0, and θ=135∘.
View SolutionA liquid has surface tension T dyne −cm−1. A drop of this liquid of volume 0.09cm3 is placed on the surface of a glass slide. Then another glass slide is placed on it in such a way that the liquid forms a thin layer of area 30cm2 between the surfaces of the two slides. To separate the slides, a force of 16×105 dyne has to be applied normal to the surfaces. Find the value of T.
View SolutionA small drop of water of surface tension S is squeezed between two clean glass plates so that a thin layar of thickness d and area A is formed between them. If the angle of contact is zero, what is the force required to pull the plates apart?
View SolutionA car moving at a speed of 36 km/h is taking a turn on a circular road of radius 50 m. A small wooden plate is kept on the seat with its plane perpendicular to the radius of the circular road figure. A small bock of mass 100 g is kept on the seat which rests against the plate. The friction coefficient between the block and the plate is μ=0.58. a. Find the normal contact force exerted by the plate on the block. b. The plate is slowly turned so that the angle between the normal to the plate and the radius of the road slowly increases Find the angle at which the block will just start sliding on the plate.

View Solution
