Determine the wavelength of spectral lines appearing on transition of exicted Li atoms from the state 3S down to the ground state 2S. The Rudberg corrections for the S and P terms are −0.41 and −0.04.
The enrgy of the 3S state is
E(3S)=−ħR(3−0.41)2=−2.03eV
The energy of a 2S state is
E(2S)=−ħR(2−0.41)2=−5.39eV
The energy of a 2P state is
E(2P)=ħR(2−.04)2=−3.55eV
We see that
E(2S)<E(2P)<E(3S)
The transitions are 3S→2P and 2P→2S.
Direct 3S→2S transition is forbidden by selection rules. The wavelength are determined by
E2−E1=ΔE=2πħcλ
Substitution gives
λ=0.816μm(2S→2P)
and λ=0.67μm(2P→2S)
Topper's Solved these Questions
- ATOMIC AND NUCLEAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ATOMIC AND NUCLEAR PHYSICSEXERCISE - Molecules And Crystals
48 Videos
- ATOMIC AND NUCLEAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ATOMIC AND NUCLEAR PHYSICSEXERCISE - Radioactivity
35 Videos
- ATOMIC AND NUCLEAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ATOMIC AND NUCLEAR PHYSICSEXERCISE - Wave Properties Of Particle
48 Videos
- DIRECT CURRENTBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - DIRECT CURRENTEXERCISE - All Questions
15 Videos
Similar Questions
Knowledge Check
The wavelength of the spectral line that corresponds to a transition in hydrogen atom from n=10 to ground state would be [In which part of electromagnetic spectrum this line liues?]
A92.25nm, ultravioletB92.25nm, infraredC86.95nm, ultravioletD97.65nm, ultravioletIf the shortest wavelength of spectral line of H-atom in Lyman series is x, then match the following for Li2+ and select the correct code.
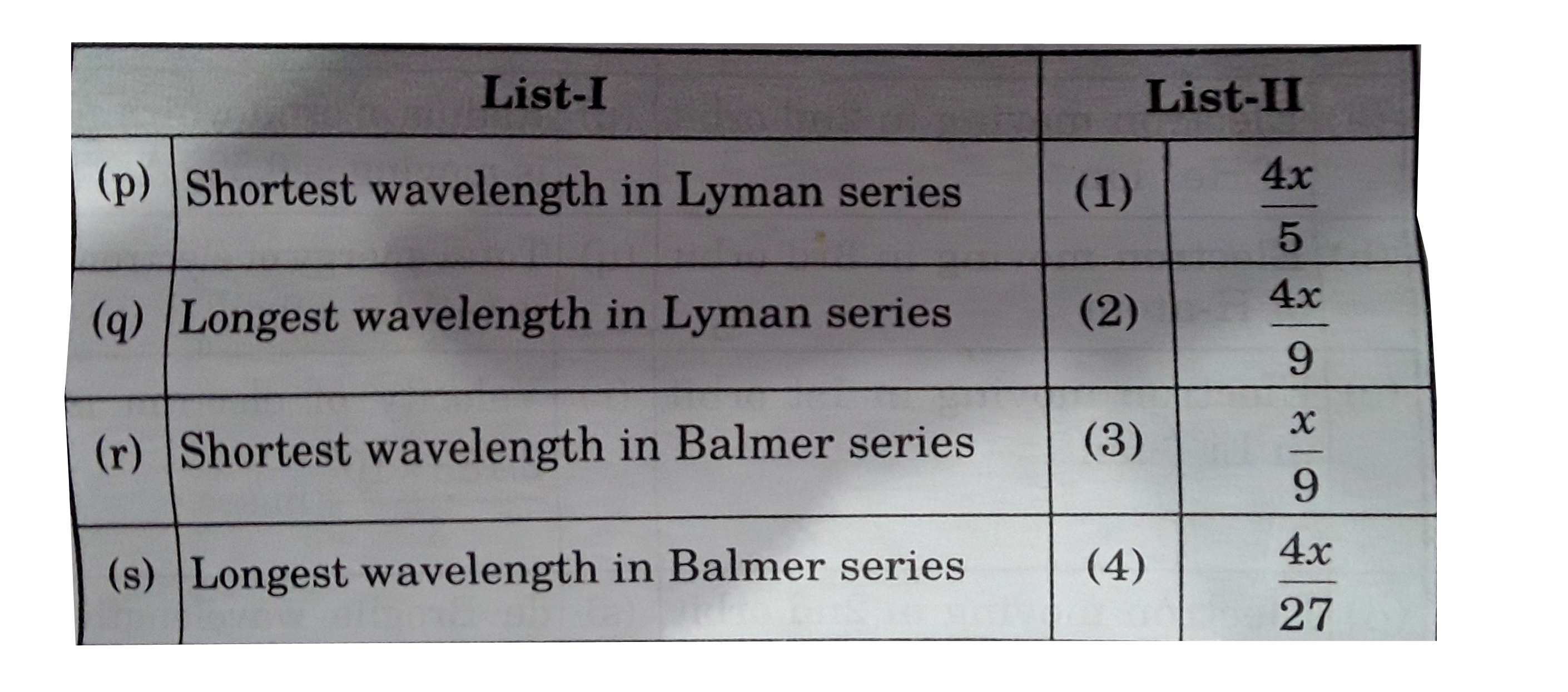
Codes :
p q r s " "p q r sA2 4 1 3B3 4 2 1C3 4 1 2D2 1 4 3For the hydrogen atom the energy of radiation emitted in the transitation from 4th excited state
to 2nd exicited state according to Bohr 's theory isA0.57 eVB0.667 eVC0.967 eVD1.267 eV
What is the wavelength in nm of the spectral line associated with a transition from n=3 to n= 2 for the Li2+ ion?
View SolutionThe wavelength of the spectral line that corresponds to a transition in hydrogen atom from n=10 to ground state would be [In which part of electromagnetic spectrum this line liues?]
View SolutionThe binding energy of the valance electron in a Li atom in the states 2S and 2P is equal to 5.39 and 3.54eV respectively. Find the Rydberg corrections for s and p terms of the atom.
View SolutionIf electron make transition from 7th excited state to 2nd state in H atom sample find the max. number of spectral lines observed.
View SolutionIf electron make transition from 7th excited state to 2nd state in H atom sample find the max. number of spectral lines observed.
View Solution
