If ABCDE is a pentagon, then
2
2
3
The correct Answer is:B
To solve the problem, we need to analyze the vector equation given for the pentagon ABCDE. The expression we need to evaluate is:
¯¯¯¯¯¯¯¯AB+¯¯¯¯¯¯¯¯AE+¯¯¯¯¯¯¯¯BC+¯¯¯¯¯¯¯¯¯DC+¯¯¯¯¯¯¯¯¯ED
1. Identify the Vectors:
- Let ¯¯¯¯¯¯¯¯AB be the vector from point A to point B.
- Let ¯¯¯¯¯¯¯¯AE be the vector from point A to point E.
- Let ¯¯¯¯¯¯¯¯BC be the vector from point B to point C.
- Let ¯¯¯¯¯¯¯¯¯DC be the vector from point D to point C.
- Let ¯¯¯¯¯¯¯¯¯ED be the vector from point E to point D.
2. Rearranging the Vectors:
- We can rearrange the expression to group the vectors that share common points:
¯¯¯¯¯¯¯¯AB+¯¯¯¯¯¯¯¯AE+¯¯¯¯¯¯¯¯BC+¯¯¯¯¯¯¯¯¯DC+¯¯¯¯¯¯¯¯¯ED=¯¯¯¯¯¯¯¯AB+¯¯¯¯¯¯¯¯BC+¯¯¯¯¯¯¯¯AE+¯¯¯¯¯¯¯¯¯ED+¯¯¯¯¯¯¯¯¯DC
3. Using Vector Addition:
- Notice that ¯¯¯¯¯¯¯¯AB+¯¯¯¯¯¯¯¯BC=¯¯¯¯¯¯¯¯AC (the vector from A to C).
- Similarly, we can combine ¯¯¯¯¯¯¯¯AE+¯¯¯¯¯¯¯¯¯ED=¯¯¯¯¯¯¯¯¯AD (the vector from A to D).
4. Combining the Results:
- Now we can rewrite the expression:
¯¯¯¯¯¯¯¯AC+¯¯¯¯¯¯¯¯¯AD+¯¯¯¯¯¯¯¯¯DC
5. Further Simplification:
- The segment ¯¯¯¯¯¯¯¯¯AD+¯¯¯¯¯¯¯¯¯DC can be combined as ¯¯¯¯¯¯¯¯AC since it connects points A to C through D.
- Therefore, we have:
¯¯¯¯¯¯¯¯AC+¯¯¯¯¯¯¯¯AC=2¯¯¯¯¯¯¯¯AC
6. Final Result:
- Thus, the final result is:
¯¯¯¯¯¯¯¯AB+¯¯¯¯¯¯¯¯AE+¯¯¯¯¯¯¯¯BC+¯¯¯¯¯¯¯¯¯DC+¯¯¯¯¯¯¯¯¯ED=2¯¯¯¯¯¯¯¯AC
Conclusion:
The expression evaluates to 2¯¯¯¯¯¯¯¯AC.
- Let ¯¯¯¯¯¯¯¯AB be the vector from point A to point B.
- Let ¯¯¯¯¯¯¯¯AE be the vector from point A to point E.
- Let ¯¯¯¯¯¯¯¯BC be the vector from point B to point C.
- Let ¯¯¯¯¯¯¯¯¯DC be the vector from point D to point C.
- Let ¯¯¯¯¯¯¯¯¯ED be the vector from point E to point D.
- We can rearrange the expression to group the vectors that share common points:
¯¯¯¯¯¯¯¯AB+¯¯¯¯¯¯¯¯AE+¯¯¯¯¯¯¯¯BC+¯¯¯¯¯¯¯¯¯DC+¯¯¯¯¯¯¯¯¯ED=¯¯¯¯¯¯¯¯AB+¯¯¯¯¯¯¯¯BC+¯¯¯¯¯¯¯¯AE+¯¯¯¯¯¯¯¯¯ED+¯¯¯¯¯¯¯¯¯DC
- Notice that ¯¯¯¯¯¯¯¯AB+¯¯¯¯¯¯¯¯BC=¯¯¯¯¯¯¯¯AC (the vector from A to C).
- Similarly, we can combine ¯¯¯¯¯¯¯¯AE+¯¯¯¯¯¯¯¯¯ED=¯¯¯¯¯¯¯¯¯AD (the vector from A to D).
- Now we can rewrite the expression:
¯¯¯¯¯¯¯¯AC+¯¯¯¯¯¯¯¯¯AD+¯¯¯¯¯¯¯¯¯DC
- The segment ¯¯¯¯¯¯¯¯¯AD+¯¯¯¯¯¯¯¯¯DC can be combined as ¯¯¯¯¯¯¯¯AC since it connects points A to C through D.
- Therefore, we have:
¯¯¯¯¯¯¯¯AC+¯¯¯¯¯¯¯¯AC=2¯¯¯¯¯¯¯¯AC
- Thus, the final result is:
¯¯¯¯¯¯¯¯AB+¯¯¯¯¯¯¯¯AE+¯¯¯¯¯¯¯¯BC+¯¯¯¯¯¯¯¯¯DC+¯¯¯¯¯¯¯¯¯ED=2¯¯¯¯¯¯¯¯AC
The expression evaluates to 2¯¯¯¯¯¯¯¯AC.
Topper's Solved these Questions
- VECTORSBOOK - MARVEL PUBLICATIONCHAPTER - VECTORSEXERCISE - PREVIOUS YEARS MHT-CET EXAM QUESTIONS
10 Videos
- VECTORSBOOK - MARVEL PUBLICATIONCHAPTER - VECTORSEXERCISE - TEST YOUR GRASP
20 Videos
- TRIGONOMETRIC FUNCTIONSBOOK - MARVEL PUBLICATIONCHAPTER - TRIGONOMETRIC FUNCTIONSEXERCISE - TEST YOUR GRASP: CHAPTER 3-3.3
13 Videos
Similar Questions
Knowledge Check
If ABCD is a square, then
¯¯¯¯¯¯AB+2¯¯¯¯¯¯BC+3¯¯¯¯¯¯CD+4¯¯¯¯¯¯DA =A4 ¯¯¯¯¯¯ACB3 ¯¯¯¯¯¯ACC2 ¯¯¯¯¯¯CAD¯¯¯¯¯¯ACIf ABCDE is a regular penatagon then :
¯¯¯¯¯¯AB+¯¯¯¯¯¯BC+¯¯¯¯¯¯AD+¯¯¯¯¯¯ED+¯¯¯¯¯¯AE=A¯¯¯¯¯¯AC−2¯¯¯¯¯¯DCB3¯¯¯¯¯¯AC−2¯¯¯¯¯¯DCC¯¯¯¯¯¯CA−¯¯¯¯¯¯DCD3¯¯¯¯¯¯AC−¯¯¯¯¯¯DCIf ABCDEF is a regular hexagon , then
¯¯¯¯¯¯AB+¯¯¯¯¯¯AC+¯¯¯¯¯¯AE+¯¯¯¯¯¯AF =A4¯¯¯¯¯¯ADB3¯¯¯¯¯¯ADC2¯¯¯¯¯¯ADD¯¯¯¯¯¯AD
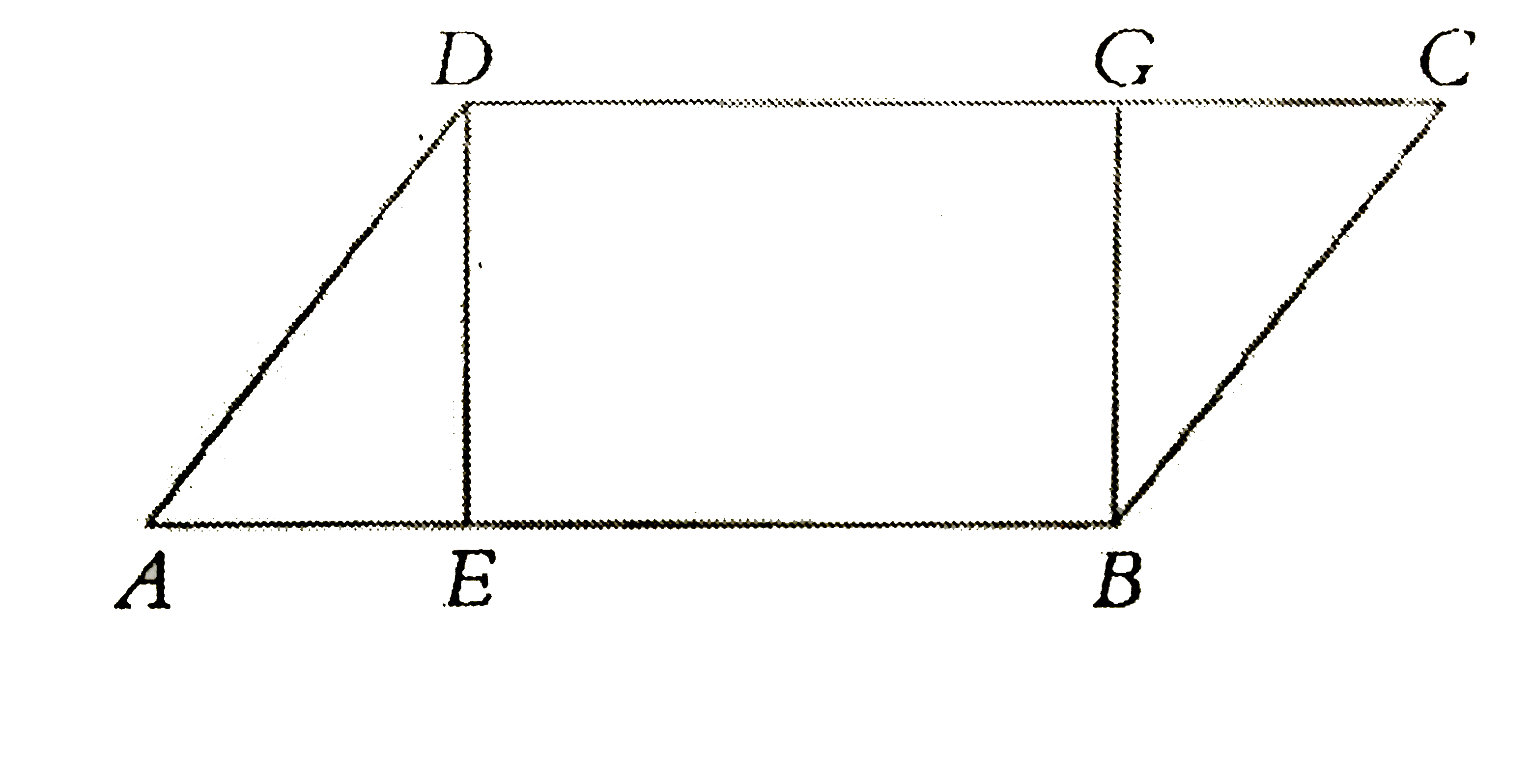
In the figure above, ABCD is a parallelogram, ¯¯¯¯¯¯BE⊥¯¯¯¯¯¯AB,¯¯¯¯¯¯BG⊥¯¯¯¯¯¯CD, and EBGD is a square. If BG=12 cm and BC=13 cm , then find AB.
View SolutionIf PQRST is a pentagon, then
¯¯¯¯¯¯PQ+¯¯¯¯¯¯PR+¯¯¯¯¯PS−¯¯¯¯¯¯TQ−¯¯¯¯¯¯TR−¯¯¯¯¯TS =
View SolutionIf diagonals of a parallelogram ABCD intersect each other in M, then ¯¯¯¯¯¯OA+¯¯¯¯¯¯OB+¯¯¯¯¯¯OC+¯¯¯¯¯¯OD =
View SolutionIf ABCD is a square then,
2¯¯¯¯¯¯AB+4¯¯¯¯¯¯BC+5¯¯¯¯¯¯CD+7¯¯¯¯¯¯DA=
View SolutionIn a ΔABC,¯¯¯¯¯¯AB2+¯¯¯¯¯¯AC2=¯¯¯¯¯¯BC2 and ¯¯¯¯¯¯BC=√2¯¯¯¯¯¯AB , then ∠ABCis :
View Solution
