The potential energies of a linear harmonic oscillator at the mean position, extreme positions and an intermediate position are 0, 0.5J and 0.3 J respectively. Its total energy is given by
0.7 J
0.5 J
0.8 J
0.25 J
The correct Answer is:B
The linear harmonic oscillator has maximum potential energy, when it is in the extreme position and Maximum P.E. =Total energy =0.5 J
Topper's Solved these Questions
Similar Questions
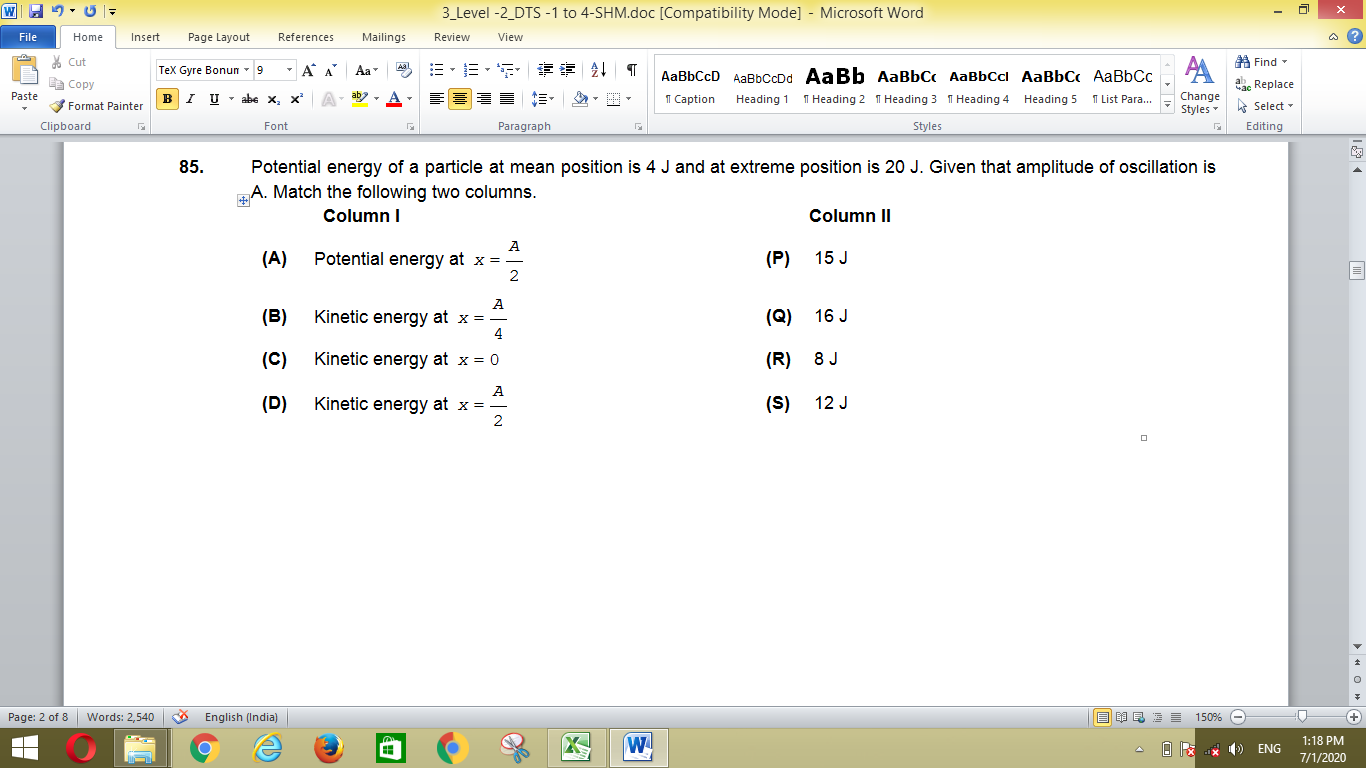
Potential energy of a particle at mean position is 4 J and at extreme position is 20 J. Given that amplitude of oscillation is A. Match the following two columns
View SolutionFor a linear harmonic oscillator, its potential energy, kinetic energy and total energy given by EP,KKandET respectively. Its maximum acceleration is given by
View Solution
Knowledge Check
In a simple harmonic oscillator, at the mean position
A(a) kinetic energy is minimum, potential energy is maximum.B(b) both kinetic and potential energies are maximum.C(c ) kinetic energy is maximum, potential energy is minimum.D(d) both kinetic and potenteal energies are minimum.In. a simple harmonic oscillator, at the mean position
Akinetic energy Is minimum potential energy Is maximumBBoth kinetic and potential energy are maximumCkinetic energy Is maximum, potential energy Is minimumDBoth kinetic and potential energies are minimumThe potential energy of a particle performing S.H.M. at extreme position is
AminimumBmaximumCremains constantD1/2mω2x2
The potential energy of a harmonic oscillator of mass 2 kg in its mean positioin is 5J. If its total energy is 9J and its amplitude is 0.01m, its period will be
View SolutionThe potential energy of a particle oscillating on x-axis is given as U+20+(x−2)2. The mean position is at
View SolutionThe potential energy of a simple harmonic oscillator of mass 2 kg in its mean position is 5 J. If its total energy is 9 J and its amplitude is 0.01 m, its time period would be
View SolutionThe total energy of a harmonic oscillator of mass 2 kg is 9 J. If its potential energy at mean position is 5 J , its KE at the mean position will be
View SolutionWhen a particle executing a linear S.H.M. moves from its extreme position to the mean position, its
View Solution
