A simple pendulum oscillates with an anglular amplitude of $θ$ . If the maximum tension in the string is 3 times the minimum tension, then the value of $cos θ$ is

A

$\frac{1}{2}$

B

$\frac{2}{3}$

C

$\frac{3}{4}$

D

$\frac{3}{5}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:D

Maximum tension is at O and minimum tension is at A.
By the principle of conservation at energy
K.E. at O=P.E. at A
$∴ \frac{1}{2} m v^{2} = m g h = m g l (1 - cos θ)$
$∴ v^{2} = 2 g l (1 - cos θ)$

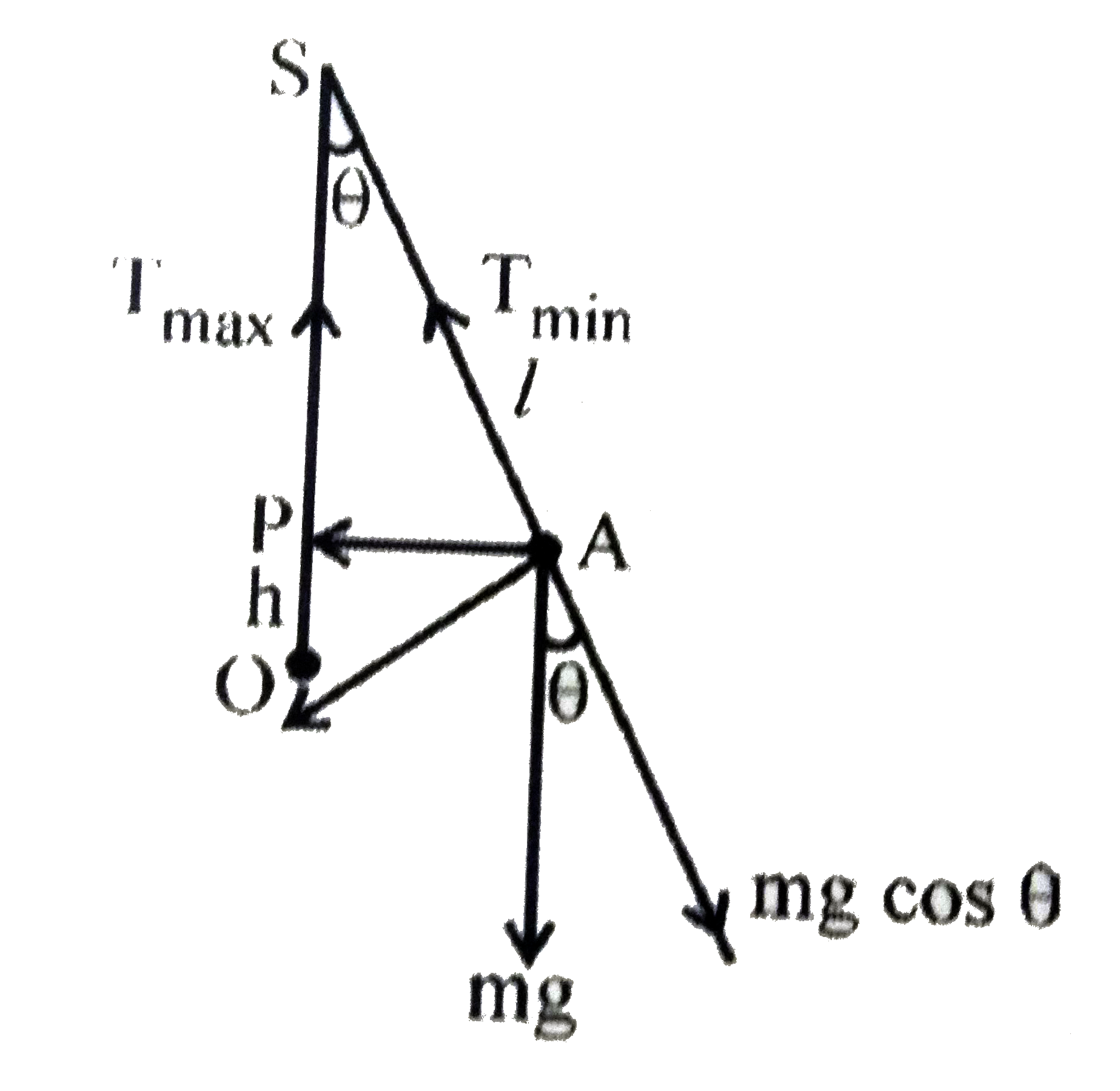
$T_{max} = m g + \frac{m v^{2}}{l}$
$= m g + \frac{m}{l} [2 g l (1 - cos θ)]$
$= 3 m g - 2 m g cos θ$
and $T_{min} = m g cos θ$
$∵ T_{max} = 3 T_{min}$
$∴ 3 m g - 2 m g cos θ = 3 m g cos θ$
$∴ 3 = 5 cos θ ∴ cos θ = \frac{3}{5}$

|

Updated on:21/07/2023

Class 12 PHYSICS OSCILLATIONS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The maximum tension in the string of a pendulum is two times the minimum tension. Let $θ_{0}$ is
A $\frac{1}{2}$
B $\frac{3}{4}$
C $\frac{2}{3}$
D $\frac{3}{5}$
Question 2 - Select One
The maximum tension in the string of a pendulum is three times the minimum tension:
AMaximum tension in string is $\frac{4 m g}{5}$
BMaximum tension in string is $\frac{6 m g}{5}$
CMaximum speed of bob is $3 \sqrt{\frac{g l}{5}}$
DMaximum speed of bob is $2 \sqrt{\frac{g l}{5}}$
Question 3 - Select One
Tension in the string is minimum when the pendulum is at
Amean position
Bextreme position
Cmid way between mean and extreme position
Dany position

MARVEL PUBLICATION-OSCILLATIONS-MCQ

Home
Ask Doubt
Profile