A stationary sound wave has a frequency of 165 Hz. If the speed of sound in air is 330 m/s , then the distance between a node and the adjacent antinode is
30 cm
40 cm
50 cm
60cm
The correct Answer is:C
λ=Vn=33000165=200 cm
The distance between a node and the adjacent antinode
=λ4=50 cm
Topper's Solved these Questions
- BOOK - MARVEL PUBLICATIONCHAPTER - STATIONARY WAVESEXERCISE - MULTIPLE CHOICE QUESTIONS (HIGHER LEVEL )7videos
STATIONARY WAVES
- BOOK - MARVEL PUBLICATIONCHAPTER - STATIONARY WAVESEXERCISE - MULTIPLE CHOICE QUESTIONS ( STANDARD LEVEL )65videos
STATIONARY WAVES
- BOOK - MARVEL PUBLICATIONCHAPTER - SEMICONDUCTORS EXERCISE - MCQs261videos
SEMICONDUCTORS
- BOOK - MARVEL PUBLICATIONCHAPTER - SURFACE TENSION EXERCISE - MULTIPLE CHOICE QUESTIONS . (TEST YOUR GRASP )10videos
SURFACE TENSION
Similar Questions
In a stationary wave of wavelength 0.4 m, the distance between node and adjacent antinode is ________.
View SolutionA stationary police car sounds a siren with a frequency of 990 Hz. If the speed of sound is 330 m/s, an observer, driving towards the car with a speed of 33 m/s, will hear a frequency of
View Solution
Knowledge Check
- Question 1 - Select One
In stationary wave, the distance between a node and its adjacent antinode is ……….
AλBλ4Cλ2D2λ - Question 2 - Select One
A car is moving with a velocity of 5m/s towards huge wall. The driver sounds a horn of frequency 165Hz. If the speed of sound in air is 335m/s, the number of beats heard per second by the driver is
A6B5C4D3 - Question 3 - Select One
A violin string emits sound waves with a frequency of 850 Hz as shown in the given figure.
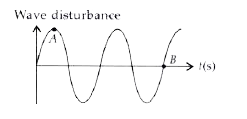
If the speed of sound in air is 340 m s−1, the distance between points A and B isA6 mB7 mC60 cmD70 cm
What is the distance between a node and an adjoining antinode in a stationary wave?
View SolutionA tube closed at one end has a vibrating diaphragm at the other end , which may be assumed to be a displacement node . It is found that when the frequency of the diaphragm is 2000Hz , a stationary wave pattern is set up in which the distance between adjacent nodes is 8cm. When the frequency is gradually reduced , the stationary wave pattern reappears at a frequency of 1600Hz. Calculate
i. the speed of sound in air ,
ii. the distance between adjacent nodes at a frequency of 1600Hz,
iii. the distance between the diaphragm and the closed end ,
iv. the next lower frequencies at which stationary wave patterns will be obtained.View SolutionAn open pipe of length 33 cm resonates with frequency of 100 Hz . If the speed of sound is 330 m/s , then this frequency is
View SolutionTwo sound waves each of frequency 500Hz are travelling along the same line in opposite direction . If the speed of sound in air is 350 m/s. find the distance between the consecutive antinodes of the resulting stationary wave.
View SolutionAn open pipe of length 33cm resonates with frequency of 1000Hz. If the speed of sound is 330m/s , then this frequency is -
View Solution

