Two stars X and Y emit blue and yellow lights respectively. What is the relation between their temperatures ( $T_{x} and T_{y}$ ) ?

A

$T_{x} > T_{y}$

B

$T_{x} < T_{y}$

C

$T_{x} = T_{y}$

D

$T_{x} = \frac{1}{2} T_{y}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

X - Blue Y - Yellow
$∵ λ_{Y} > λ_{B} ∴ T_{Y} < T_{X}$

|

Updated on:21/07/2023

Class 12 PHYSICS KINETIC THEORY OF GASES ,THERMODYNAMICS AND RADIATION

Topper's Solved these Questions

BOOK - MARVEL PUBLICATIONCHAPTER - KINETIC THEORY OF GASES ,THERMODYNAMICS AND RADIATIONEXERCISE - TEST YOUR GRASP - 9
30videos
KINETIC THEORY OF GASES ,THERMODYNAMICS AND RADIATION
BOOK - MARVEL PUBLICATIONCHAPTER - INTERFERENCE AND DIFFRACTIONEXERCISE - TEST YOUR GRASP
20videos
INTERFERENCE AND DIFFRACTION
BOOK - MARVEL PUBLICATIONCHAPTER - MAGNETIC EFFECTS OF ELECTRIC CURRENTEXERCISE - MCQ
195videos
MAGNETIC EFFECTS OF ELECTRIC CURRENT

Similar Questions

Knowledge Check

Question 1 - Select One
Two absolute scales $X$ and $Y$ assigned numerical values $200$ and $450$ to the triple of water. What is the relation between $T_{X}$ and $T_{Y}$ ?
A $9 T_{X} = 4 T_{Y}$
B $4 T_{X} = 9 T_{Y}$
C $T_{X} = 3 T_{Y}$
DNone of these
Question 2 - Select One

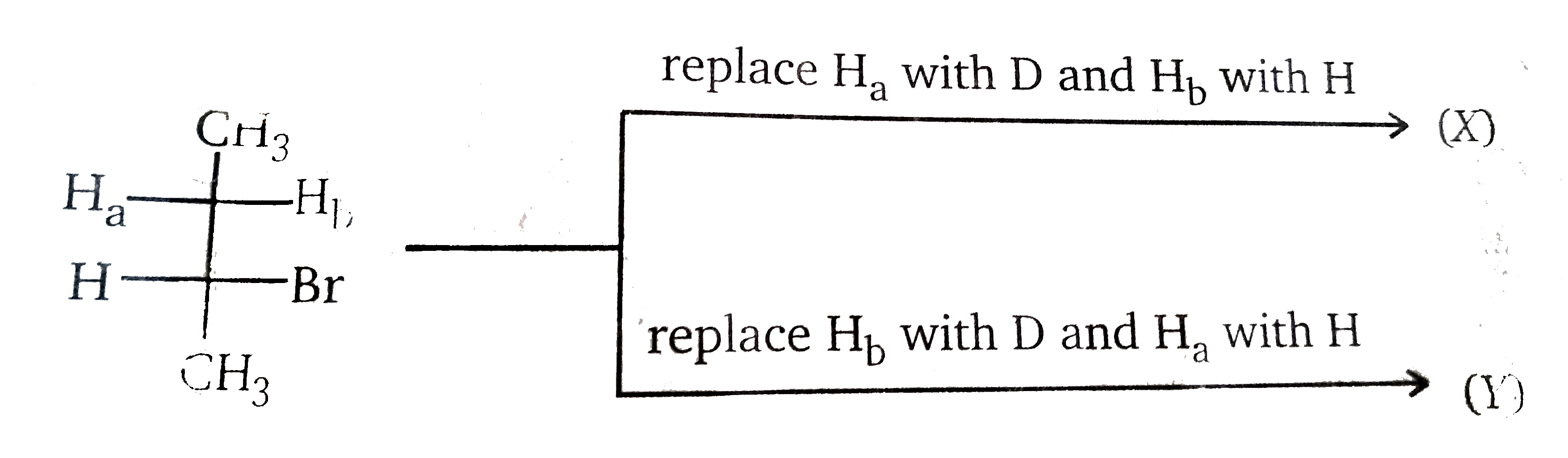
Relation between (X) and (Y) is :
Awnantiomers
Bdiastereomers
CE and Z isomer
Dconstitutional isomer
Question 3 - Select One
The sun emits light with a maximum wavelength of 510 nm while another star X emits light of maximum wavelength of 350 nm. What is the ratio of the surface temperatures of the sun and the star ?
A0.35
B1.5
C1.1
D0.69

MARVEL PUBLICATION-KINETIC THEORY OF GASES ,THERMODYNAMICS AND RADIATION-TEST YOUR GRASP - 9

Home
Ask Doubt
Profile