In a regular hexagon ABCDEF, −−→AE
¯¯¯¯¯¯AC+¯¯¯¯¯¯AB+¯¯¯¯¯¯AF
¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯AC−+¯¯¯¯¯¯AB+¯¯¯¯¯¯AF
¯¯¯¯¯¯AC+¯¯¯¯¯¯AB−¯¯¯¯¯¯AF
−¯¯¯¯¯¯AC+¯¯¯¯¯¯AB+¯¯¯¯¯¯AF
The correct Answer is:B
Step by step video, text & image solution for In a regular hexagon ABCDEF, vec(AE) by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Knowledge Check
In a regular hexagon ABCDEF,
→AB+→AC+→AD+→AE+→AF=A4→ADB3→ADC2→ADD0If ¯aand¯b represent the sides ¯¯¯¯¯¯ABand¯¯¯¯¯¯BC of a regular hexagon ABCDEF, then ¯¯¯¯¯¯FA=
Ab−aBa−bCa+bDnone of theseIn a regualr hexagon ABCDEF, A→B=→a,B→C=→bandC→D=→c. Then A→E=
A→a+→b+→cB2→a+→b+→cC→a+→cD→a+2→b+2→c
In a regular hexagon ABCDEF, prove that −−→AB+−−→AC+−−→AD+−−→AE+−−→AF=3−−→AD
View SolutionThe coordinates of two consecutive vertices A and B of a regular hexagon ABCDEF are (1,0) and (2,0) respectively. The equation of the diagonal CE is:
View Solutiona and b form the consecutive sides of a regular hexagon ABCDEF.
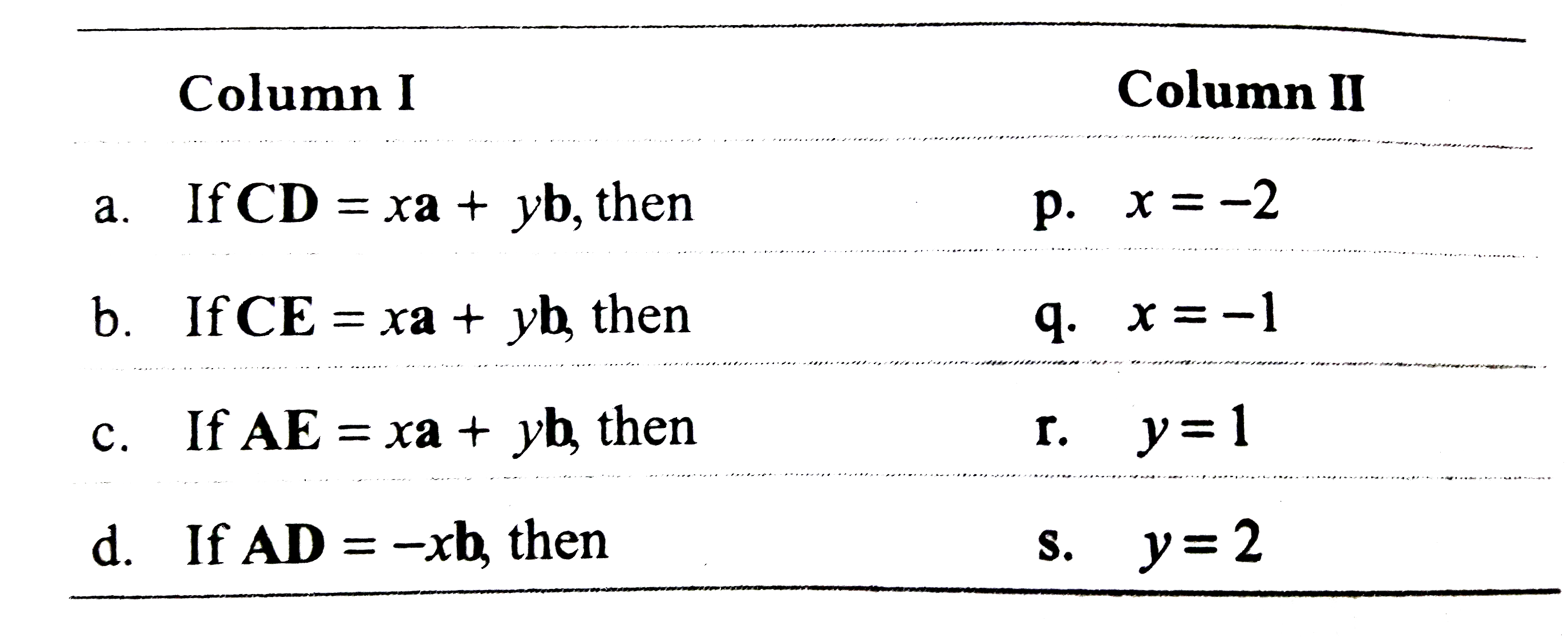
View SolutionIn a regular hexagon , →AE =
View Solution¯a,¯b are vectors, ¯¯¯¯¯¯AB,¯¯¯¯¯¯BC determined by two adjacent sides of a regular hexagon ABCDEF.The vector represented by ¯¯¯¯¯¯EF is
View Solution
