Question

If a,b,c and d are any four consecutive
coefficients in the expansion of ${(1+x)}^n$ , then prove that
(i)$\frac{a}{a+b}+\frac{c}{b+c}=\frac{2b}{b+c}$
(ii) ${\left(\frac{b}{b+c}\right)}^2>\frac{ac}{(a+b)(c+d)},\text{if}x>0$ .

Explanation

Let a,b,c and d be the coefficient of the r th,(r + 1) th
(r +2)th and (r + 3)th terms respectively , in the expansion oof ` (1 + x)^(n)` . Then
`T_(r) = T_(r-1 + 1) = ""^(n)C_(r-1) x^(r-1)`
` therefore a= ""^(n)C_(n-1)` …(i)
` because T_(r+1) = ""^(n)C_(r) x^(r)`
` therefore b = ""^(n)C_(r) ` ...(ii)
` because T_(r+2) = T_((r+ 3) +1) = ""^(n)C_(r+1) x^(r +1)`
` therefore c = ""^(n)C_(r +1)` ... (iii)
and ` T_(r +3) = T_((r + 2) + 1)=""^(n)C_(r+2) x^(r +2)`
` therefore d = ""^(n)C_(r +2) ` ...(iv)
From Equ . (i) and (ii) , we get
` a + b = ""^(n)C_(r-1) + ""^(n)C_(r) = ""^(n+1)C_(r)`
` = (n +1)/(r) . ""^(n)C_(r-1) = ((n +1)/(r))a `
` therefore (a)/(a+b) = (r)/(n +1) ` ...(v)
From Eqs. (ii) and (iii), we get
` b + c = ""^(n)C_(r +1) = ""^(n+1)C_(r+1) `
` = ((n+1)/(r+1)) ""^(n)C_(r) = ((n+1)/(r +1)) b `
` therefore (b) /(b +c) = (r +1)/(n+1) `...(vi)
From Eqs.(iii) and (iv) , we get ltbr ` c + d = ""^(n)C_(r+1) = ((n+1)/(r +2))c `
` therefore (c)/(c+ d) = (r+2)/(n+1) ` ...(vii)
From Eqs (v) , (vi) and (vii) , we get
` (a)/(a+ b) , (b)/(b +c) "and " (c)/(c +d)` are in AP
(i) ` (a)/(a + b) + (c)/(c + d) = 2 ((b)/(b + c))`
or ` (a)/(a + b) + (c)/(c + d) = (2b)/(b + c)`
(ii) `AM gt GM `
`therefore ((b)/(b + c)) gt sqrt(((a)/(a + b))((c)/(c + d))) `
` rArr ((b)/(b + c))^(2) gt (ac)/((a + b)(c + d))`
Remembering Method

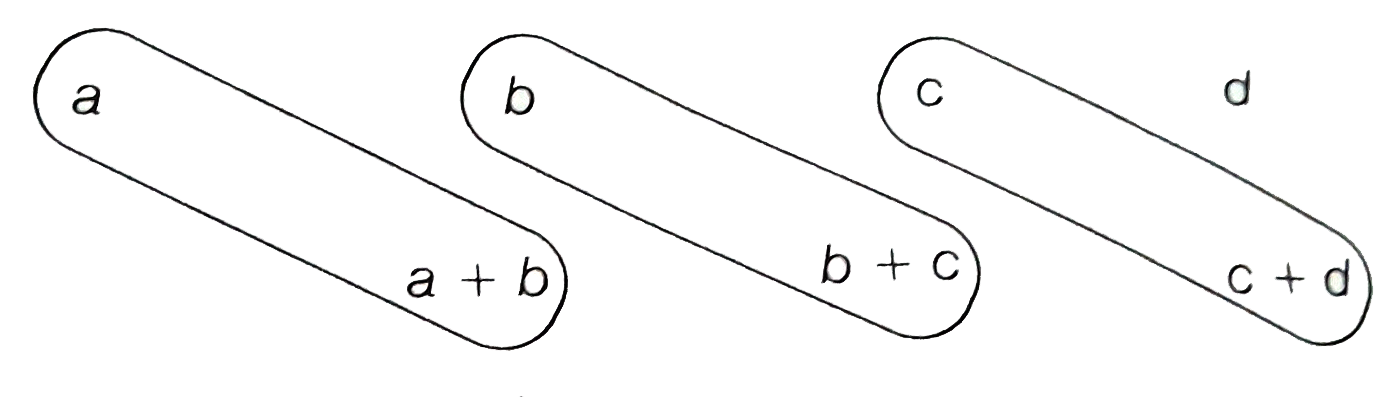
`(a)/(a + b) , (b)/(b + c) " and " (c)/(c + d) ` are in AP .