In ΔABC, P is the mid point of BC,Q divides CA internally in the ratio 2:1 and R divides AB externally in the ratio 1:2 then
R divides PQ externally in the ratio 2:1
P,Q,R are collinear
P divides QR externally in the ratio 3:2
Q divides PR internally in the ration 3:2
The correct Answer is:B
Step by step video, text & image solution for In DeltaABC, P is the mid point of BC,Q divides CA internally in the ratio 2:1 and R divides AB externally in the ratio 1:2 then by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- VECTORSBOOK - TARGET PUBLICATIONCHAPTER - VECTORSEXERCISE - Competitive Thinking
79 Videos
- VECTORSBOOK - TARGET PUBLICATIONCHAPTER - VECTORSEXERCISE - Evaluation Test
18 Videos
- VECTORSBOOK - TARGET PUBLICATIONCHAPTER - VECTORSEXERCISE - Evaluation Test
18 Videos
- TRIGONOMETRIC FUNCTIONS BOOK - TARGET PUBLICATIONCHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - Evaluation Test
34 Videos
Similar Questions
If point P(3,2) divides the line segment AB internally in the ratio of 3:2 and point Q(−2,3) divides AB externally in the ratio 4:3 then find the coordinates of points A and B.
View Solution→a,→b,→c are the position vectors of the three points A,B,C respectiveluy. The point P divides the ilne segment AB internally in the ratio 2:1 and the point Q divides the lines segment BC externally in the ratio 3:2 show that 3→)PQ)=−→a−8→b+9→c.
View Solution
Knowledge Check
A(x1,y1),B(x2,y2),C(x3,y3) are three vertices of a triangle ABC. lx+my+n=0 is an equation of the line L.
If P divides BC in the ratio 2:1 and Q divides CA in the ratio 1:3 then R divides AB in the ratio (P,Q,R are the points as in problem 1)A2:3 internallyB2:3 externallyC3:2 internallyD3:2 externallyThe point (107,337) divides P(1,3) and Q(2,7) internally in the ratio :
A3:4B3:2C2:3D4:3In ΔABC,L,M,N are points on BC,CA,AB respectively, dividing them in the ratio 1:2,2:3,3:5, if the point K divides AB in the ratio 5:3, then ∣∣¯¯¯¯¯¯AL+¯¯¯¯¯¯¯BM+¯¯¯¯¯¯CN∣∣∣∣¯¯¯¯¯¯CK∣∣=
A115B25C58D35
If P divides OA internally in the ratio λ1:λ2 and Q divides OA externally in the ratio λ1;λ2, then prove that OA is the harmonic mean of OP and OQ.
View SolutionIn figure, in what ratio, P divides AB internally.
View SolutionFrom the following figure, point P divides AB internally in the ratio ________.
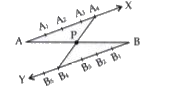
View SolutionFind the coordinates of the point R which divides PQ externally in the ratio 2:1 and verify that Q is the mid point of PR.
View SolutionIn a ΔABC, a line is drawn passing through centroid dividing AB internaly in ratio 2:1 and AC in λ:1 (internally). The value of λ is
View Solution
