In the Cartesian plane, O is the origin of the coordinate axes. A person starts at O and walks a distance of 3 units in the NORTH−EAST direction and reaches the point P. From P he walks 4 units distance of 3 parallel to NORTH−WEST direction and reaches the point Q. Express the vector OQ in terms of iandj (observe that ∣(XOP)=45∘).
Step by step video & image solution for In the Cartesian plane, O is the origin of the coordinate axes. A person starts at O and walks a distance of 3 units in the NORTH-EAST direction and reaches the point P. From P he walks 4 units distance of 3 parallel to NORTH - WEST direction and reaches the point Q. Express the vector OQ in terms of i and j (observe that |(XOP)=45^@). by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
In the Cartesian plane, a man starts at origin and walks a distance of 3 units of the north-east direction and reaches a point P. from P, he walks a distance of 4 units in the north-west direction to reach a point Q. construct the parallelogram OPQR with OP and PQ as adjacent sides. let M be the mid-point of PQ.
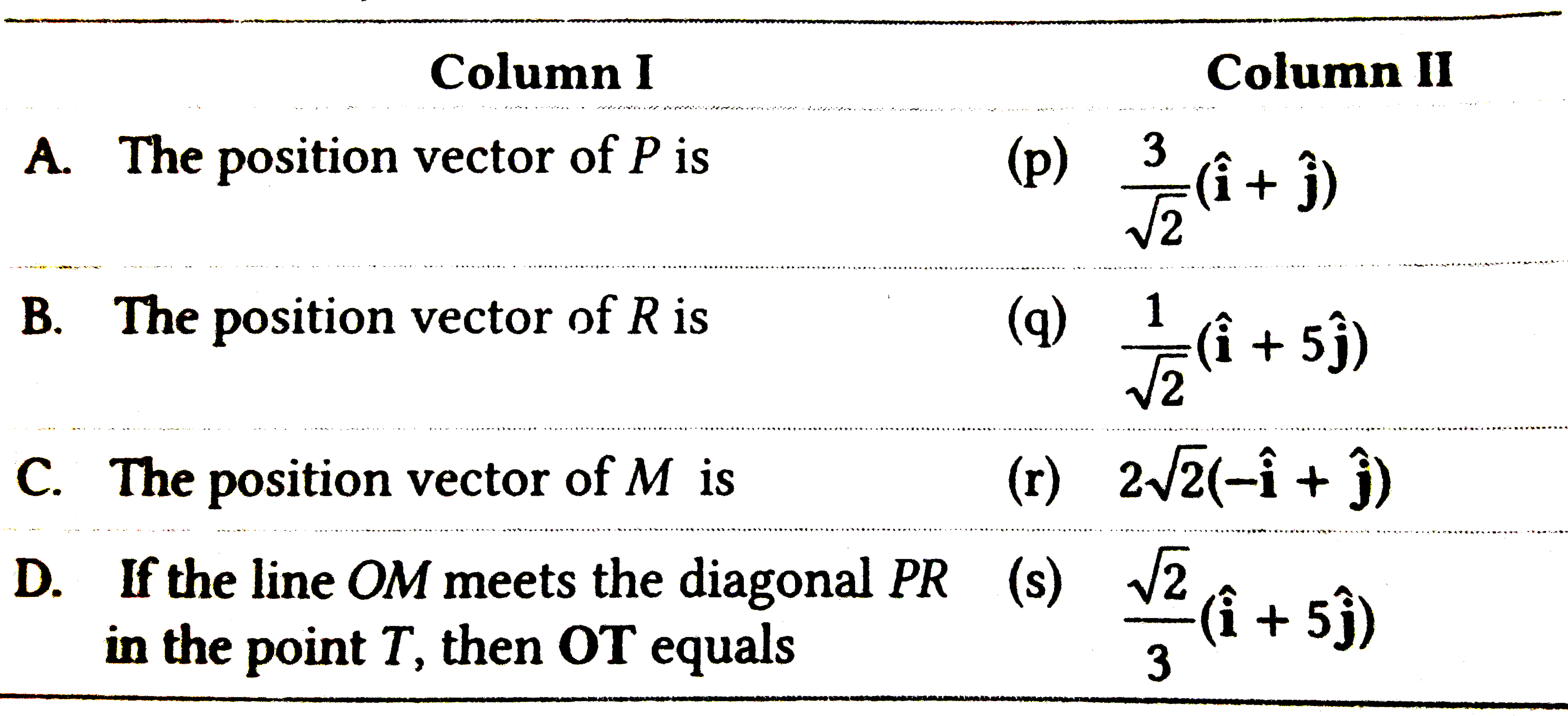
View SolutionA man walks a distance of 3 units from the origin towards north-east to reach position A. from there he walks a distance of 4 units towards North west to reach position P. Then position of P in argand plane is
View Solution
Knowledge Check
A man walks a distance of 3 units from the origin towards the North-East (N450E) direction.From there, he walks a distance of 4 units towards the North-West (N450W) direction to reach a point P. Then, the position of P in the Argand plane is 3eiπ4+4i (b) (3−4i)eiπ4 (4+3i)eiπ4 (d) (3+4i)eiπ4
A3eiπ/4+4iB(3−4i)eiπ/4C(4+3i)eiπ/4D(3+4i)eiπ/4O is the origin and P is point at a distance of 3 units from origin. If direction ratios of OP are <1,−2,−2>, then the coordinates of P are
A(1,−2,−2)B(3,−6,−6)C(13,−23,−23)D(19,−29,−29)Let O be the origin and P be the point at a distance 3 units from origin. If direction ratios of OP are (1,−2,−2), then coordinates of P is given by
A(1,−2,−2)B(3,−6,−6)C(1/3,−2/3,−2/3)D(1/9,−2/9,−2/9)
A man walks 6 km towards north and turns left and walks 2 kms towards West. If he has started from point Z and reached point A, then what is the shortest distance between point Z and A
View SolutionIf the distance between the origin O and point P is 5 units and the direction ratios of line segment are (1,−2,3) then the coordinates of P are
View SolutionLet O be the origin and P be the point at a distance 3 units from origin. If direction ratios of OP are (1,-2,-2), then coordinates of P is given by
View SolutionA person starts walking in south direction and walks a distance of 7 meters. Now he tooks a left turn and walk 6m. Again he talks a left turn and walks 15m and reached a point P. Find the distance between starting point and P and in which direction is the person from the initial point.
View SolutionEach of the questions below consists of a question and two statements numbered I and II given below it. You have to decide whether the data provided in the statement are sufficient to answer the question . Read both the statements and -
In which direction point 'A' islocated with respect to point 'B' ?
I. A man starts walking from point 'A' towards east and after walking 3 mitres reached point 'N', he turns right and walks 6 metres to reach point 'M'. Then he turns right ans walks 6 metres to reach point 'O'. He again turns right and walks 7 walks 7 metres to reach point 'P'. He, then, turns left and walks 2 metres to reach point 'B'.
II. A man starts walking from point 'A' towards east and after walking 3 metres reaches point 'N'. From point 'N' he walks 7 metres towards south and reaches point 'M'. From point 'M' he walks 6 metres towards west and reaches Point 'O'. From potin 'O' he walks 7 metres towards north and reaches point 'P'. From point 'P' he walks towards west and reaches point 'B' . The distance between point A and B is 8 metres.
View Solution
