Two particle of masses 4kg and 8kg are kept at x=−2m and x=4m respectivley. Then, the gravitational field intensity at the origin is
G
2G
G/2
G/4
The correct Answer is:C
To find the gravitational field intensity at the origin due to two particles of masses 4 kg and 8 kg located at x = -2 m and x = 4 m respectively, we will follow these steps:
Step 1: Identify the positions of the masses
- Mass m1=4kg is located at x=−2m.
- Mass m2=8kg is located at x=4m.
Step 2: Calculate the distances from the origin
- The distance from the origin to m1 is r1=2m (since it's at -2 m).
- The distance from the origin to m2 is r2=4m (since it's at 4 m).
Step 3: Calculate the gravitational field intensity due to each mass
The gravitational field intensity E due to a mass m at a distance r is given by the formula:
E=G⋅mr2
where G is the gravitational constant.
For mass m1:
E1=G⋅422=G⋅44=G
The direction of E1 is towards the mass m1, which is to the left (negative x-direction), so:
E1=−G^i
For mass m2:
E2=G⋅842=G⋅816=G2
The direction of E2 is towards the mass m2, which is to the left (negative x-direction), so:
E2=−G2^i
Step 4: Calculate the net gravitational field intensity at the origin
The net gravitational field intensity Enet at the origin is the vector sum of E1 and E2:
Enet=E1+E2=−G^i−G2^i
Enet=−(G+G2)^i=−3G2^i
Final Result
Thus, the gravitational field intensity at the origin is:
Enet=−3G2^i
- Mass m1=4kg is located at x=−2m.
- Mass m2=8kg is located at x=4m.
- The distance from the origin to m1 is r1=2m (since it's at -2 m).
- The distance from the origin to m2 is r2=4m (since it's at 4 m).
The gravitational field intensity E due to a mass m at a distance r is given by the formula:
E=G⋅mr2
where G is the gravitational constant.
E1=G⋅422=G⋅44=G
The direction of E1 is towards the mass m1, which is to the left (negative x-direction), so:
E1=−G^i
E2=G⋅842=G⋅816=G2
The direction of E2 is towards the mass m2, which is to the left (negative x-direction), so:
E2=−G2^i
The net gravitational field intensity Enet at the origin is the vector sum of E1 and E2:
Enet=E1+E2=−G^i−G2^i
Enet=−(G+G2)^i=−3G2^i
Thus, the gravitational field intensity at the origin is:
Enet=−3G2^i
Topper's Solved these Questions
- GRAVITATIONBOOK - NARAYNACHAPTER - GRAVITATIONEXERCISE - LEVEL-II (H.W)
32 Videos
- GRAVITATIONBOOK - NARAYNACHAPTER - GRAVITATIONEXERCISE - LEVEL-V
54 Videos
- GRAVITATIONBOOK - NARAYNACHAPTER - GRAVITATIONEXERCISE - NCERT BASED QUESTIONS
26 Videos
- FRICTIONBOOK - NARAYNACHAPTER - FRICTIONEXERCISE - Passage type of questions I
6 Videos
- KINETIC THEORY OF GASESBOOK - NARAYNACHAPTER - KINETIC THEORY OF GASESEXERCISE - LEVEL-III(C.W)
52 Videos
Similar Questions
Two particles of masses 0.2 kg and 0.8 kg are separated by 12 cm. At which point from the 0.2 kg particle, gravitational field intensity due to the two particles is zero?
View SolutionTwo particle of mass 1 kg and 2 kg are located at x = 0 and x = 3 m. Find the position of their centre of mass.
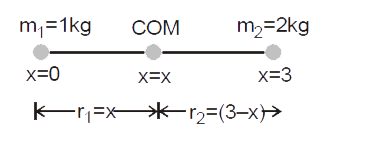
View Solution
Knowledge Check
Two bodies of masses 2 kg and 8 kg are separated by a distance of 9 m. The point where the resultant gravitational field intensity is zero at the distance of
A4.5 m from each massB6 m from 2 kgC6 m from 8 kgD2.5 m from 2 kgTwo particles of masses 1kg and 2kg are located at x=0 and x=3m. Find the position of their centre of mass.
A2m from 1 kgB2m from 2 kgC1m from 1 kgDnone of theseTwo bodies of masses 50 kg and 100 kg are at a distance 1m apart. The intensity of gravitational field at the mid-point of the line joining them is (in joules)
A100GB150GC50GD200G
Two masses 90kg and 160kg are 5m apart. The gravitational field intensity at a point 3m from 90kg and 4m from 160kg is
View SolutionMasses 2kg and 8kg are 18cm apart. The point where the gravitational field due to them is zero, is
View SolutionMasses 4kg and 36kg are 16cm apart. The point where the gravitational field due to them is zero is
View SolutionMasses 8 kg and 2 kg are 18 cm apart. The point where the gravitational field due to the masses is zero is
View SolutionMasses of 1kg each are placed 1m,2m,4m,8m, ... from a point P. The gravitational field intensity at P due to these masses is
View Solution
