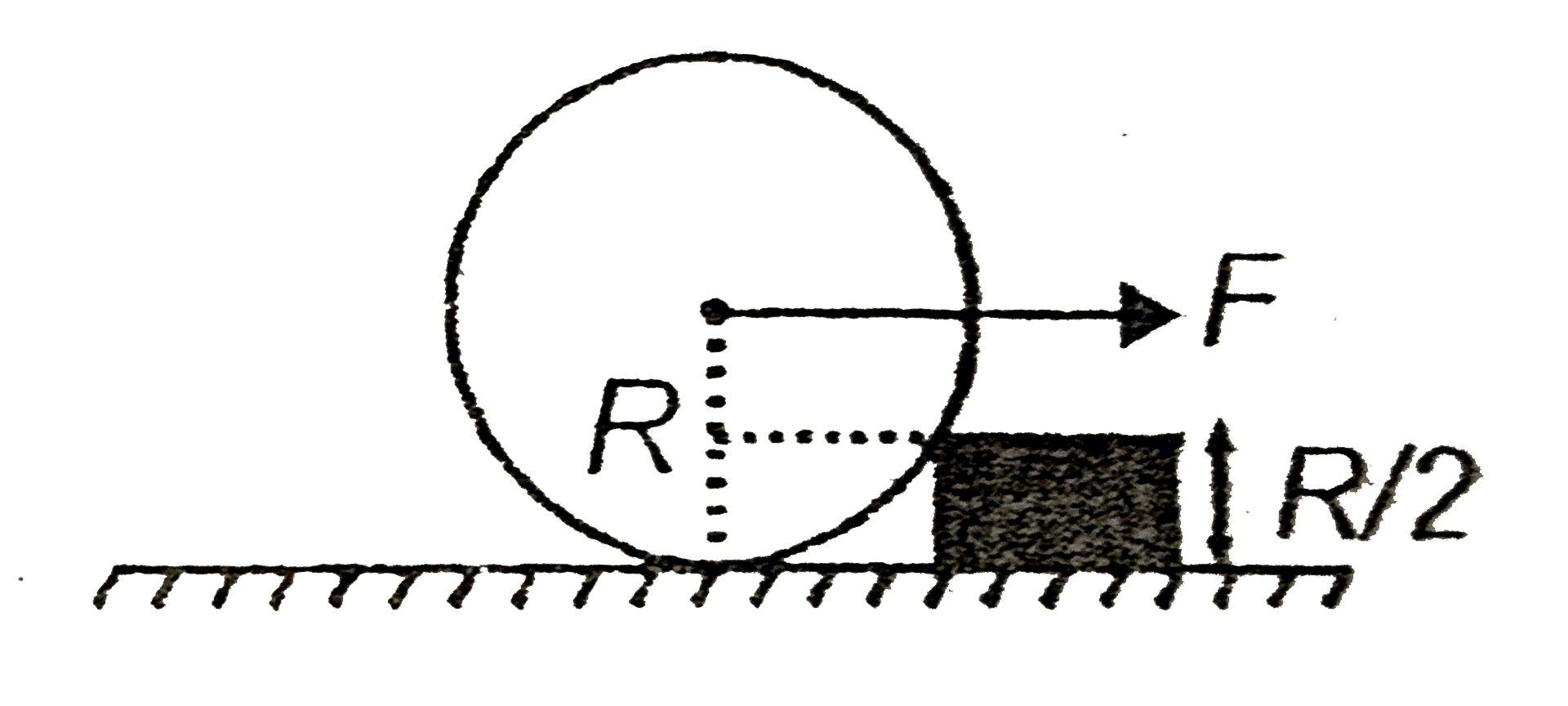
Calculate the force $F$ that is applied horizontally at the axle of the wheel which is necessary to raise the wheel over the obstacle of height $0.4 m$ . Radius of wheel is $1 m$ and $m a s s = 10 k g$ . $F$ is

$100 N$

$66 N$

$167 N$

$133.3 N$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:D

Updated on:21/07/2023

The angular velocity of a wheel is $70 r a d / sec$ . If the radius of the wheel is 0.5 m , then linear velocity of the wheel is
A $70 m / s$
B $35 m / s$
C $30 m / s$
D $20 m / s$
The moment of inertia of a solid flywheel about its axis is $0.1 k g - m^{2}$ .A tangential force of 2 kg-wt. is applied round the circumference of the flywheel with the help of a string and mass arrangement as shown in the figure. If the radius of the wheel is 0.1 m, find the acceleration $({in rad/sec}^{2})$ of the mass :

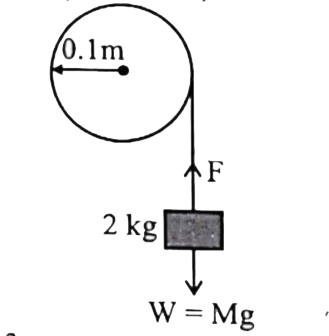A163.3
B16.3
C81.66
D8.16
The M.I. of a wheel of mass 8 kg and radius of gyration 25 cm is
A $5 k g m^{2}$
B $1.5 k g m^{2}$
C $2.5 k g m^{2}$
D $0.5 k g m^{2}$