A balance is made of a rigid rod free to rotate about a point not at the centre of the rod. When an unknown mass m is placed in the left pan, it is balanced by a mass m1 placed in the right pan and similarly when the mass m is placed in the right pan, it is balanced by a mass m2 in the left pan. Neglecting the masses of the pans, m is
m1+m22
√m1m2
√m21+m222
√(m21+m22)2
The correct Answer is:B
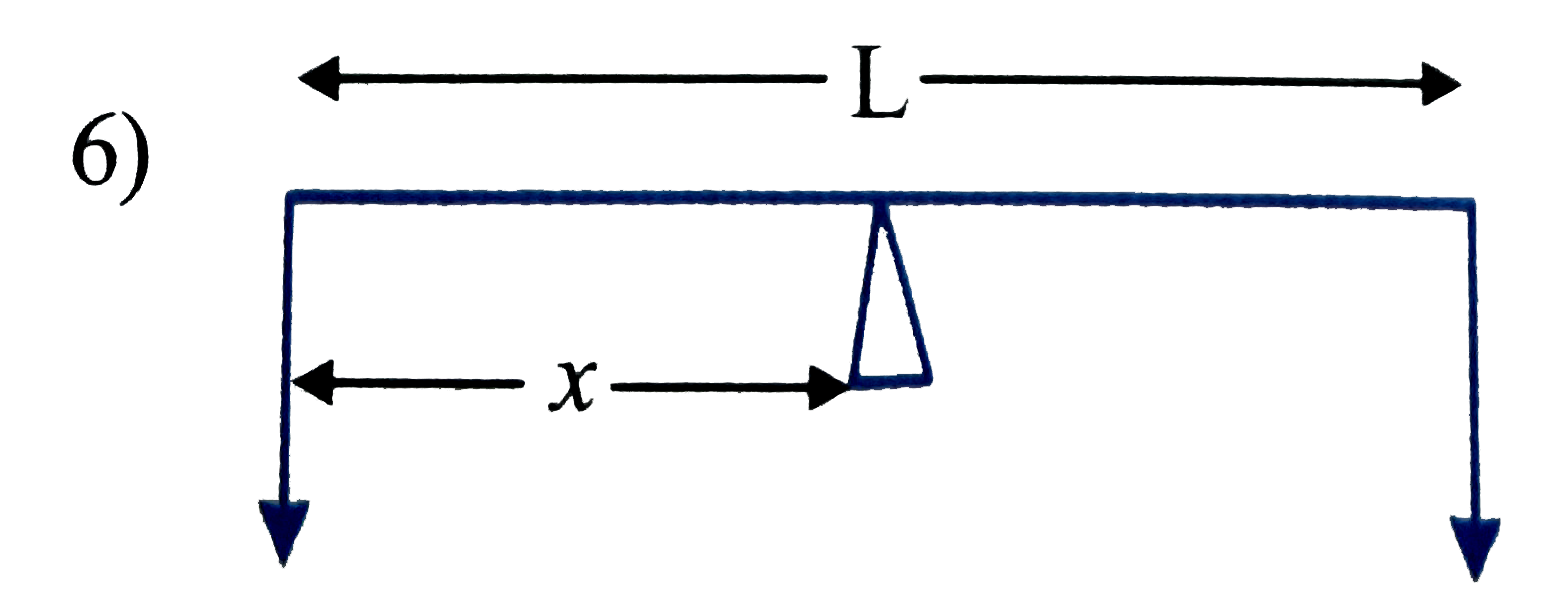
Let L be the length of the rod and knife edge is placed at a distance x from the left pan
About the knife edge
mg(x)=m1g(L−x)………..(1)
m2g(x)=mg(L−x)………..(2)
dividing (1) by (2)
mm2=m1m⇒m=√m1m2
Topper's Solved these Questions
- BOOK - NARAYNACHAPTER - EXPERIMENTAL PHYSICSEXERCISE - Level-v(Single answer)23videos
EXPERIMENTAL PHYSICS
- BOOK - NARAYNACHAPTER - EXPERIMENTAL PHYSICSEXERCISE - Multiple answer6videos
EXPERIMENTAL PHYSICS
- BOOK - NARAYNACHAPTER - ELECTROSTATICS AND GAUSS LAWEXERCISE - Intergers type question11videos
ELECTROSTATICS AND GAUSS LAW
- BOOK - NARAYNACHAPTER - MAGNETISMEXERCISE - LEVEL-II (H.W)24videos
MAGNETISM
Similar Questions
In a experiment it was found that the string vibrated in three loops when 8 g were placed on the scale pan. What must be placed on the pan to make the string vibrate in six loops? Neglect the mass of the string and the scale pan.
View SolutionAt wood machine is attached below the left hand pan of a physical balance while equal weight (M+m) g is placed in the right hand pan. The beam is horizontal when the masses M and m on the left hand side are braked What will happen if the masses M and m are unbraked on the left hand side?
View Solution
Knowledge Check
- Question 1 - Select One
A body weighs 6gms when placed in one pan and 24gms when placed on the other pan of a false balance. If the beam is horizontal when both the pans are empty, the true weight of the body is :
A13 gmB12 gmC15.5 gmD15 gm - Question 2 - Select One
in an experiment it was found that string vibrates in n loops when a mass M is placed on the pan. What mass should be placed on the pan to make it vibrate in 2n loops with same frequency ? ( neglect the mass of pan )
A2MBM4C4MDM2 - Question 3 - Select One
In Melde's experiment in parallel position the mass of the pan is M0. When a mass m1 is kept in the pan, the number of loops formed is p1. For the mass m2, the number of loops, formed is p2. Then the mass of the pan M0, in terms of m1,m2,p1 and p2 is given by
Am0=p21−p22m2p22−m1p21Bm0=m2p22−m1p21p21−p22Cm0=m2p22+m1p21p21−p22Dm0=m2p22−m1p21p21+p22
Find the gravitatinal force of between a point mass m placed at a disance x on the prolongation of a thin rod of mass M andlength l from near end .
View SolutionA string vibrates in three loops when 8gram weight is placed in the pan. What mass should be kept in the pan to make it vibrate in six loops. Neglect the mass of string and mass of pan.
View SolutionIn an experiment with a beam balance, an unknown mass m is balanced by two known masses of 16kg and 4kg shown in Fig. The value of the unknown mass m is
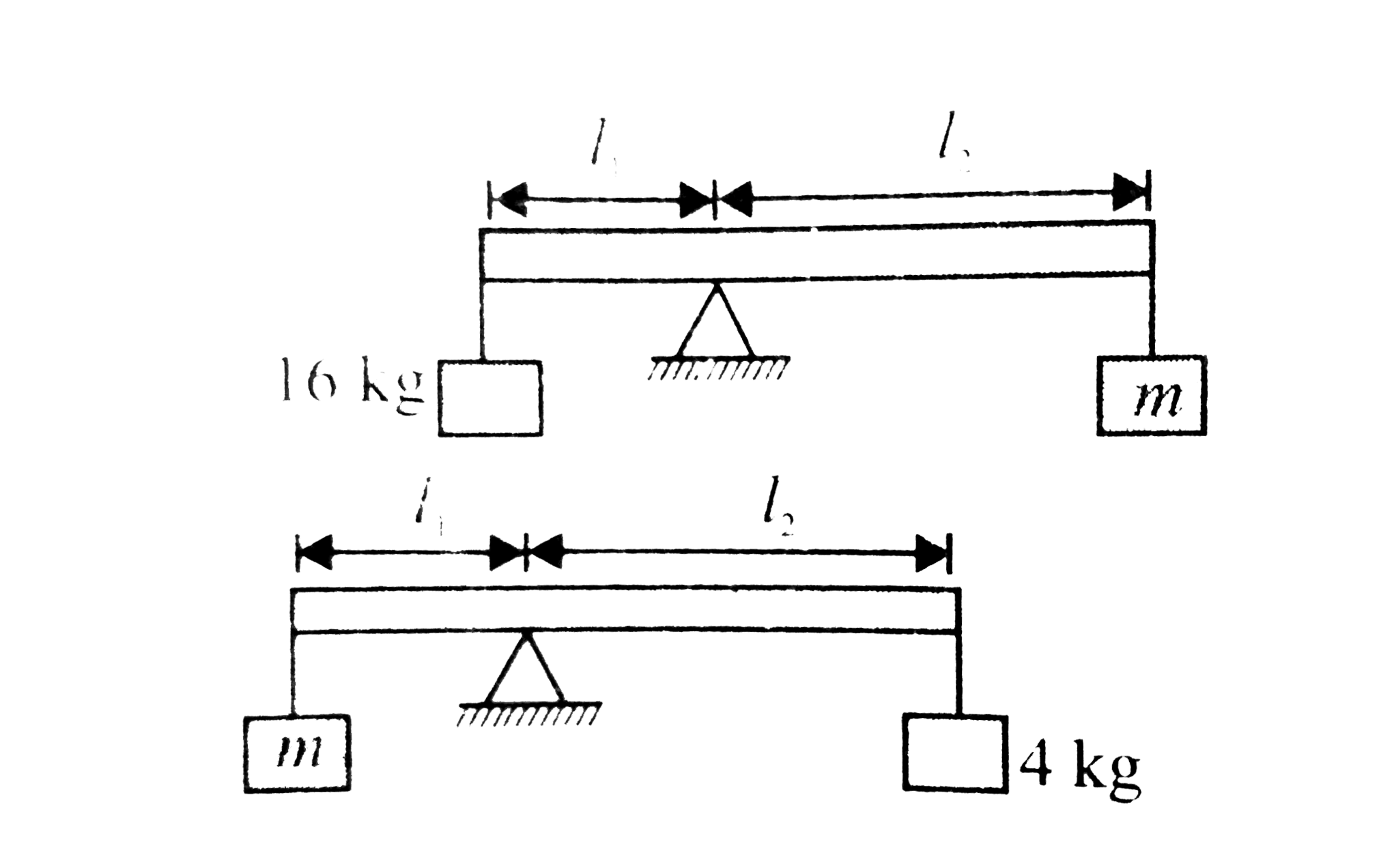 View Solution
View SolutionThe figure shows a spherical shell of mass M. The point a is not at the centre but away from the centre of the shell. If a particle of mass m is placed at A, then
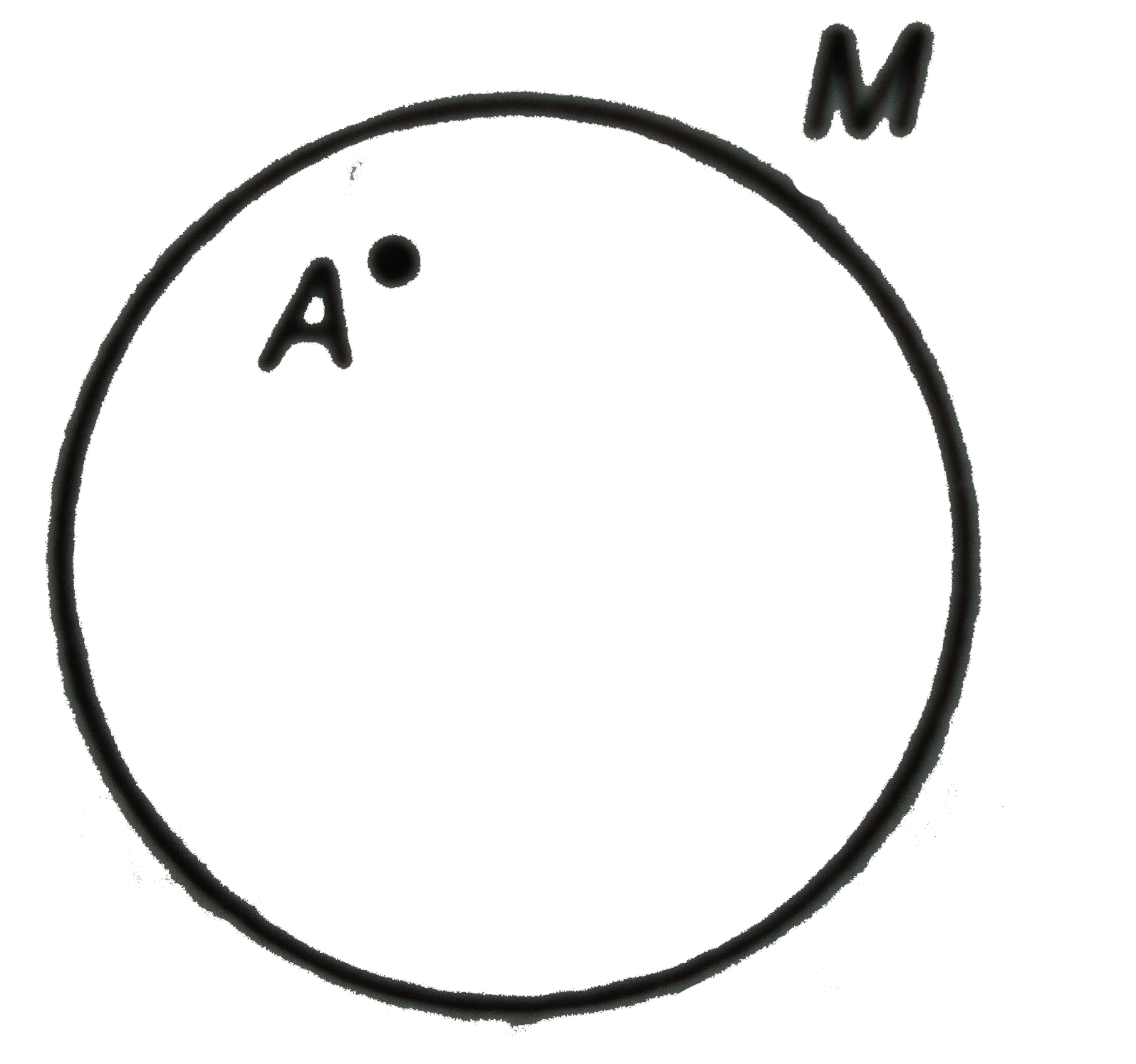 View Solution
View SolutionThe beam and pans of a balance have negligible mass. An object weight W1 when placed in one pan and W2 when placed in the other pan. The weights W of the object is.
View Solution

