A particle is thrown with speed u at an angle of projection θ with horizontal as shown.

(i) The avergae velocity of particle from O to A is ucosθ
(ii) The average velocity of particle from O to H (highest point) is u2√1+3cos2θ
(iii) The average velocity of particle from J to K is ucosθ
(iv) The angle between the velocity and the acceleration between O and H is greater than 90∘, between H to A is less than 90∘ and at highest point is 90∘
(i), (ii)
(i), (iii)
(ii), (iv)
all option are correct
The correct Answer is:D
(i) O to A: ¯¯¯vy=0,¯¯¯¯vx=ucosθ,¯v=ucosθ
(ii) 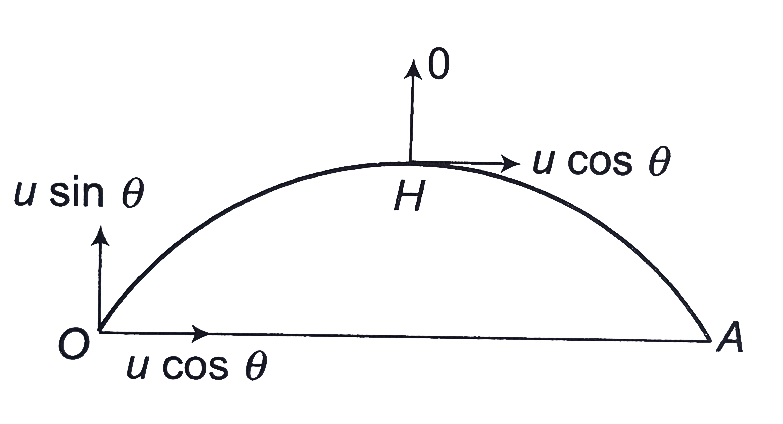
¯¯¯¯vx=ucosθ,vy=usinθ+02=usinθ2
¯v=√¯¯¯¯vx2+¯¯¯vy2=√(ucos2θ)2+(usinθ2)2
=u2√4cos2θ+sin2θ=u2√1+3cos2θ
(iii) J to K: →vy=0,¯¯¯¯vx=ucosθ,¯v=ucosθ
(iv) 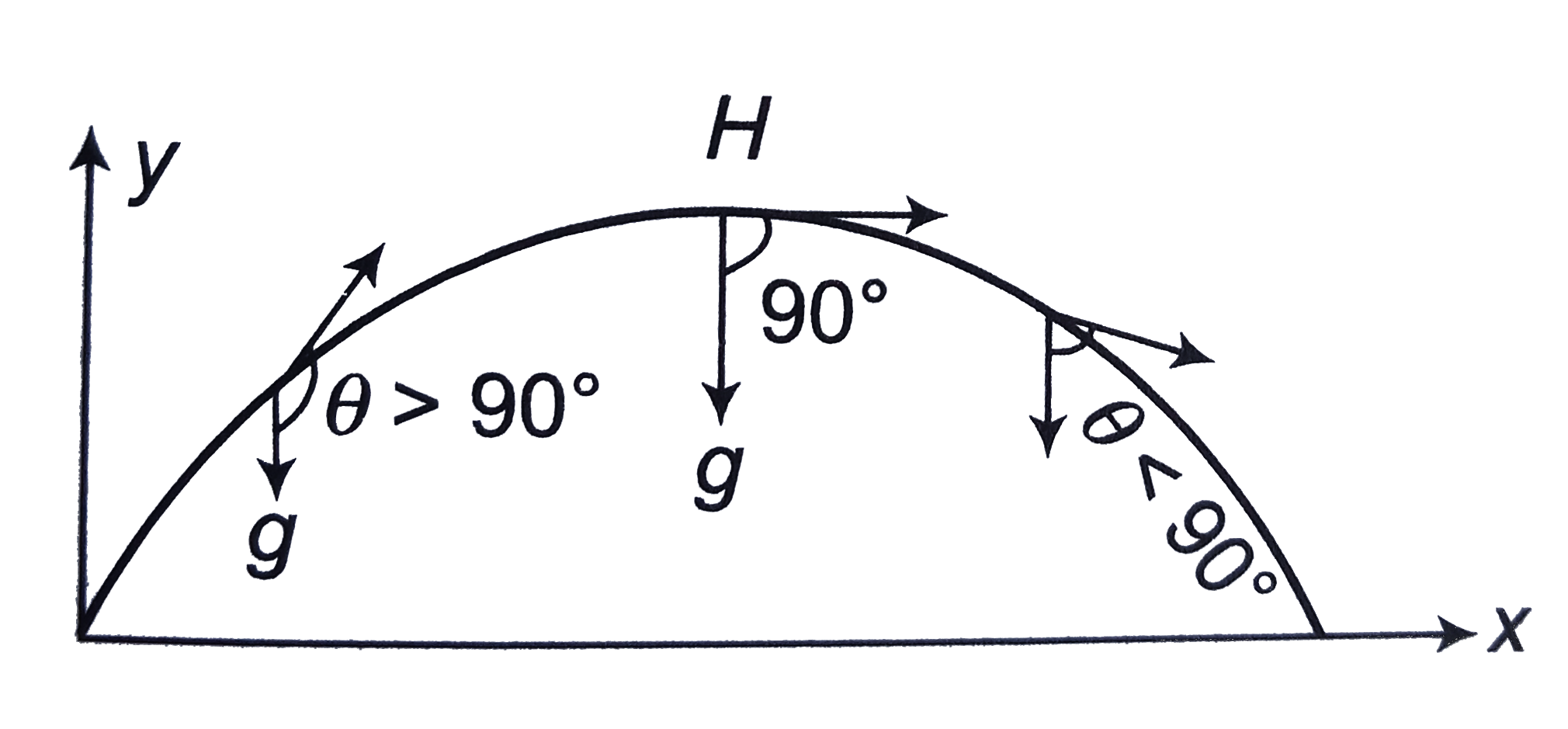
θ: angle between velocity and acceleration.
Topper's Solved these Questions
Similar Questions
A particle is thrown with speed u at an angle θ with horizontal.
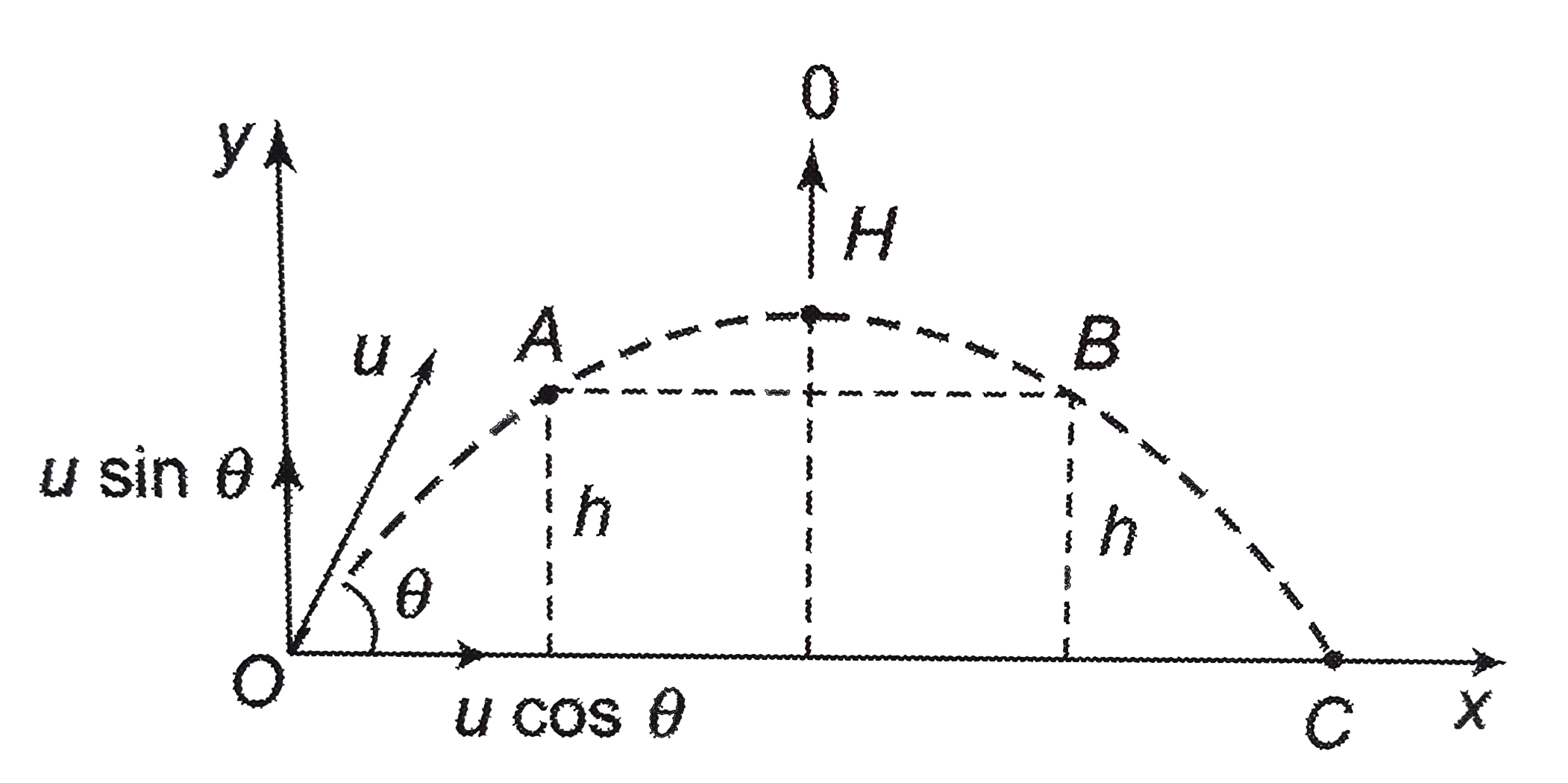
Find the average velocity of particle:
(a) O to C (b) A to B ( c) O to HView SolutionA particle is thrown with speed u at an angle α with horizontal from the ground. After how much time, the velocity of particle will make an angle β with horizontal.
View Solution
Knowledge Check
- Question 1 - Select One
A particle of mass m is thrown with speed u at an angle of projection θ with horizontal. The average power imparted by the gravitational force until it strikes the ground is
AzeroBmgHmaxTCmgHmax2TD2mgHmaxT - Question 2 - Select One
A particle is thrown with a speed u at an angle θ with horizontal. After how much time the velocity of particle will be perpendicular to the initial motion of direction
AugcosθBugsinθCusinθgDucosθg - Question 3 - Select One
A particle is projected with velocity v at an angle of θ with horizontal. The average angular velocity of the particle from the point of projection to impact equals:
AgcosθθvBgvsinθCgvθDgθvsinθ
A particle of mass m is projected with a speed u at an angle θ with the horizontal. Find angular momentum of particle after time t about point of projection.
View SolutionA particle is projected with velocity v at an angle θ aith horizontal. The average angle velocity of the particle from the point of projection to impact equals
View SolutionA particle is fired from a point on the ground with speed u making an angle θ with the horizontal.Then
View SolutionA projectile is projected with speed u at an angle θ with the horizontal . The average velocity of the projectile between the instants it crosses the same level is
View SolutionA particle is fired with velocity u making angle θ with the horizontal.What is the change in velocity when it is at the highest point?
View Solution

