A bird of mass m is flying horizontally with speed v0 suddenly strikes a vertical rod of mass 3m and length L hinged at its base at distance L/3 from the top of the rod. After collision the bird drops to the ground (but soon recovers to fly happly away). What is the angular velocity of the rod
(a) just after it is hit by the bird and
(b) just as it reaches the ground?
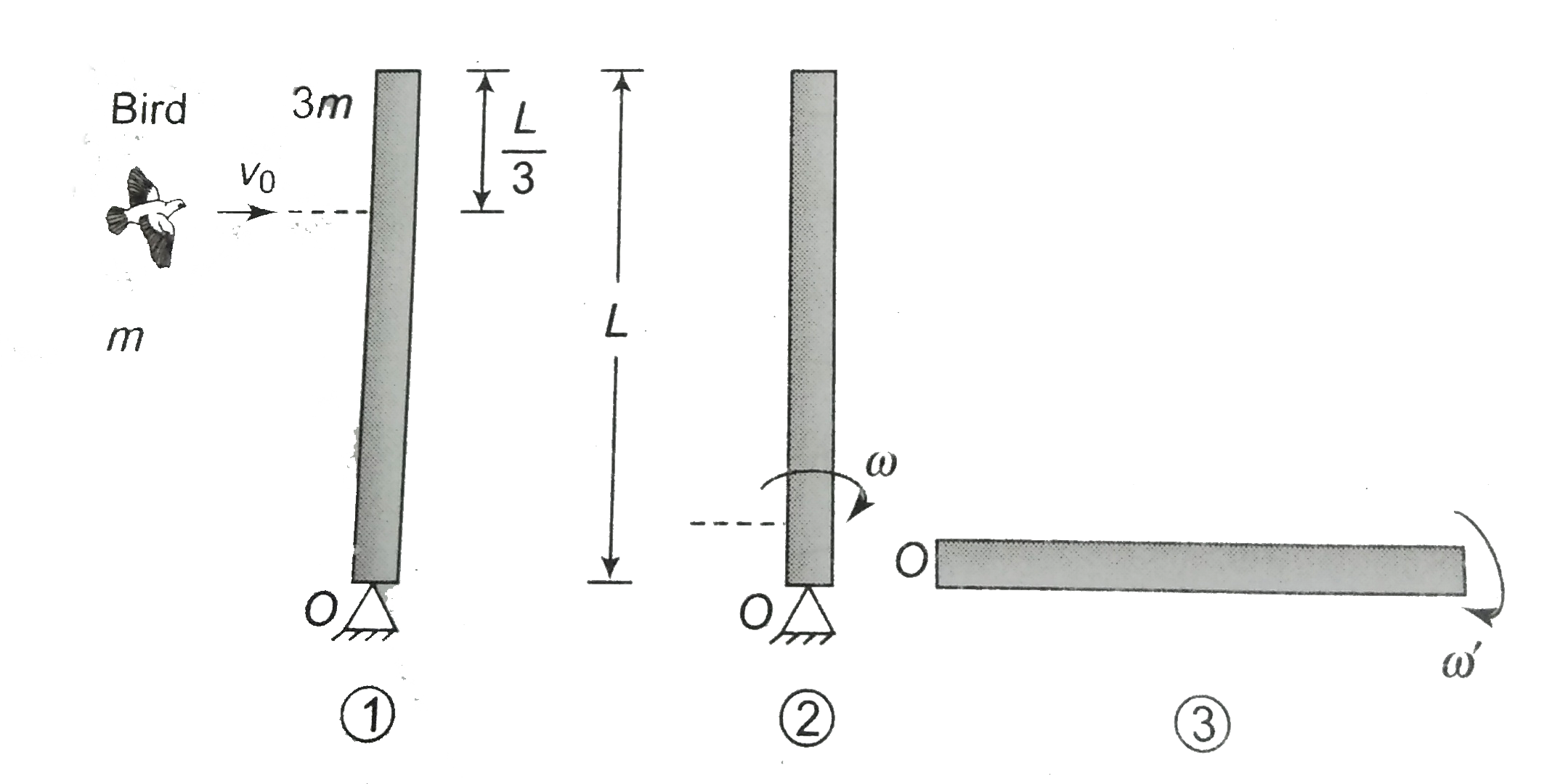
Applying the conservation on angular momentum about O
mv02L3=I0ω=3mL23ω
ω=2v03L
Applying the energy conservation between (2) and (3)
3mg(L2)+12I0ω2=12I0ω'2
3mgL2+12I0(ω'2−ω2)=123mL23(ω'2−ω2)
ω'=√ω2+3gL=√4v209L2+3gL
Topper's Solved these Questions
Similar Questions
A uniform rod of mass M and length a lies on a smooth horizontal plane. A particle of mass m moving at a speed v perpendicular to the length of the rod strikes it at a distance a4 from the centre and stops after the collision. Find a. the velocity of the cente of the rod and b. the angular velocity of the rod abut its centre just after the collision.
View SolutionA uniform rod of mass m and length l is pivoted smoothly at O. A horizontal force acts at the bottom of the rod.
a. Find the angular velocity of the rod as the function of angle of rotation θ.
b.What is the maximum angular displacement of the rod?
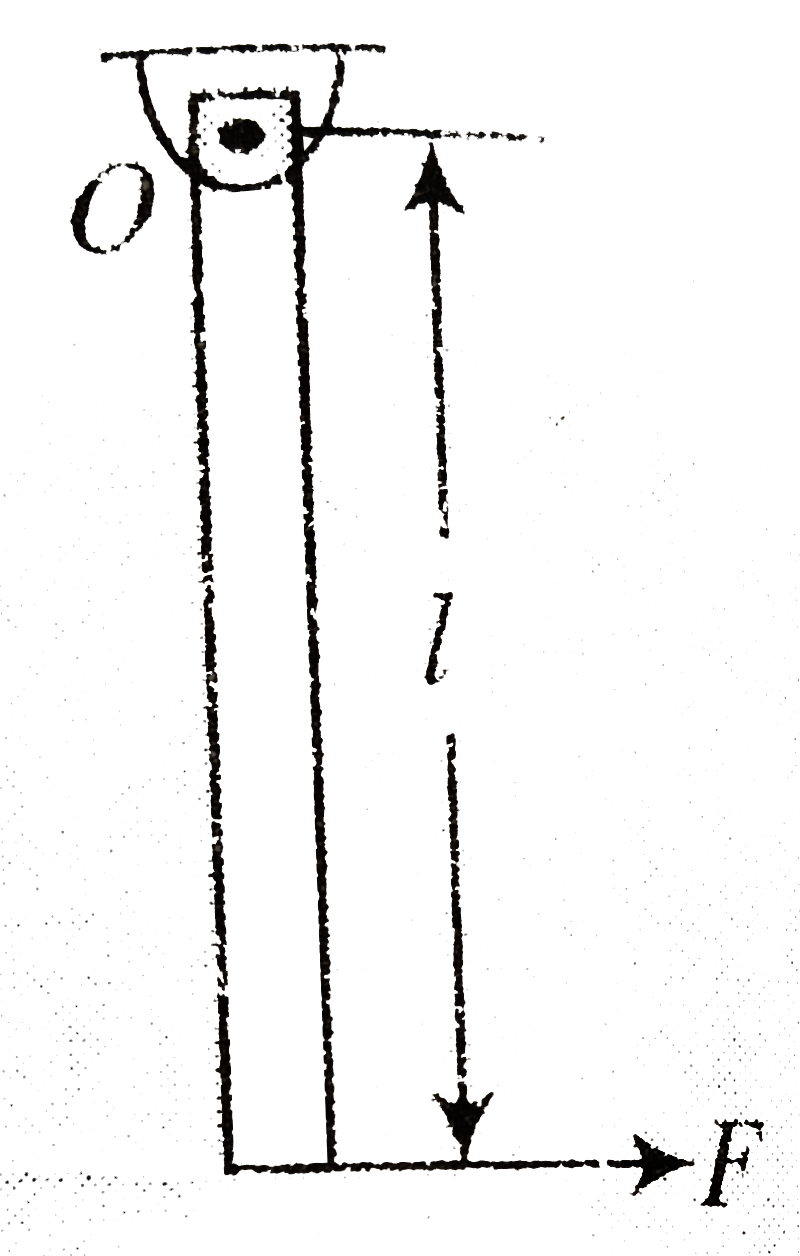 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A uniform thin rod of length l and mass m is hinged at a distance l/4 from one of the end and released from horizontal position as shown in Fig. The angular velocity of the rod as it passes the vertical position is
 A2√5g71B2√6g71C√3g71D2√21
A2√5g71B2√6g71C√3g71D2√21 - Question 2 - Select One
A rod of mass 4m and length L is hinged at the mid point. A ball of mass 'm' moving with speed V in the plane of rod, strikes at the end at an angle of 45∘ and sticks to it. The angular velocity of system after collision is-
A3√2V7LB√2V7LC√2V3LD3V7L - Question 3 - Select One
A uniform thin rod of length l and mass m is hinged at a distance l/4 from one of the end and released from horizontal position as shown in Fig. The angular velocity of the rod as it passes the vertical position is
 A2√5g7lB2√6g7lC√3g7lD2√gl
A2√5g7lB2√6g7lC√3g7lD2√gl
A uniform rod of mass M and length L lies on a smooth horizontal plane. A particle of mass m moving at a speed v perpendicular to the length of the rod strikes it at a distance L/6 from the center and stops after the collision. Find
(a) the velocity of center of mass of rod and
(b) the angular velocity of the rod about its center just after collision.View SolutionA rod AB of mass M and length L is shown in figure. End A of rod is hinged and end B is lying on the ground. Find the
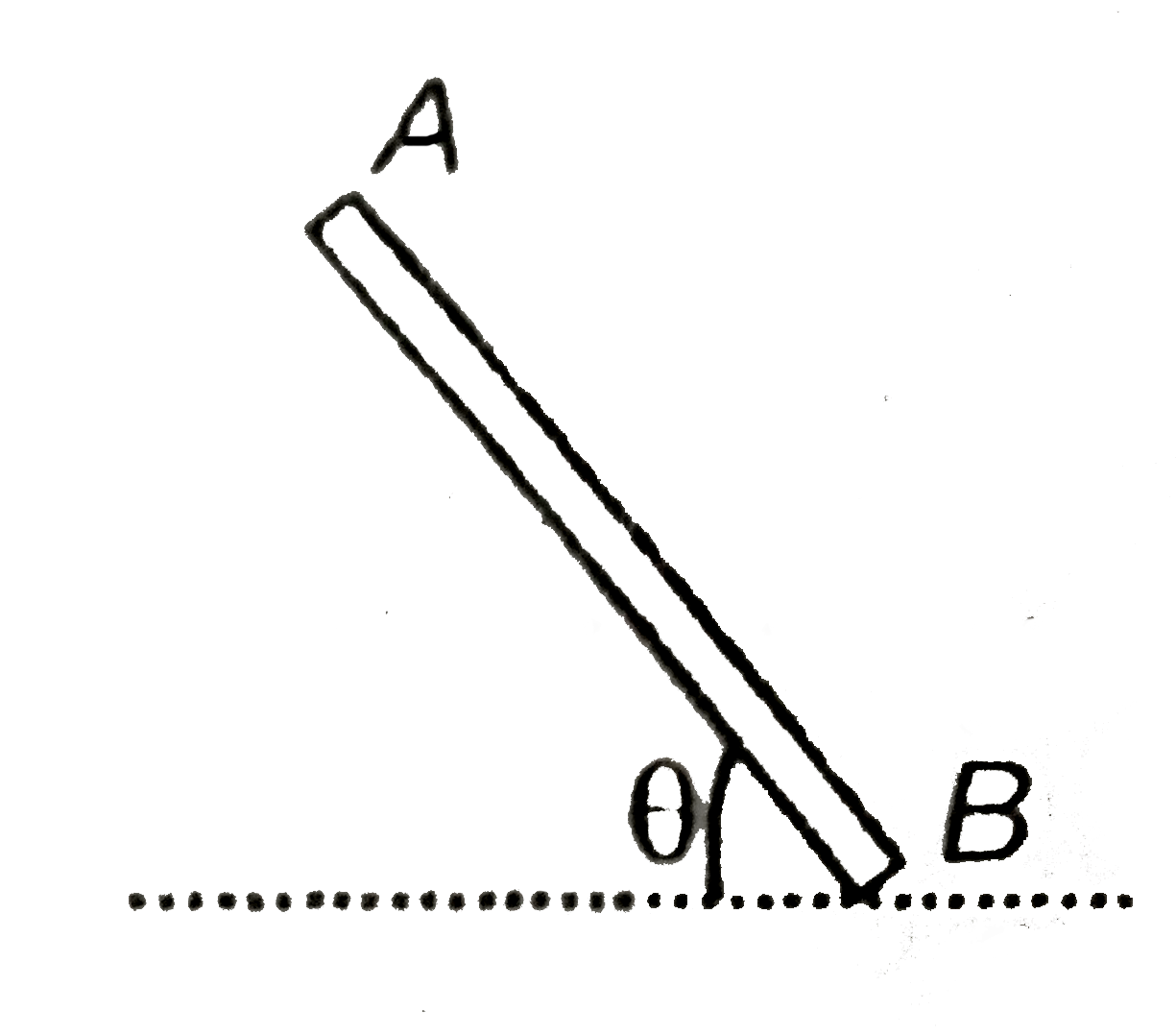
Normal reaction by ground on the rodView SolutionA rod of length L is hinged from one end. It is brought to horizontal position and released. The angular velocity of the rod when it is in vertical position. Is
View SolutionA rod of length L is hinged from one end. It is brought to a horizontal position and released. The angular velocity of the rod, when it is in vertical position, is
View SolutionConsider a rod of mass M and length L pivoted at its centre is free to rotate in a vertical position plane. The rod is at rest in the vertical position. A bullet of mass M moving horizontally at a speed v strikes and embedded in one end of the rod. The angular velocity of the rod just after the collision will be.
View Solution

