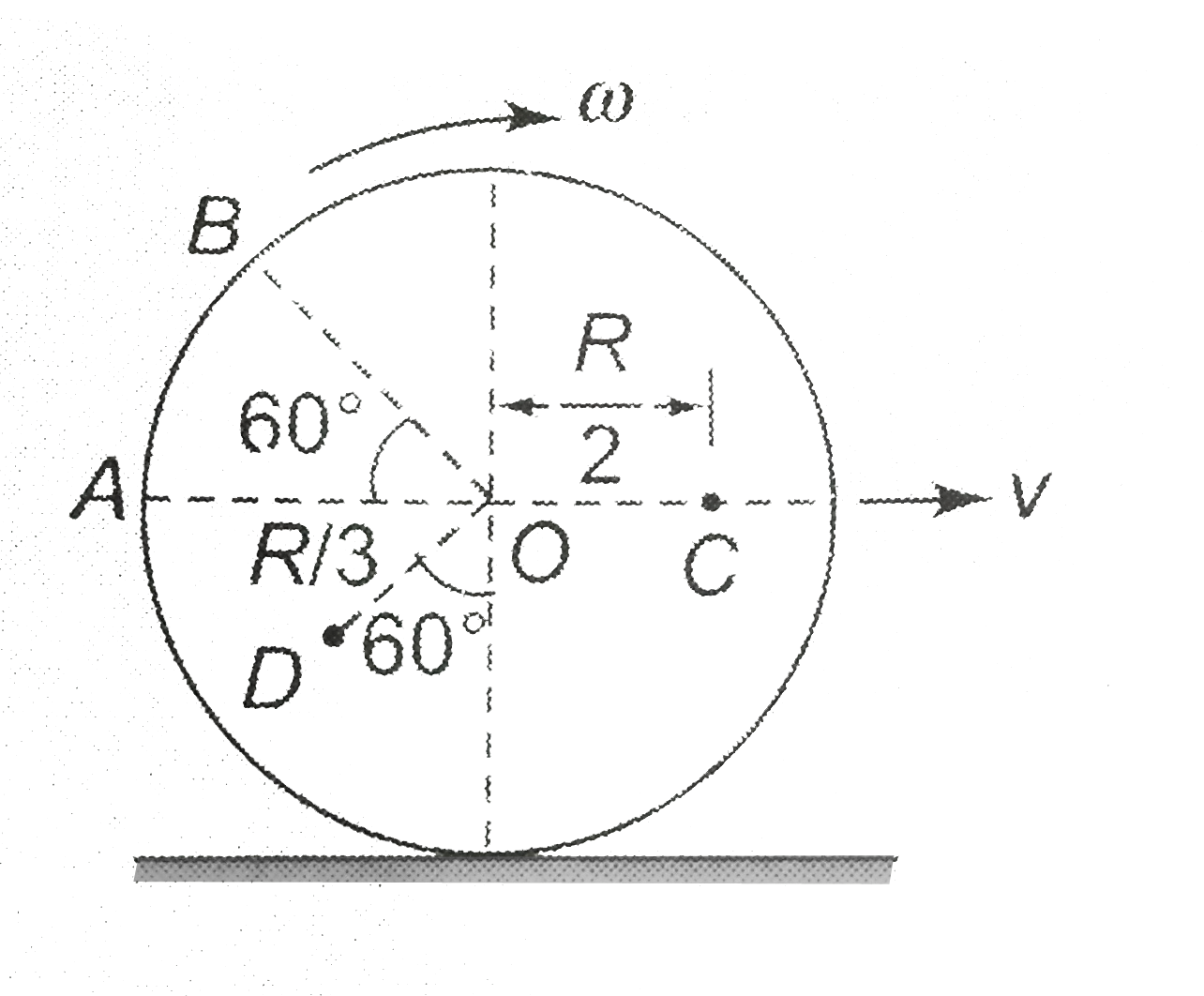A hollow sphere rolls without slipping the on the horizontal surface such that its translational velocity is v. Find that the maximum height attained by it on an inclined surface.
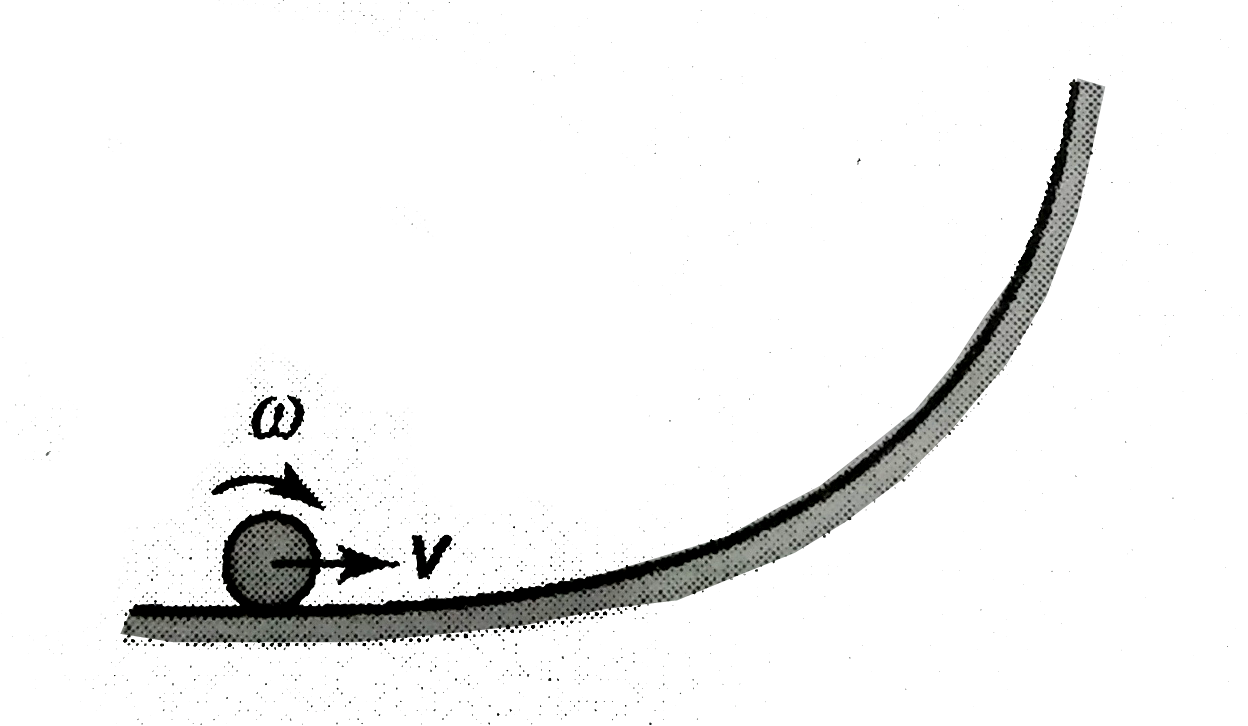
In case of pure rolling, energy is conserved, v=rω. Applying the energy conservation between positions (1) and (2)

K1+U1=K2+U2
12mv2c.m.+12Ic.m.ω2=0+mgh
12mv2+12×23mr2(vr)2=mgh
56mv2=mgh
h=5v26g
OR
12mv2c.m.(1+k2R2)=mgh
(K2R2=23for hollow sphere)
12mv2(1+23)=mgh⇒h=5v26g
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
A wheel rolls without slipping on a horizontal surface such that its velocity of center of mass is v. The velocity of a particle at the highest point of the rim is
AzeroBvC2vD√2v - Question 2 - Select One
A solid sphere of mass m and radius R rolls without slipping on a horizontal surface such that vc.m.=v0.
AThe kinetic energy of rotation is 15mv20.BThe total kinetic energy is 710mv20.CThe mechanical energy (assume the ground as reference) is mgR+710mv20.DAll options are correct. - Question 3 - Select One
A solid sphere rolls without slipping along a horizontal surface. What percentage of its total kinetic energy is rotational kinetic energy?
A0.33B0.29C0.5D0.75
A hollow sphere is rolling without slipping on a rough surface. The ratio of translational kinetic energy to rotational kinetic energy is
View SolutionA solid sphere of mass m and radius R rolls without slipping on the horizontal surface such that its velocity of center of mass is v0. Find its translational kinetic energy, rotational kinetic energy, total kinetic energy, gravitational potential energy taking surface as reference level and mechanical energy.
View SolutionA solid sphere is rolling without slipping on a horizontal plane. The ratio of its rotational kinetic energy and translational kinetic energy is
View Solution
A uniform circular righ rolls without slipping on a horizontal surface. At any instant, its position is as shown in the figure. ThenView SolutionWhen a hollow sphere is rolling without slipping on a rough horizontal surface then the percentage of its total K.E. which is translational is
View Solution