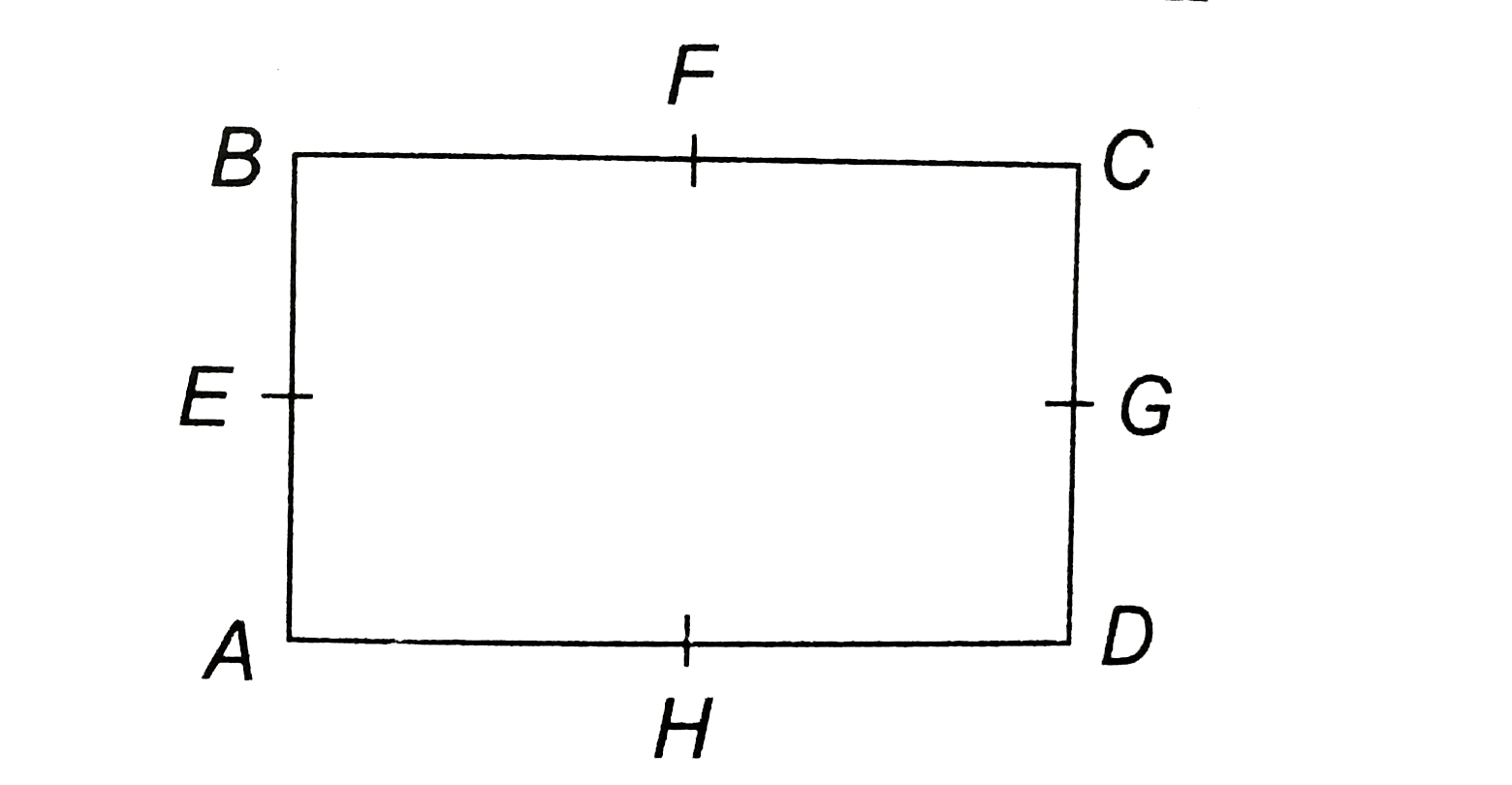
In a rectangle $A B C D$ ( $B C = 2 A B$ ). The moment of inertia along which the axis will be minimum

$B C$

$B D$

$H F$

$E G$

Video Solution

Text Solution

Verified by Experts

The moment of inertia will be minimum about that axis, which is passing through the center of mass.

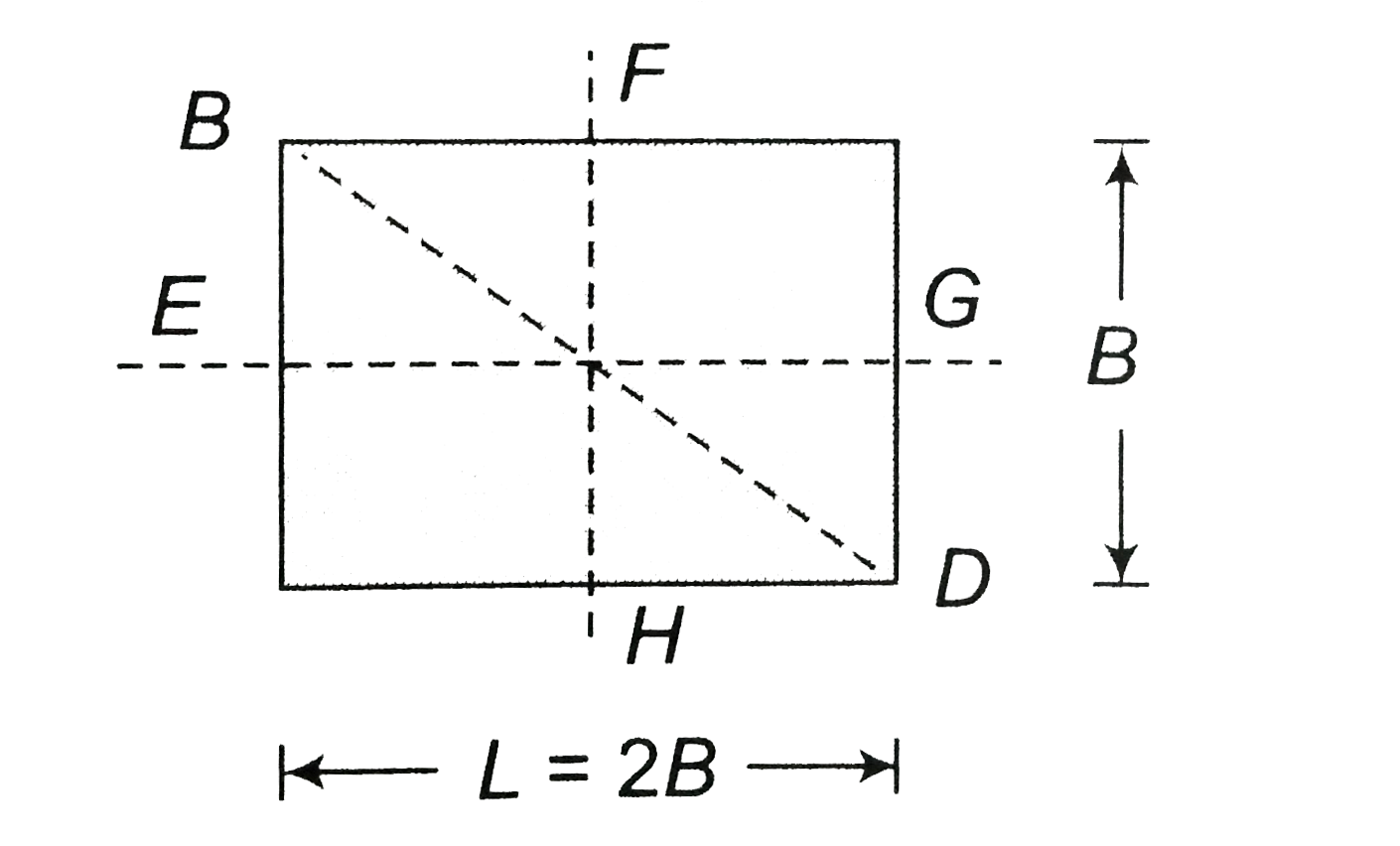
$I_{E G} = \frac{M B^{2}}{12}$
$I_{F H} = \frac{M {(2 B)}^{2}}{12} = \frac{M B^{2}}{6}$
$M . I .$ of the rectangular plate about its diagonal
$I_{B D} = \frac{M B^{2} L^{2}}{6 (B^{2} + L^{2})} = \frac{M B^{2} \times 4 B^{2}}{6 (B^{2} + 4 B^{2})} = \frac{2}{15} M B^{2} = \frac{M B^{2}}{7.5}$
$M . I .$ about $E G$ is minimum.
OR
By observation, distribution of mass is the nearest about axis $E G$ .

Updated on:21/07/2023