A circular disc of radius 20cm is cut from one edge of a larger circular disc of radius 50cm. The shift of centre of mass is
5.7cm
−5.7cm
3.2cm
−3.2cm
The correct Answer is:B
To solve the problem of the shift of the center of mass when a smaller circular disc is cut from a larger circular disc, we can follow these steps:
1. Identify the Parameters:
- Radius of the larger disc, R1=50cm=0.5m
- Radius of the smaller disc, R2=20cm=0.2m
- The mass of the larger disc can be expressed in terms of its density and area.
2. Calculate the Area and Mass of the Discs:
- Area of the larger disc, A1=πR21=π(0.5)2=π4m2
- Area of the smaller disc, A2=πR22=π(0.2)2=π25m2
3. Assume the Density:
- Let the density of the material be ρ.
- Mass of the larger disc, m1=ρA1=ρ⋅π4
- Mass of the smaller disc, m2=ρA2=ρ⋅π25
4. Calculate the Masses:
- The mass of the larger disc is m1=πρ4
- The mass of the smaller disc is m2=πρ25
5. Use the Concept of Negative Mass:
- When the smaller disc is cut out, we can consider it as placing a negative mass −m2 at its center.
- The center of the larger disc is at the origin (0,0), and the center of the smaller disc is at (30 cm, 0) or (0.3 m, 0).
6. Calculate the Center of Mass:
- The x-coordinate of the center of mass xcm is given by:
xcm=m1⋅x1+m2⋅x2m1+m2
- Here, x1=0 (for the larger disc) and x2=0.3 m (for the smaller disc).
- Substitute the values:
xcm=m1⋅0+(−m2)⋅0.3m1−m2
- Substitute m1 and m2:
xcm=0−(πρ25)⋅0.3πρ4−πρ25
7. Simplify the Expression:
- The denominator becomes:
πρ4−πρ25=πρ(25−4100)=21πρ100
- The numerator becomes:
−πρ25⋅0.3=−0.3πρ25
- Thus,
xcm=−0.3πρ2521πρ100=−0.3⋅10025⋅21=−3021 m=−1.42857 m
8. Convert to Centimeters:
- Converting to centimeters gives:
xcm=−142.857 cm≈−5.7 cm
9. Conclusion:
- The center of mass shifts approximately 5.7cm towards the left (negative x-direction).
Final Answer:
The shift of the center of mass is approximately −5.7cm.
- Radius of the larger disc, R1=50cm=0.5m
- Radius of the smaller disc, R2=20cm=0.2m
- The mass of the larger disc can be expressed in terms of its density and area.
- Area of the larger disc, A1=πR21=π(0.5)2=π4m2
- Area of the smaller disc, A2=πR22=π(0.2)2=π25m2
- Let the density of the material be ρ.
- Mass of the larger disc, m1=ρA1=ρ⋅π4
- Mass of the smaller disc, m2=ρA2=ρ⋅π25
- The mass of the larger disc is m1=πρ4
- The mass of the smaller disc is m2=πρ25
- When the smaller disc is cut out, we can consider it as placing a negative mass −m2 at its center.
- The center of the larger disc is at the origin (0,0), and the center of the smaller disc is at (30 cm, 0) or (0.3 m, 0).
- The x-coordinate of the center of mass xcm is given by:
xcm=m1⋅x1+m2⋅x2m1+m2
- Here, x1=0 (for the larger disc) and x2=0.3 m (for the smaller disc).
- Substitute the values:
xcm=m1⋅0+(−m2)⋅0.3m1−m2
- Substitute m1 and m2:
xcm=0−(πρ25)⋅0.3πρ4−πρ25
- The denominator becomes:
πρ4−πρ25=πρ(25−4100)=21πρ100
- The numerator becomes:
−πρ25⋅0.3=−0.3πρ25
- Thus,
xcm=−0.3πρ2521πρ100=−0.3⋅10025⋅21=−3021 m=−1.42857 m
- Converting to centimeters gives:
xcm=−142.857 cm≈−5.7 cm
- The center of mass shifts approximately 5.7cm towards the left (negative x-direction).
The shift of the center of mass is approximately −5.7cm.
Topper's Solved these Questions
- BOOK - NARAYNACHAPTER - SYSTEM OF PARTICLESEXERCISE - Level -II(H.W)47videos
SYSTEM OF PARTICLES
- BOOK - NARAYNACHAPTER - SYSTEM OF PARTICLESEXERCISE - Level-V72videos
SYSTEM OF PARTICLES
- BOOK - NARAYNACHAPTER - SYSTEM OF PARTICLESEXERCISE - NCERT based questions15videos
SYSTEM OF PARTICLES
- BOOK - NARAYNACHAPTER - PHYSICAL WORLDEXERCISE - C.U.Q10videos
PHYSICAL WORLD
- BOOK - NARAYNACHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTION EXERCISE - EXERCISE - IV39videos
SYSTEM OF PARTICLES AND ROTATIONAL MOTION
Similar Questions
A circular disc of radius R4 is cut from a uniform circular disc of radius R.
The centre of cut portion is at a distance of R2 from the centre of the disc from which it is removed. Calculate the centre of mass of remaining portion of the disc.View SolutionA circular hole of radius R/2 is cut from a circular disc of radius R. The disc lies in the xy-plane and its centre coincides with the origin. If the remaining mass of the disc is M, then
a. determine the initial mass of the disc and
b. determine its moment of inertia about the z-axis.
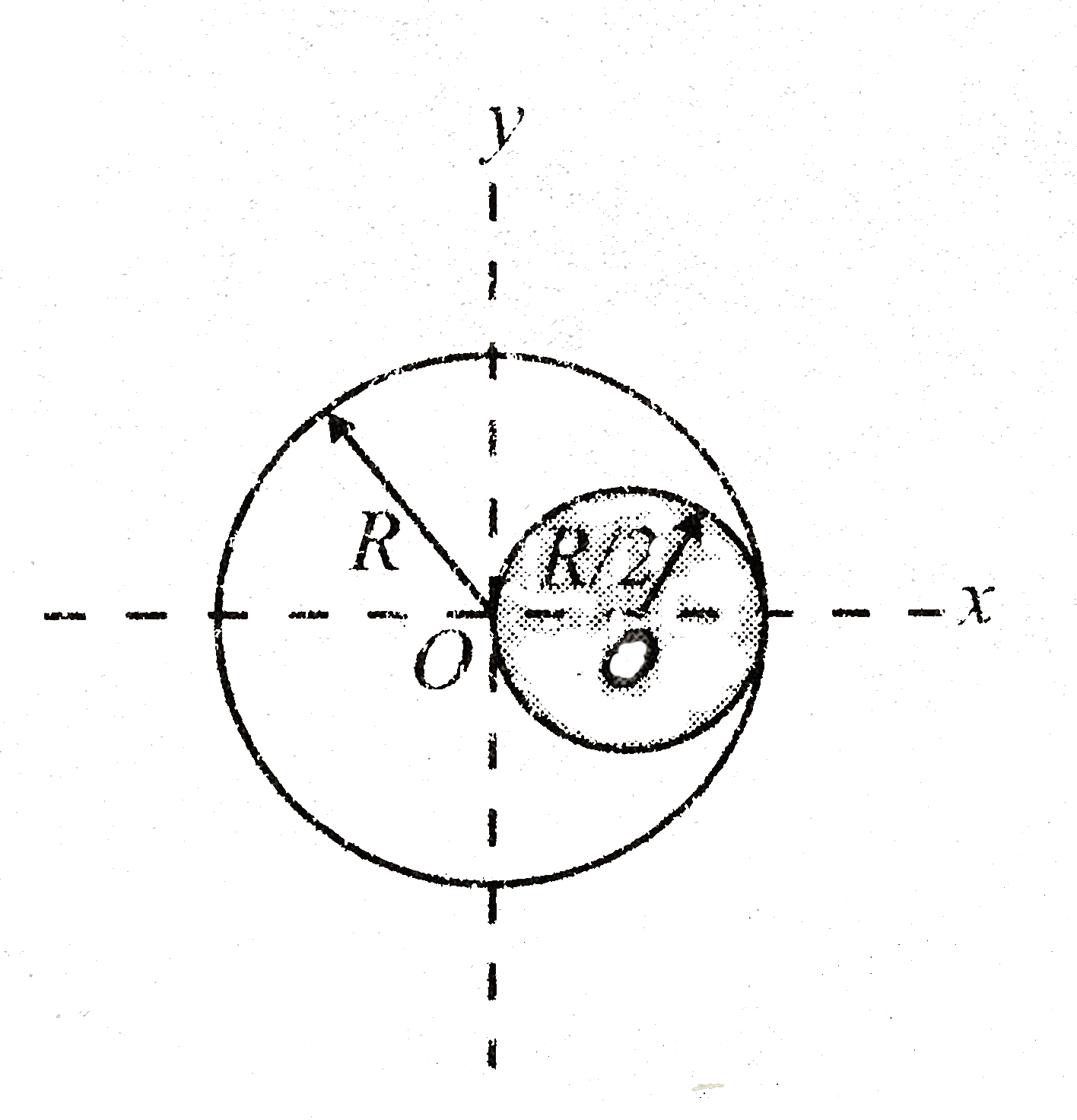 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A circular disc of radius R is removed from a bigger circular disc of radius 2R such that the circumference of the discs coincoid . The centre of mass of the new disc is αR from the centre of the bigger disc . the value of α is
A1/3B1/2C1/6D1/4 - Question 2 - Select One
A circular disc of radius R is removed from a bigger circular disc of radius 2R such that the cirucmferences of the discs coincide. The centre of mass of the new disc is αR from the center of the bigger disc. The value of α is
A14B13C12D16 - Question 3 - Select One
A circular disc of radius R is removed from a bigger circular disc of radius 2R, such that the circumference of the discs coincide. The centre of the bigger disc. The value of α is
A13B12C16D14
A circular disc of radius R is removed from a bigger circular disc of radius 2R, such that the circumference of the discs coincide. The centre of the bigger disc. The value of α is
View SolutionA circular disc of radius R is removed from a bigger circular disc of radius 2R such that the circumferences of the discs coincide. The center of mass of new disc is αR from the center of the bigger disc. The value of α is
View SolutionA circular disc of radius R is removed from a bigger circular disc of radius 2R such that the circumference of the discs coincoid . The centre of mass of the new disc is αR from the centre of the bigger disc . the value of α is
View SolutionA solid toy is in the form of a hemisphere surmounted by a right circular cone of same radius. The height of the cone is 10 Cm and the radius of the base is 7 cm. Determine the volume of the toy. Also find the area of the coloured sheet required to cover the toy. (Use π=22/7 and √149=12.2)
View SolutionA circular disc of radius R is removed from a bigger circular dise of radius such that the circumferences of the discs coincide. The centre of mass of the new disc is αR from the centre of the bigger disc. The value of a is:
View Solution

