Two identical slabs are welded end to end and 20cal of heat flows through if for 4min If the two slabs are now welded by placing them one through two ends under the same difference of temperatures the time taken is .
1min
2min
4min
16min
The correct Answer is:A
To solve the problem, we need to analyze the heat transfer through the two slabs in two different configurations: first, when they are arranged end to end, and second, when they are arranged in parallel.
1. Understanding the Setup:
- We have two identical slabs, each with thermal conductivity K, length L, and cross-sectional area A.
- When arranged end to end, the total length becomes 2L.
2. Heat Transfer Through End-to-End Arrangement:
- The total thermal resistance R for two slabs in series is given by:
R=R1+R2=LKA+LKA=2LKA
- The heat Q flowing through the slabs is given by:
Q=T1−T2R
- Given that Q=20kcal flows in 4min:
20kcal4min=T1−T22LKA⟹T1−T2=20kcal⋅2LKA⋅4min=10kcal⋅2LKA
3. Heat Transfer Through Parallel Arrangement:
- When the slabs are arranged in parallel, the thermal resistance for each slab is:
R1=R2=LKA
- The total thermal resistance Rthermal for two slabs in parallel is given by:
Rthermal=R1R2R1+R2=(LKA)(LKA)LKA+LKA=L2K2A22LKA=L2KA
- The heat flow Q in parallel arrangement is given by:
Q=T1−T2Rthermal=T1−T2L2KA=2KA(T1−T2)L
4. Relating Heat Flow in Parallel to End-to-End:
- From the previous step, we know T1−T2=10kcal⋅2LKA.
- Substituting this into the equation for Q:
Q=2KA⋅10kcal⋅2LKAL=40kcal
- This heat Q will flow through the parallel arrangement in time t:
40kcalt=T1−T2L2KA⟹t=40kcal⋅L2KAT1−T2
- Substituting T1−T2=10kcal⋅2LKA:
t=40kcal⋅L2KA10kcal⋅2LKA=4010=4min
5. Final Calculation:
- The time taken for 40kcal to flow through the slabs arranged in parallel is:
t=1min
Conclusion:
The time taken for the heat to flow through the two slabs when arranged in parallel is 1 minute.
- We have two identical slabs, each with thermal conductivity K, length L, and cross-sectional area A.
- When arranged end to end, the total length becomes 2L.
- The total thermal resistance R for two slabs in series is given by:
R=R1+R2=LKA+LKA=2LKA
- The heat Q flowing through the slabs is given by:
Q=T1−T2R
- Given that Q=20kcal flows in 4min:
20kcal4min=T1−T22LKA⟹T1−T2=20kcal⋅2LKA⋅4min=10kcal⋅2LKA
- When the slabs are arranged in parallel, the thermal resistance for each slab is:
R1=R2=LKA
- The total thermal resistance Rthermal for two slabs in parallel is given by:
Rthermal=R1R2R1+R2=(LKA)(LKA)LKA+LKA=L2K2A22LKA=L2KA
- The heat flow Q in parallel arrangement is given by:
Q=T1−T2Rthermal=T1−T2L2KA=2KA(T1−T2)L
- From the previous step, we know T1−T2=10kcal⋅2LKA.
- Substituting this into the equation for Q:
Q=2KA⋅10kcal⋅2LKAL=40kcal
- This heat Q will flow through the parallel arrangement in time t:
40kcalt=T1−T2L2KA⟹t=40kcal⋅L2KAT1−T2
- Substituting T1−T2=10kcal⋅2LKA:
t=40kcal⋅L2KA10kcal⋅2LKA=4010=4min
- The time taken for 40kcal to flow through the slabs arranged in parallel is:
t=1min
The time taken for the heat to flow through the two slabs when arranged in parallel is 1 minute.
Topper's Solved these Questions
- BOOK - NARAYNACHAPTER - TRANSMISSION OF HEATEXERCISE - LEVEL-III (C.W)1videos
TRANSMISSION OF HEAT
- BOOK - NARAYNACHAPTER - TRANSMISSION OF HEATEXERCISE - LEVEL- (C.W)25videos
TRANSMISSION OF HEAT
- BOOK - NARAYNACHAPTER - TRANSMISSION OF HEATEXERCISE - LEVEL - 2 (C.W)1videos
TRANSMISSION OF HEAT
- BOOK - NARAYNACHAPTER - THERMODYNAMICSEXERCISE - Exercise187videos
THERMODYNAMICS
- BOOK - NARAYNACHAPTER - UNITS AND MEASUREMENTSEXERCISE - STATEMENT TYPE QUESTION23videos
UNITS AND MEASUREMENTS
Similar Questions
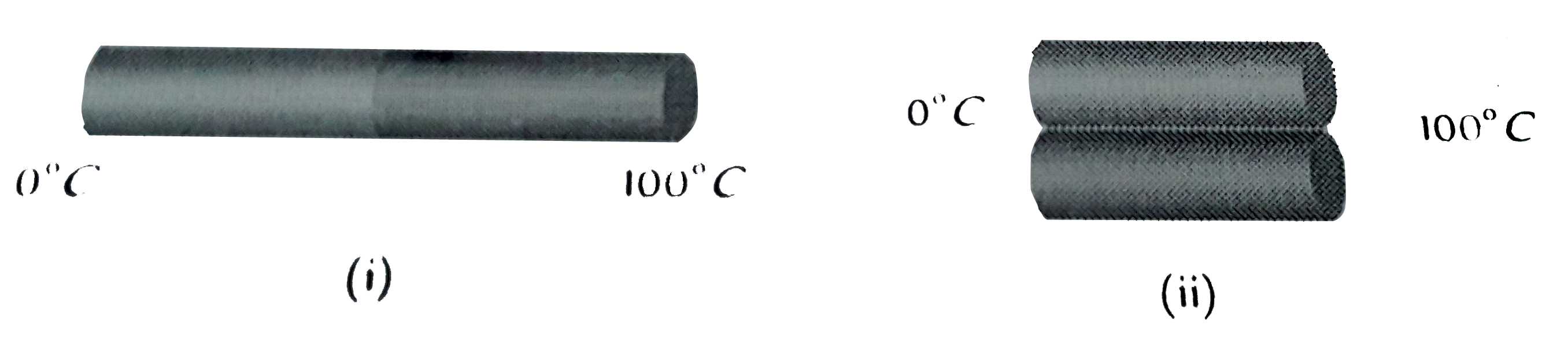
Two identical metal plates are welded end to end as shown in - (i) . 20 cal of heat flows through it in 4 minutes. If the plates are welded as shown in figure-(ii) , find the time (in minutes) taken by the same amount of heat to flow through the plates.
_S01_132_Q01.png) View Solution
View SolutionTwo identical square rods of metal are welded end to end as shown in figure (i), 20 calories of heat flows through it in 4 minutes. If the rods are welded as shown in figure (ii), the same amount of heat will flow through the rods in
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
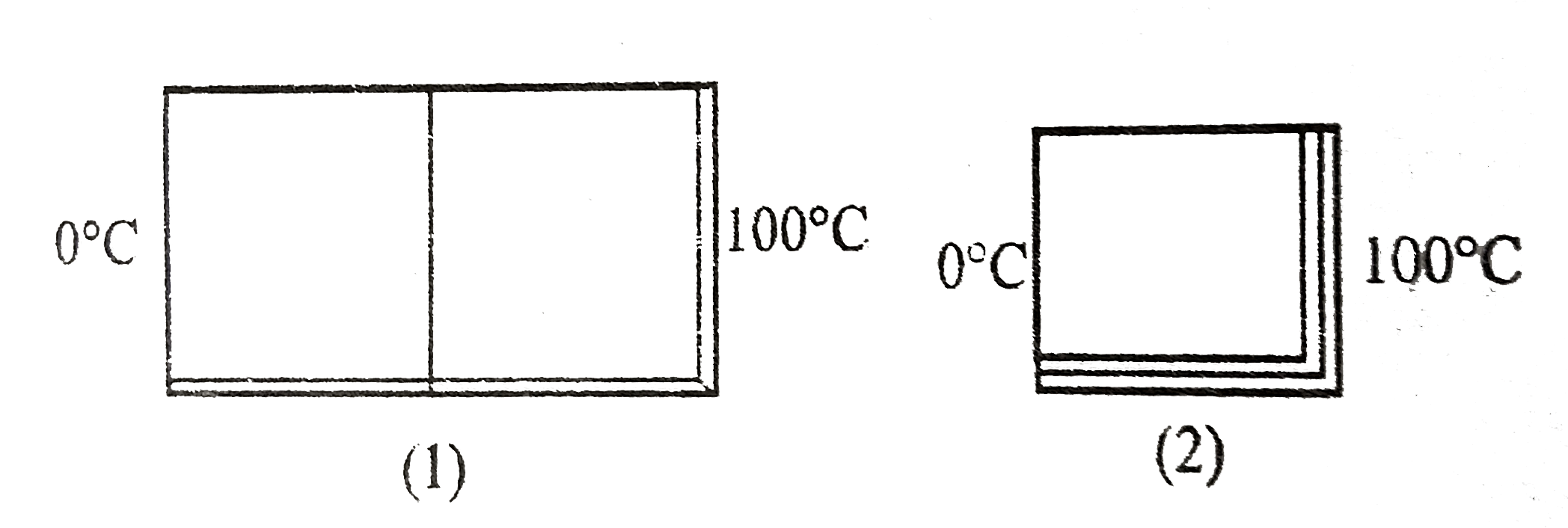
Two identical square rods of metal are welded end to end as shown in fig (1). 20cal of heat flows through it in 4min. If the rods are welded as shown in fig.(2), the same amount of heat will flow through the rods in
 A1minB2minC4minD16min
A1minB2minC4minD16min - Question 2 - Select One
Two identical square rods of metal are welded end to end as shown in figure (i), 20 calories of heat flows through it in 4 minutes. If the rods are welded as shown in figure (ii), the same amount of heat will flow through the rods in
 A1 minuteB2 minutesC4 minutesD16 minutes
A1 minuteB2 minutesC4 minutesD16 minutes - Question 3 - Select One
Two identical rods of a metal are welded as shown in figure-4.39(a). 20 cal of heat flows through them in 4 minute. If the rods are welded as shown in figure-4.39(b), then the same amount of heat will flow in:
 A1 minuteB2 minuteC4 minuteD16 minute
A1 minuteB2 minuteC4 minuteD16 minute
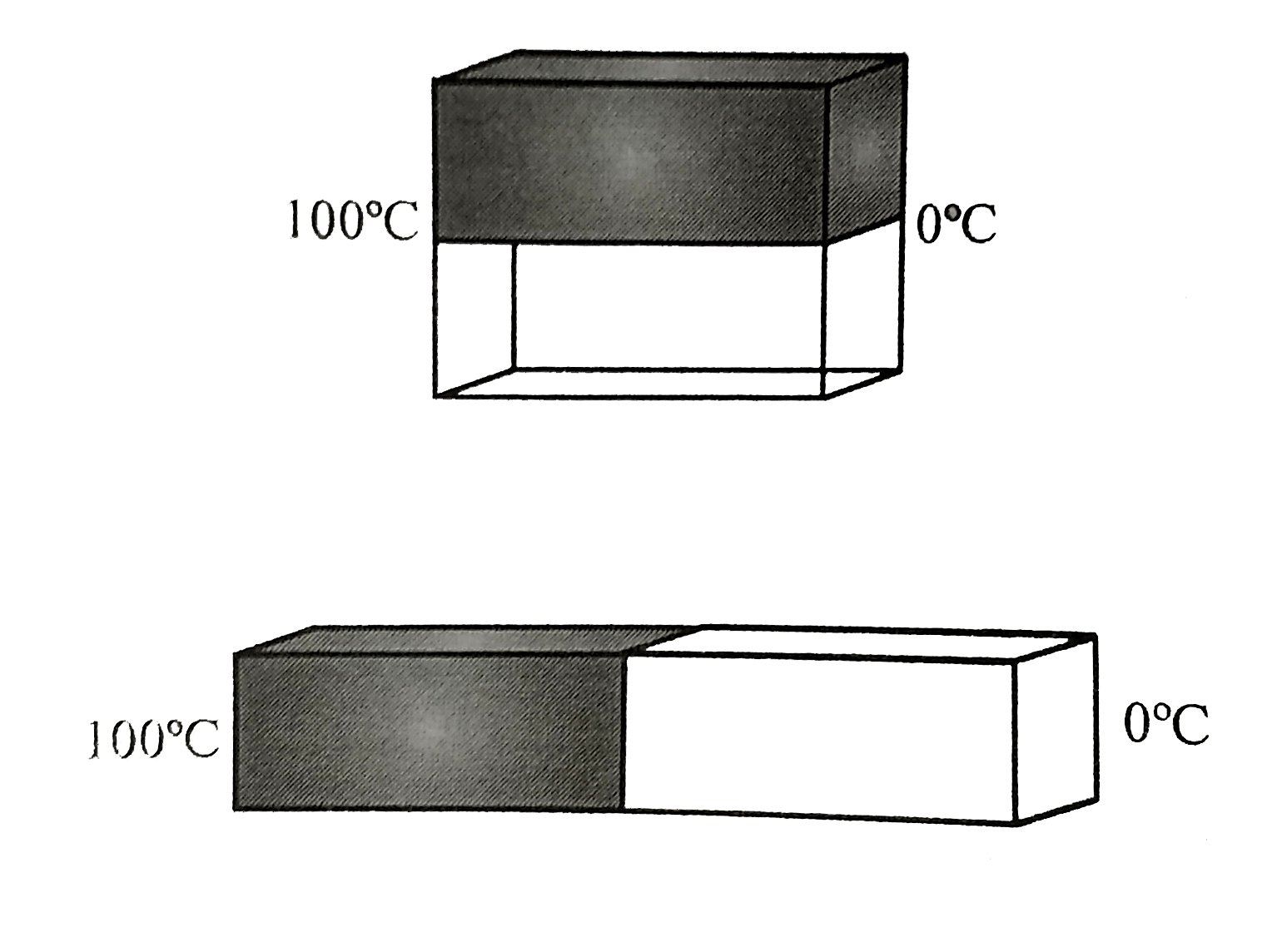
Two identical rectangular rods of metal are welded as shown in figure (1) and 20J of heat flows through the rods in 1min. How long would it take for 20J heat to flow through the rods if they are welded as shown in figure (2)
 View Solution
View SolutionTwo identical rectangular rods of metal are welded end to end in series between temperature 0oC and 100∘C and 10J of heat is conducted (in steady state process) through the rod in 2.00min. If 5 such rods are taken and joined as shown in the figure maintaining the same temperature difference between A and B, then the time in min in which 20J of heat will flow through the rods is
'(##CEN_KSR_PHY_JEE_C13_E01_014_Q03##)'View SolutionTwo identical square rods of metal are welded end to end as shown in figure (a). Assume that 10 cal of heat flows through thr rod in 2min. Now the rods are welded as shown in figure (b). The time it would take for 10 cal to flow through the rods now, is
 View Solution
View SolutionTwo ends of a conductor are at different temperatures the electromotive force generated between two ends is
View SolutionTwo rods are of same material and having same length and area. If heat ΔQ flows through them for 12 min when they are joined side by side. If now both the rods are joined in parallel, then the same amount of heat ΔQ will flow in
View Solution

