The gap between the plates of a parallel plate capacitor is filled with glass of dielectric constant and of specific resistivity . The capacitance of the capacitor is . When a voltage of is applied to the capacitor, the leakage current of the capacitor will be.
Zero
The correct Answer is:C
Let be the initial charge on the capacitor and its initial potential. Let be the area of the plates and be the distance between them.
Capacitance of the capacitor is
Resistance of the capacitor is ltbegt If be the charge at time , then the leakage current is where is the volt age acros the capacitor at time . But
Hence
Hence . The minus sign is because is negative.
Integrating between limits,
Hence
This gives the leakage current at instant . Initial leakage current is obtained at
Hence initial leakage current.
.
Topper's Solved these Questions
- BOOK - NARAYNACHAPTER - CAPACITANCEEXERCISE - Level-VI28videos
CAPACITANCE
- BOOK - NARAYNACHAPTER - CAPACITANCEEXERCISE - Subjective9videos
CAPACITANCE
- BOOK - NARAYNACHAPTER - CAPACITANCEEXERCISE - NCERT Based13videos
CAPACITANCE
- BOOK - NARAYNACHAPTER - ATOMS EXERCISE - EXERCISE -447videos
ATOMS
- BOOK - NARAYNACHAPTER - COMMUNICATION SYSTEMEXERCISE - Level-II(H.W)25videos
COMMUNICATION SYSTEM
Similar Questions
The gap between the plates of a parallel-plate capacitor is filled with glass of resistivity ρ=100GΩm, The capacitance of the capacitors equals C=4.0nF. Find the leakeage current of the capacitor when a voltage V=2.0kV is applied to it.
View SolutionDerive an expression for the capacitance of a parallel-plate capacitor filled with a dielectric.
View Solution
Knowledge Check
- Question 1 - Select One
If the gap between the plates of a parallel plate capacitor is filled with medium of dielectric constant k=2 , then the field between them
AIncreases by a factor 2BIncreases by a factor √2CDecreased by a factor √2DDecreased by a factor 1/2 - Question 2 - Select One
A parallel plate capacitor is filled with dielectrics. What is capacitance?
 A2K1K2K1+K2BK1K2K1+K2C2ε0Ad(K1K2K1+K2)D2ε0Ad(2K1+K22K1K2)
A2K1K2K1+K2BK1K2K1+K2C2ε0Ad(K1K2K1+K2)D2ε0Ad(2K1+K22K1K2) - Question 3 - Select One
The gap between the plates of a parallel plate capacitor is filled with glass of resistivity ρ. The capacitance of the capacitor without glass equals C. The leakage current of the capacitor when a voltage V is applied to it is
AVρCε0BCVρε0CVε0CρDCVρε0
A parallel plate capacitor is filled with dielectrics as shown in Fig. What is its capacitance ?
 View Solution
View SolutionThe area A of a parallel plate capacitor is divided into two equal, halves and filled with two media of dielectric constants K1andK2 respectively. The capacitance of the capacitor will be
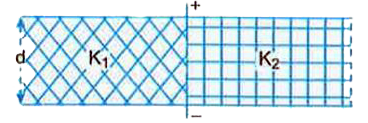 View Solution
View SolutionThe voltage between the plates of a parallel plate capacitance is changing at the rate of 10 V/s . Find the displacement current in the capacitor if the capacitance of the capacitor is 2.0 μF.
View SolutionA parallel plate capacitor with air between plates (dielectric constant K =2) has a capacitance C. If the air is removed, then capacitance of the capacitor is
View SolutionIn figure half of the space between the plates of a parallel plate capacitor is filled with dielectric material of constant K. Then which of the plots are possible?
 View Solution
View Solution

